Question

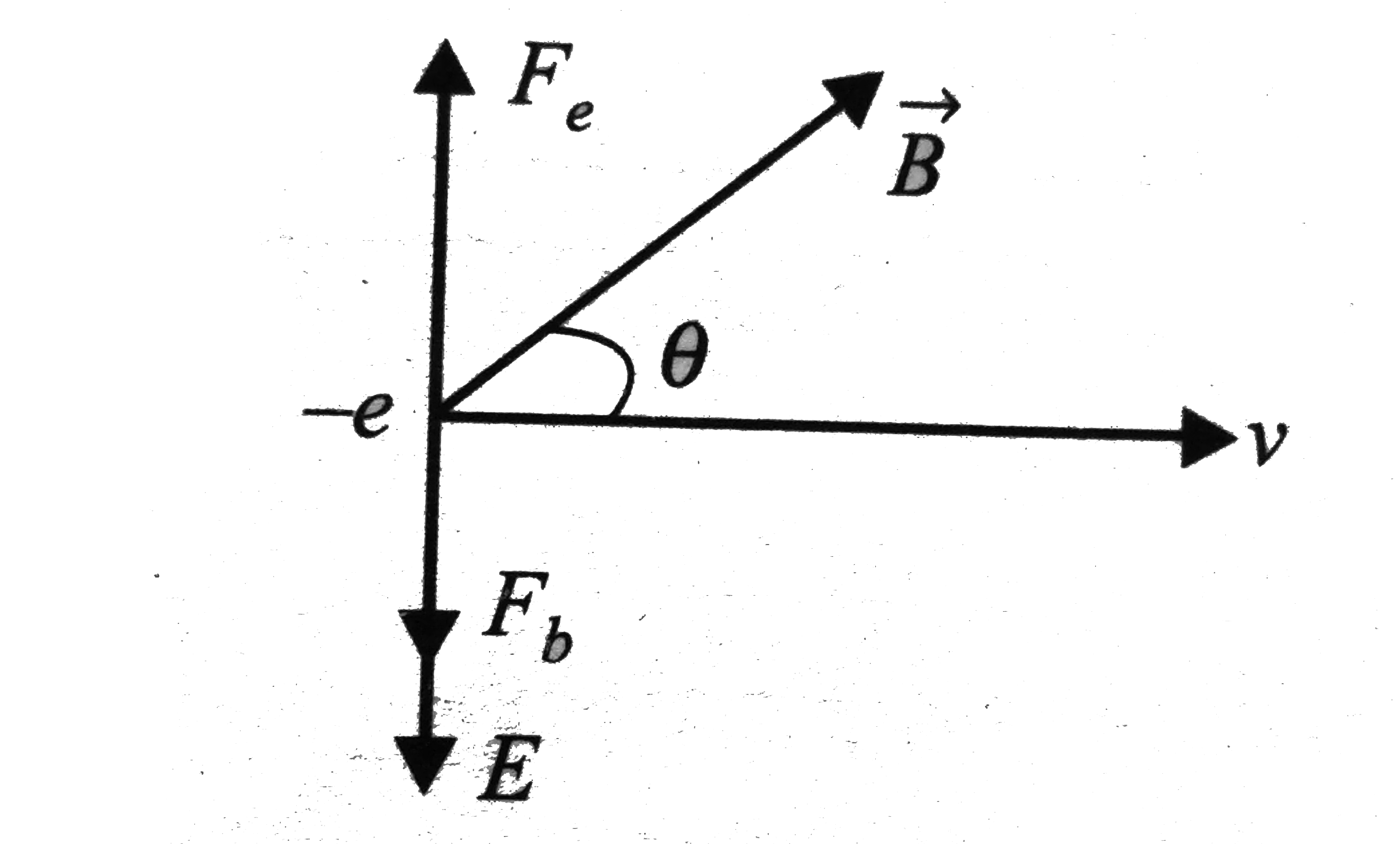
An electron accelerated by a potential difference 2.5 kV moves horizontally into a region of space in which there is a downward directed uniform electric field of magnitude $10kV{m}^{-1}.$
(a) In what direction must a magnetic field be applied so that the electron moves undeflected? Ignore the gravitational force. What is the magnitude of the smallest magnetic field possible in this case?
(b) What happens if the charge is a proton that passes through the same combination of fields ?

Explanation

Velocity of electron: `1/2mv^2=2.5xx10^-3xx1.6xx10^-19`
`implies v=sqrt((2xx2.5xx10^3xx1.6xx10^-19)/(9.1xx10^-31))=2.96xx10^7 ms^-1`
(a) For the electron to move undeflected: (magnetic field should be perpendicular to E, but it can be at any angle with `vecv`)
`F_b = F_e implies B=E/(sin theta)`
for smallest B, `sin theta` is maximum i.e. `theta=90^@`
`implies B_(min) =E/v=(10xx10^3)/(2.96xx10^7)=3.38xx10^-4 T`

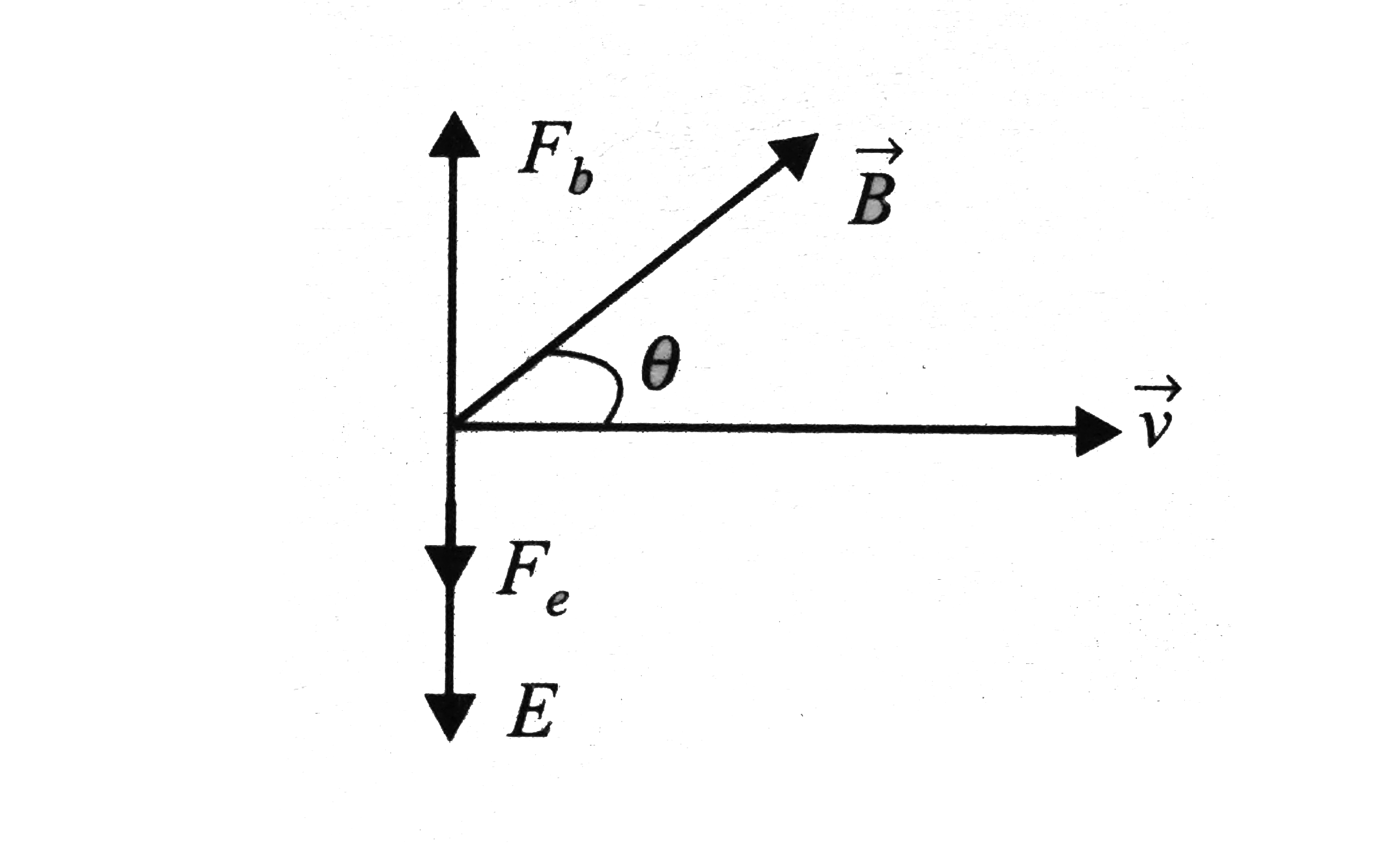
(b) B or `B_(min)` is independent of magnitude and sign of charge if the particle moves undeflected. So, in this case also
`B_(min)=3.38xx10^-4T`
But we see that direction of both `F_e` and `F_b` is opposite to that in part
(a) `B_(min)=E/v=(10xx10^3)/(2.96xx10^7)=3.38xx10^-4T`
.