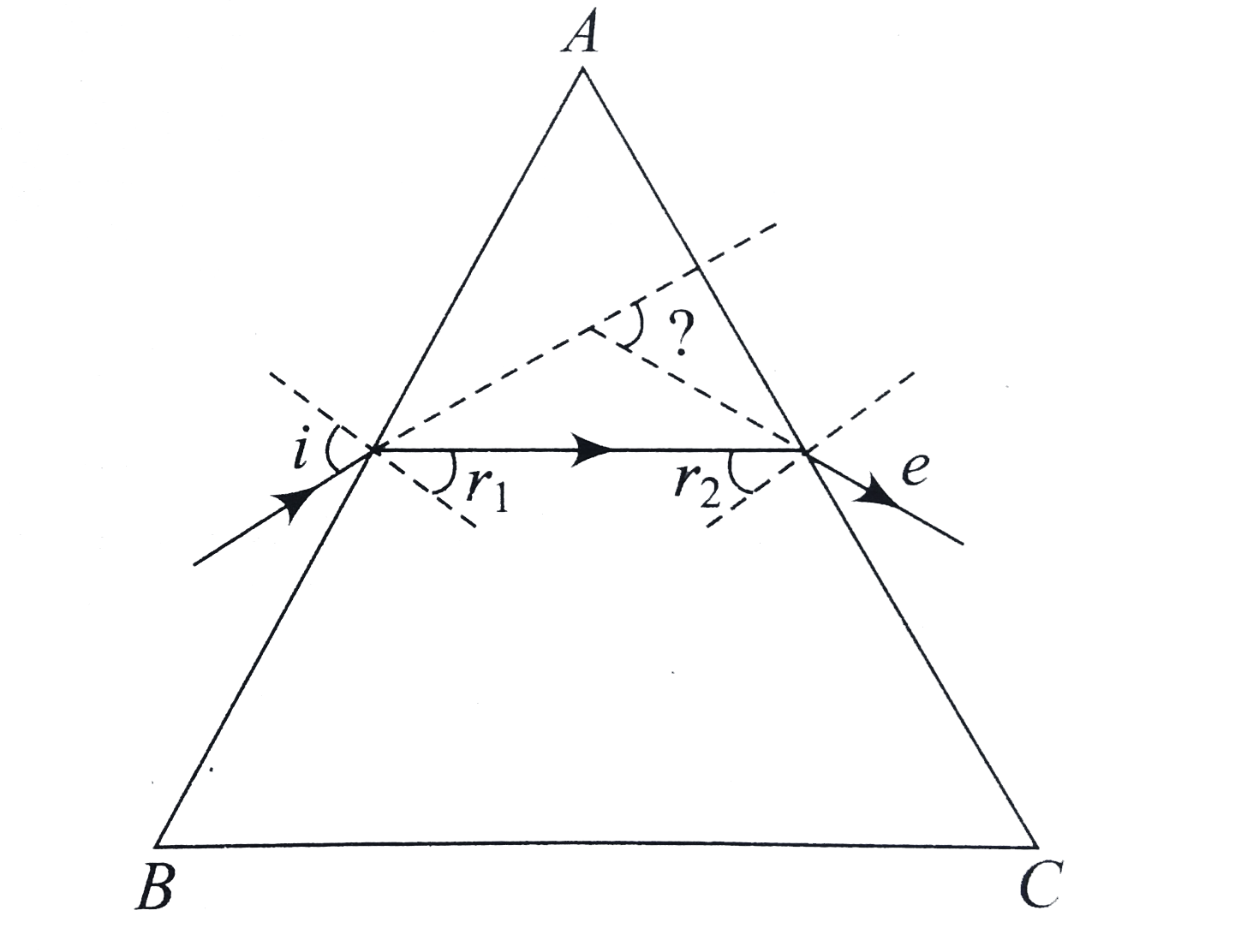
A ray of light is incident at an angle of 60∘ on one face of a prism which has refracting angle of 30∘ . The ray emerging out of the prism makes an angle of 30∘ with the incident ray. If the refractive index of the material of the prism is μ=√a , find the value of a .
The correct Answer is:3
Given i+60∘,δ=30∘andA=30∘.
We have δ=i+e−A
Form Eq. (i), we get
30∘=60∘+e−30∘ore=0
So r2 is also zero, then r1=A=30∘
So μ=sinisinr1=sin60∘sin30∘=√3
Hence the value of a=3.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Archives
7 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - True/False
5 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Linked Comprehension
51 Videos
- FRICTIONBOOK - CENGAGE PHYSICSCHAPTER - FRICTIONEXERCISE - QUESTION BANK
1 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - Question Bank
39 Videos
Similar Questions
A ray of light is incident at an angle of 60∘ on a prism whose refracting angle is 30∘ . The ray emerging out of the prism when produced backward makes an angle of 30∘ with the incident ray produced forward. Find the refractive index of the material of the prism.
View SolutionA ray of light is incident at an angle of 60∘ on the face of a prism having refracting angle 30∘. The ray emerging out of the prism makes an angle 30∘ with the incident ray. Show that the emergent ray is perpendicular to the face through which it emerges.
View Solution
Knowledge Check
A ray of light is incident at an angle of 60∘ on one face of a prism of angle 30∘. The ray emerging out of the prism makes an angle of 30∘ with the incident ray. The emergent ray is
ANormal to the face through which it emergesBInclined at 30∘ to the face through which it emergesCInclined at 60∘ to the face through which it emergesDNone of theseA ray of light is incident at an angle of 60∘ on one face of a 30∘ prism . The emergent ray from the prism makes an angle of 30∘ with the incident ray. The angle of emergence and refractive index of the material of the prism are-
A90∘,√3B0∘,√3C0∘,√2D90∘,√2A ray of light is incident at an angle of 60∘ on one face of a prism of angle 30∘. The ray emerging out of the prism makes an angle of 30∘ with the incident ray. The emergent ray is
A0∘B90∘C30∘D45∘
A ray of light is incident at an angle of 60∘ on the face of a prism having refracting angle 30∘. The ray emerging out of the prism makes an angle 30∘ with the incident ray. Show that the emergent ray is perpendicular to the face through which it emerges and calculate the refractive index of the material of prism.
View SolutionA ray of light is incident at an angle of 60∘ on one face of a prism of angle 30∘. They ray emerges normally from the other face of the prism. The refractive index of the prism material is
View SolutionWhen light rays are incident on a prism at an angle of 45∘, the minimum deviation is obtained. If refractive index of the material of prism is √2, then the angle of prism will be
View SolutionA monochromatic ray of light is incident normally on one of the refracting surfaces of a prism . The emergent ray grazes the second refracting surface . What is the angle of the prism if the refractive index of the material of the prism is 1.6 ?
View SolutionA ray of light is incident at an angle of 60∘ on the face of a prism with an angle of 60∘. Then the refractive index of the material of the prism is (the prism is in minimum deviation position)
View Solution
