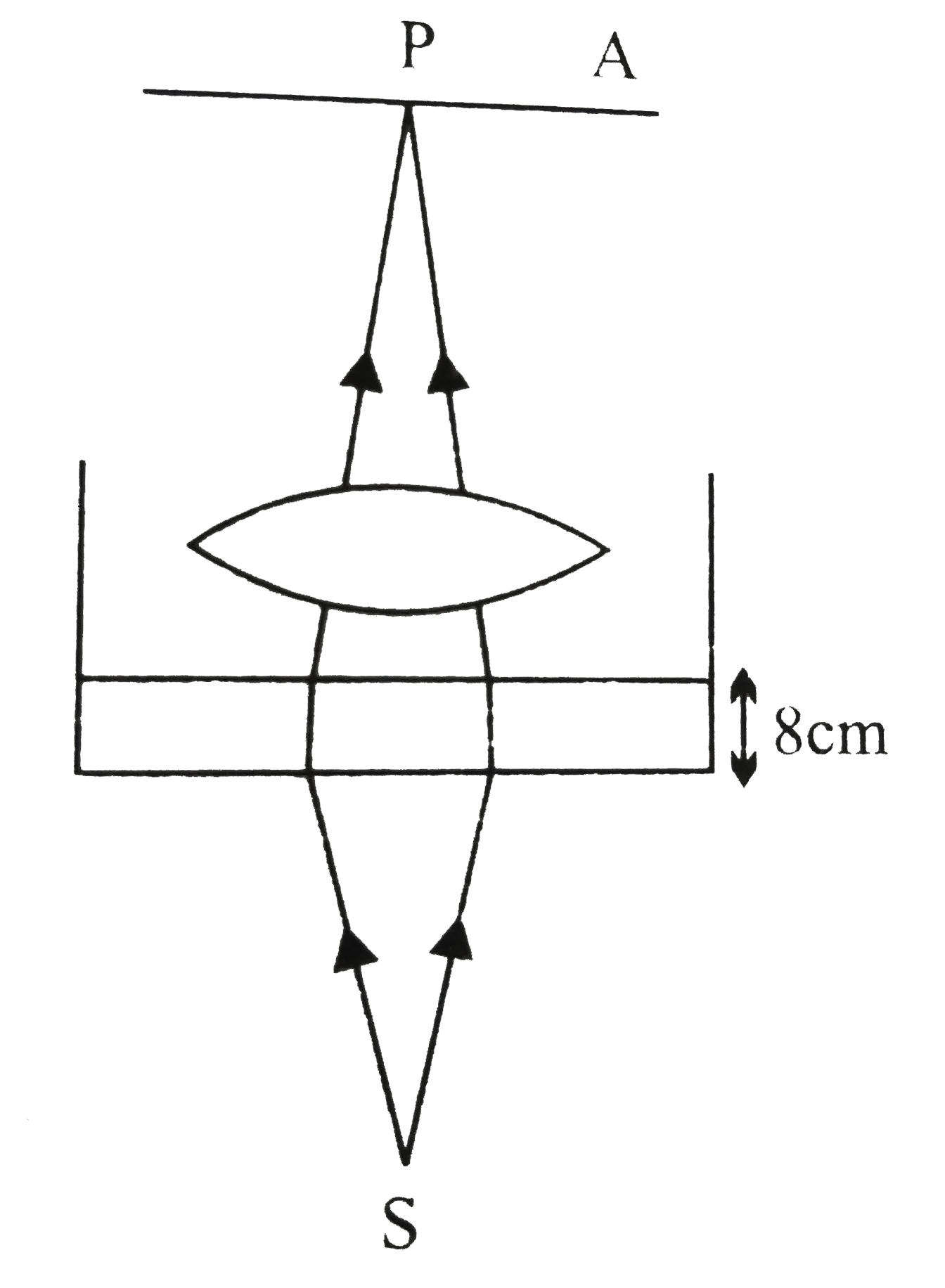
A thin convex lens of refractive index μ=1.5 is placed between a point source of Light S and a screen A, shown in figure,. Light rays from the source S are brought to focus on the screen A, forming a point image P. The distance SP is equal to 50cm. Water (μ=4/3) is now poured into a vessel interposed between the object and the lens, and it is observed that when the water level is 8cm the screen has to be moved up by a distance of 6cm in order to get a sharp image. Find the focal length of the lens.
The correct Answer is:|u|=20cm
|v|=30cm and f=12cm
Suppose that the object distance (between the oject and the lens) be u and the image distance v. Then,
|u|+|v|=50
1|v|−1|u|=1f (i)
When water is poured into the vessel,
1(|v|+6)−1−(|u|−2)=1f (ii)
Solving the equation, we get
|u|=20cm
|v|=30cm and f=12cm
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Single Correct
221 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Assertion-Reasoninig
2 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.7
21 Videos
- FRICTIONBOOK - CENGAGE PHYSICSCHAPTER - FRICTIONEXERCISE - QUESTION BANK
1 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - Question Bank
39 Videos
Similar Questions
A screen is placed 80 cm from an object. The image of the object on the screen is formed by a convex lens placed between them at two different locations separated by a distance 20 cm. Determine the focal length of the lens.
View SolutionAn object 50 cm tall is placed on the principle axis of a convex lens. Its 20 cm tall image is formed on the screen placed at a distance of 10 cm from the lens. Calculate the focal length of the lens.
View Solution
Knowledge Check
If the distance between a point source and screen is doubled, then intensity of light on the screen will become
AFour timesBDoubleCHalfDOne-fourthA convex lens forms a real image on a screen placed at a distance 60 cm from the object. When the lens is shifted towards the screen by 20 cm , another image of the object is formed on the screen. The focal length of the lens is :
A45cmB40/3cmC30cmD12cmA screen is placed a distance 40 cm away from an illuminated object. A converging lens is palced between the source and the screen and it is attempted to form the image of the source on the screen. If no position could be found, the focal length of the lens
Amust be less than 10 cmBmust be greater than 20 cmCmust not be greater than 20 cmDmust not be less then 10 cm
An object 50cm tall is placed on the principal axis of a convex lens. Its 20cm tall image is formed on the screen placed at a distance of 10cm from the lens. Calculate the focal length of the lens.
View SolutionThe distance between an object and a convex lens is changing. It is noticed that the size of the image formed on a screen is decreasing. Is the object moving in a direction towards the lens or away from it?
View SolutionAn object is placed 30cm away from a convex lens of focal length 10cm and a sharp image is formed on a screen. Now, a convex lens is placed In contact with the convex lens. The screen now has to be moved by 45cm to get a sharp image again. The magnitude of focal length of the convex lends in (in cm)
View SolutionA bi-convex lens is placed between a light source and a concave mirror as shwon such that image of the light source coincides with itself. Then,
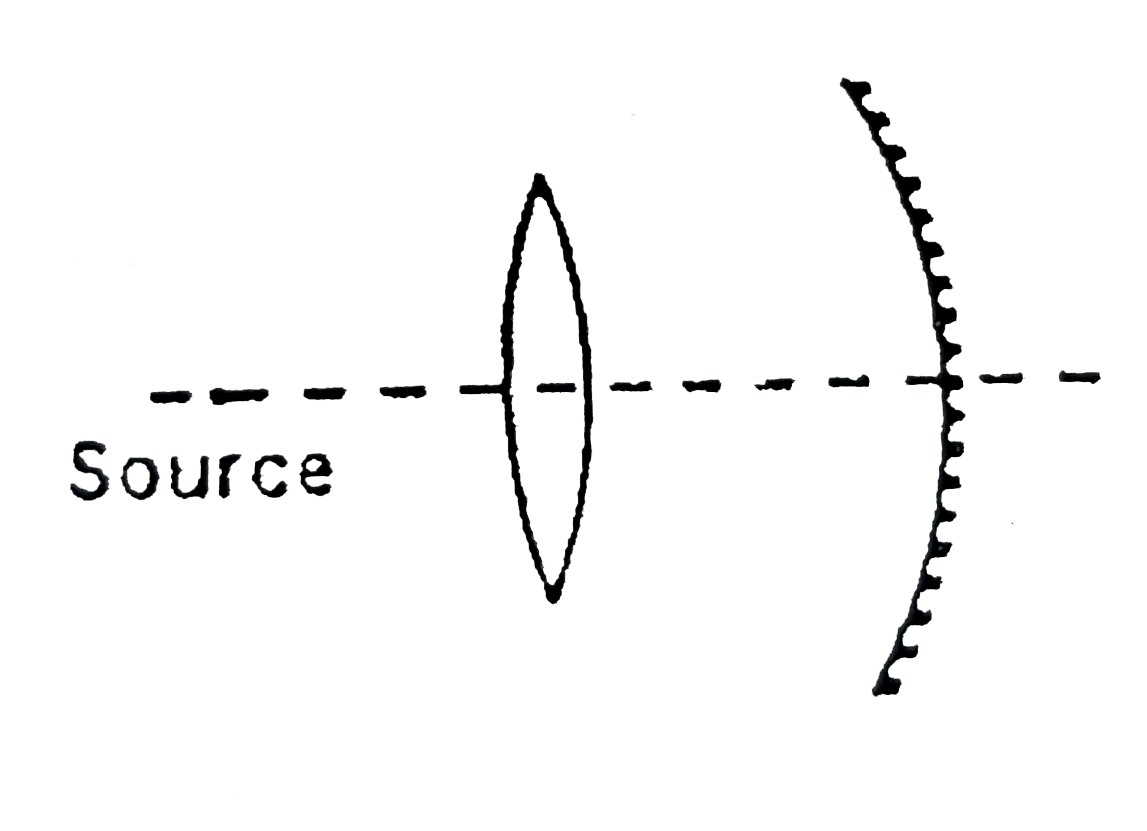
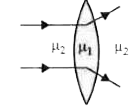
View SolutionA convex lens made up of material of refractive index μ1, is immersed in a medium of refractive index μ2 as shown in the figure. The relation between μ1 and μ2 is
View Solution
