Consider a coaxial system of two thin convex lenses of focal length f each separated by a distance d. Draw ray diagrams for image formation corresponding to an object at infinity placed on the principal axis in the following cases: (a) $d < f$ ,(b) $d = f$ , (c ) $f < d < 2 f$ , (d) $d = 2 f$ , and (e) $d > 2 f$ . Indicate the nature of the combination (concave, convex or plane) in each case.

Video Solution

Text Solution

Verified by Experts

In each of two thin lenses separated by a distance d, we have
$\frac{1}{F} = \frac{1}{f_{1}} + \frac{1}{f_{2}} - \frac{d}{f_{1} f_{2}}$
For $f_{1} = f, f_{2} = f$ ,
$\frac{1}{F} = \frac{1}{f} + \frac{1}{f} - \frac{d}{f^{2}}$
$F = \frac{f^{2}}{2 f - d}$ (i)
So, if $d < f$ : F will be positive and $> f$ . So, the system will behave as a convex lens of focal length $< f$ as shown in Figure.
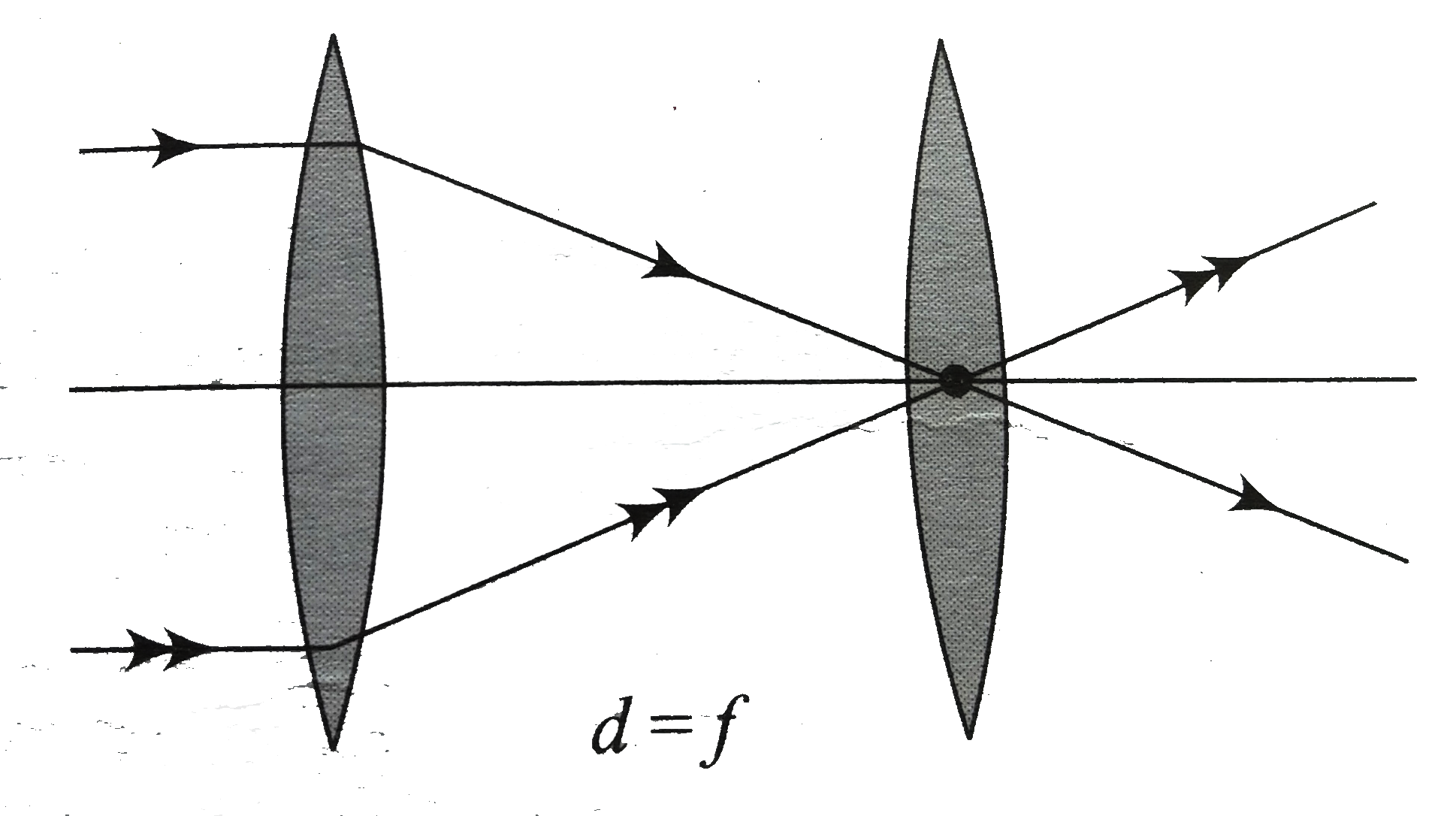
b. If $d = f : F = f$ i.e., the system will behave as a convex lens of focal length f as shown in Figure
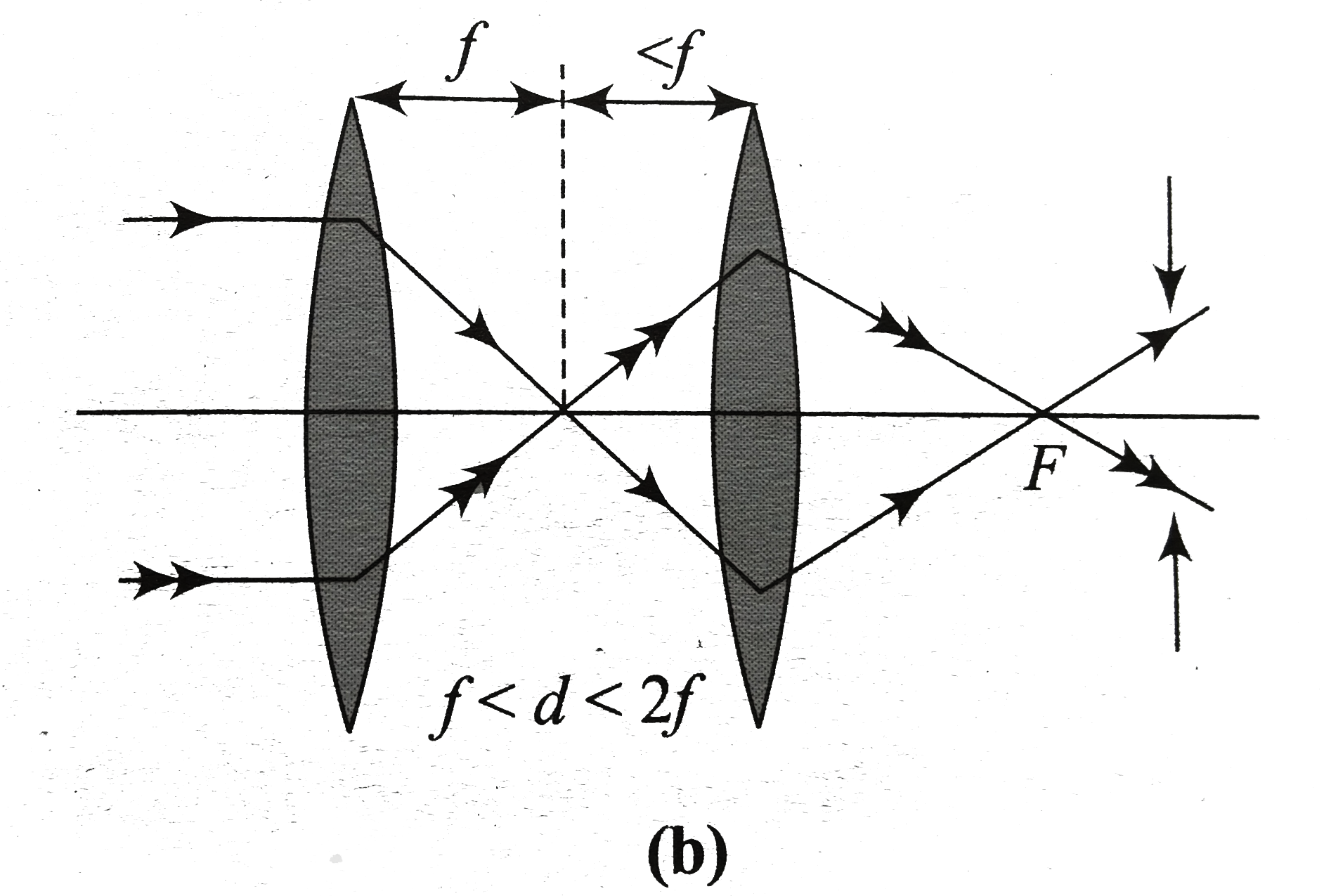
c. If $f < d < 2 f$ : F will be positive and $< f$ , so the system will behave as a convex lens of focal length $> f$ as shown in Figure.
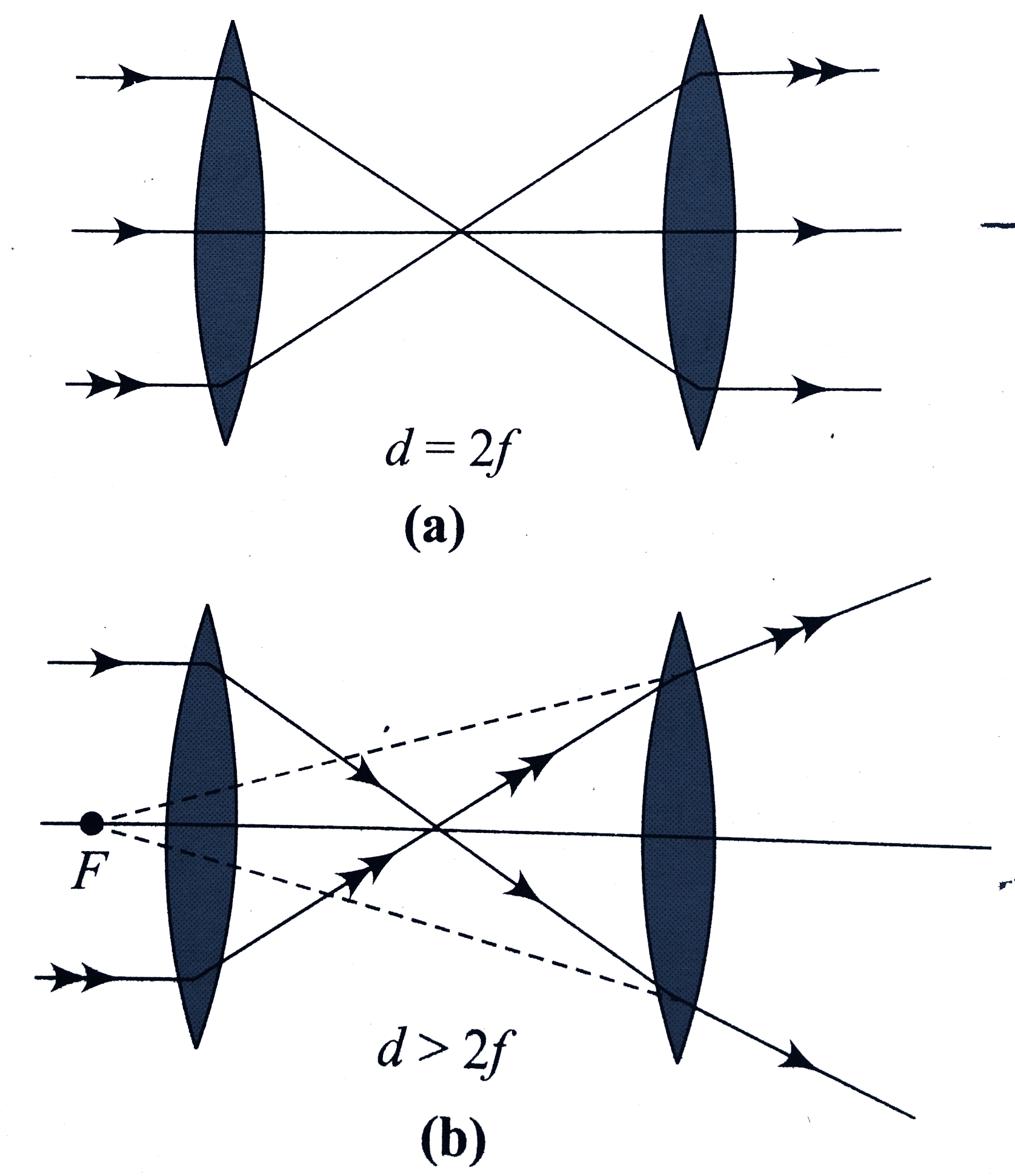
d. If $d = 2 f$ : F will be indefinit, i.e., the system iwll behave as a plane glass plate of infinite focal length
If $d > 2 f$ : F will become negative, i.e., the system will behave as concave lens as shown in Figure.

Updated on:21/07/2023

Two convex lenses of focal lengths $f_{1} and f_{2}$ are separated co-axially by a distance d. The power of the combination will be one if
A $d = (f_{1} + f_{2}) - f_{1} f_{2}$
B $d = (f_{1} - f_{2}) + f_{1} f_{2}$
C $d = \sqrt{f_{1} f_{2}} . . f_{1} + f_{2}$
D $d = (\frac{f_{1} - f_{2}}{2})$
Two thin convex lenses of focal lengths $f_{1}$ and $f_{2}$ are in contact and coaxial. The power of the combination is
A $\sqrt{\frac{f_{1}}{f_{2}}}$
B $\sqrt{\frac{f_{2}}{f_{1}}}$
C $\frac{f_{1} + f_{2}}{2}$
D $\frac{f_{1} + f_{2}}{f_{1} f_{2}}$
Two convex lenses of focal length $f_{1}$ and $f_{2}$ are mounted coaxially separated by a distance. If the power of the combination is zero, the distance between the lenses is
A $| f_{1} - f_{2} |$
B $f_{1} + f_{2}$
C $\frac{f_{1} f_{2}}{| f_{1} - f_{2} |}$
D $\frac{f_{1} f_{2}}{f_{1} - f_{2}}$