Figure.. Shows a triangular prism of refracting angle 90∘ . A ray of light incident at face AB at an angle θ1, refracts at point Q with an angle of refraction 90∘.
a. What is the regractive index of the prism in terms of θ1?
b. What is the maximum value that the refractive inde can have?
c. What happens to the light at Q if the incident angle at Q is (i) increased slightly, and (ii) decrease slightly?
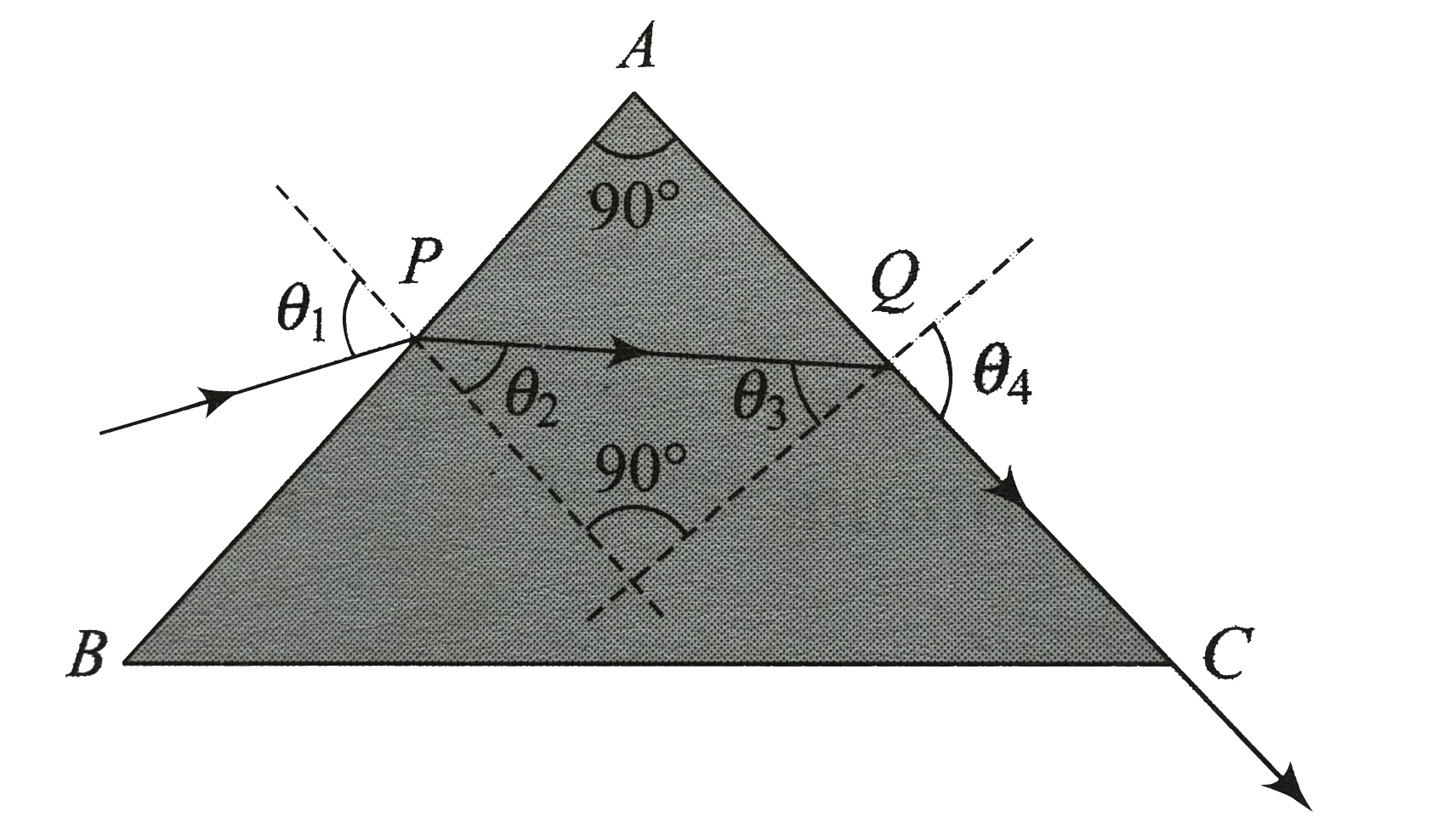
a. Let the ray be incident at an angle θ1 at the face AB. It refracts at an angle θ2 and is incident at an angle θ3 at the face AC. Finally, the ray comes out at an angle θ4=90∘.
From Fig. , the normal at faces AB and AB make an angle of 90∘ width each other, θ3=90∘−θ2
sinθ3=sin(90∘−θ2)=cosθ2=√1−sin2θ2 (i)
From Snell's law at face AC, nsinθ2=1
√1−sin2θ2=1 (ii)
Form Snell's law at face AB, 1sinθ1=nsinθ2
sinθ2=singθ1n (iii)
From Eqs. (ii) and (iii), we have
n√1−sin2θ1n2=1 (iv)
On squaring Eq. (iv) and solving for n, we get
n=√1+(sin2θ1)
b. The greatest possible value of sin2θ1 is 1, hence, the greates possible value of n is nmax=√2=1.41.
c. i. For a given n, if θ1 is increased the angle of refraction θ2 increases. As θ3=90∘−θ2 the angle θ decreases, i.e., the angle of incidence at face AC is less than the critical angle for total reflection, hence light light emerges into air.
ii. If the angle of incidence is decresed, the angle of refraction θ2 decreases. So, the angle θ3 increases. The angle of incidence at the second surface is greater than the critical angle, so light is reflected at Q.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBook:CENGAGE PHYSICSChapter:GEOMETRICAL OPTICSExercise:Solved Examples
Explore 15 Videos
- GEOMETRICAL OPTICSBook:CENGAGE PHYSICSChapter:GEOMETRICAL OPTICSExercise:Exercise1.1
Explore 14 Videos
- FRICTIONBook:CENGAGE PHYSICSChapter:FRICTIONExercise:QUESTION BANK
Explore 1 Video
- GRAVITATIONBook:CENGAGE PHYSICSChapter:GRAVITATIONExercise:Question Bank
Explore 39 Videos
Similar Questions
The face AC of a prism ABC of refracting angle 30∘ is silvered . A ray is incident on face AB at an angle of 45∘ as shown in figure . The refracted ray undergoes reflection at face AC and retraces its path . The refractive index of the prism is

View SolutionAt what angle should a ray of light be incident on the face of a prism of refracting angle 60∘ so that it just suffers total internal reflection at the other face ? The refractive index of the prism is 1.524.
View Solution
Knowledge Check
The refractive index of a prism is 2. this prism can have a maximum refracting angle of
A90∘B60∘C45∘D30∘A ray of light is incident at an angle of 60∘ on the face of a prism with an angle of 60∘. Then the refractive index of the material of the prism is (the prism is in minimum deviation position)
A1.414B1.623C1.524D1.732The refractive index of a prism is 2. The prism can have a maximum refracting angle of
A90∘B60∘C45∘D30∘
A ray of light is incident on a glass slab at an angle of 45∘. If refractive index of glass be 1.6, what is the angle of refraction ?
View SolutionA ray of light is incident at 60∘ on one face of a prism of angle 30∘ and the emergent ray makes 30∘ with the incident ray. The refractive index of the prism is
View SolutionA monochromatic ray of light strikes one face of a prism at an angle of 60∘ and the angle of refraction is 30∘ . The refracting angle of the prism is 60∘ . What is the angle of emergence ?
View SolutionA ray of light is incident at an angle of incidence 45∘ on an equilateral prism and emerge at an angle 45∘ then the refractive index of the medium of the prism is:
View SolutionA ray of light is incident at 65∘ on one face of a prism of angle of 30∘ and the emergent ray makes 35∘ with the incident ray. The refractive index of the prism is:
View Solution