A vessel having perfectly reflecting plane botton is filled with water (μ=4/3) to depth d. A point source of light is placed at a height h above the surface of water. Find the distance of final image from water surface.
As shown in Fig. water will form the image of object O at I1 such that OI1=y=d[1−(1/μ)], so that the distance of image I1 from water surface will be I1A=h−y=h−d[1−(1/μ)]. Hence, the distance of this image I1 from miror MM',
I1M=I1A+AM
=[h−d(1−1−μ)]+d=h+[dμ]
Now, image I1 will act as object for mirror MM' . As a plane mirror forms image at same distance behind the mirror as the object is in fron of is, the image of I1 formed by the mirror MM′ will be I2 such thatI1M=I2M=h+(d/μ) .
Now, this image I2 will act as object for water again and water will produce image I3 such that I2I3=y=d(1−1μ) . So, the distance of image I3 from the surface of water AC will be
AI3=AM+MI2=I2I3=d+[h+dμ]−d[1−1μ]
AI3=h+2dμ=h+32d

Alternative solution:
As shown in Fig. water will form the image of bottom, i.e., mirror MM' at a depth (d/μ) from its surface. So, the distance of object O from virtual mirror mm' will be h+(d/μ)

Now, as a plane mirror froms image behind of it, the distance of image I from mm' be h+(d/μ). Also, as the distance of virtual mirror from the surface of water is (d/μ), the distance of image I from the surface of water will be
[h+dμ]+dμ=h+2dμ=h+32d [as μ=43]
Topper's Solved these Questions
- GEOMETRICAL OPTICSBook:CENGAGE PHYSICSChapter:GEOMETRICAL OPTICSExercise:Solved Examples
Explore 15 Videos
- GEOMETRICAL OPTICSBook:CENGAGE PHYSICSChapter:GEOMETRICAL OPTICSExercise:Exercise1.1
Explore 14 Videos
- FRICTIONBook:CENGAGE PHYSICSChapter:FRICTIONExercise:QUESTION BANK
Explore 1 Video
- GRAVITATIONBook:CENGAGE PHYSICSChapter:GRAVITATIONExercise:Question Bank
Explore 39 Videos
Similar Questions
A point source of light is placed on the bed of a pond 80 cm deep. Find the diameter of the liuminatded circle on the surface of water.
View SolutionA point source is placed at a depth h below the surface of water (refractive index =μ). The medium above the surface of water is air from water.
View Solution
Knowledge Check
A bird is flying 3m above the surface of water. To a fish underwater, the height of the bird from the water surface appears to be
A2.25mB3.33mC4mD4/3mA cylinderical vessel is filled with water up to height H. A hole is bored in the wall at a depth h from the free surface of water. For maximum range h is equal to
AH/4BH/2C3H/4DHA point source of light is placed at a depth h=0.5 m below the surface of a liquid (μ=54) , Then , the fraction of light energy that escape directly from the liquid surface is
A0.1B0.2C0.3D0.4
A point source of light is placed at distance h below the surface of a large and deep lake. What fraction of light will escape through the surface of water?
View SolutionA vessel, whose bottom is flat and perfectly reflecting, is filled with water (∈dex=4/3) upto a height = 40 cm . A point object in air above is moving towards the water surface with a constant speed =4m/s What is the relative speed of its final image (∈m/s), as seen by the object itself, at a moment when the object is 30 cm above the water surface?
View SolutionA point source of light is 80.0cm below the surface of a body of water. Find the diameter of the circle at the surface through which light emerges from the water.
View SolutionConsider the situation shown in figure. Water (μ=4/3) is filled in a beaker up to a height of 10cm.
A plane mirror is fixed at a height of 5 cm from the surface of water. Distance of image from the mirror after reflection from it of an object O at the bottom of the beaker is
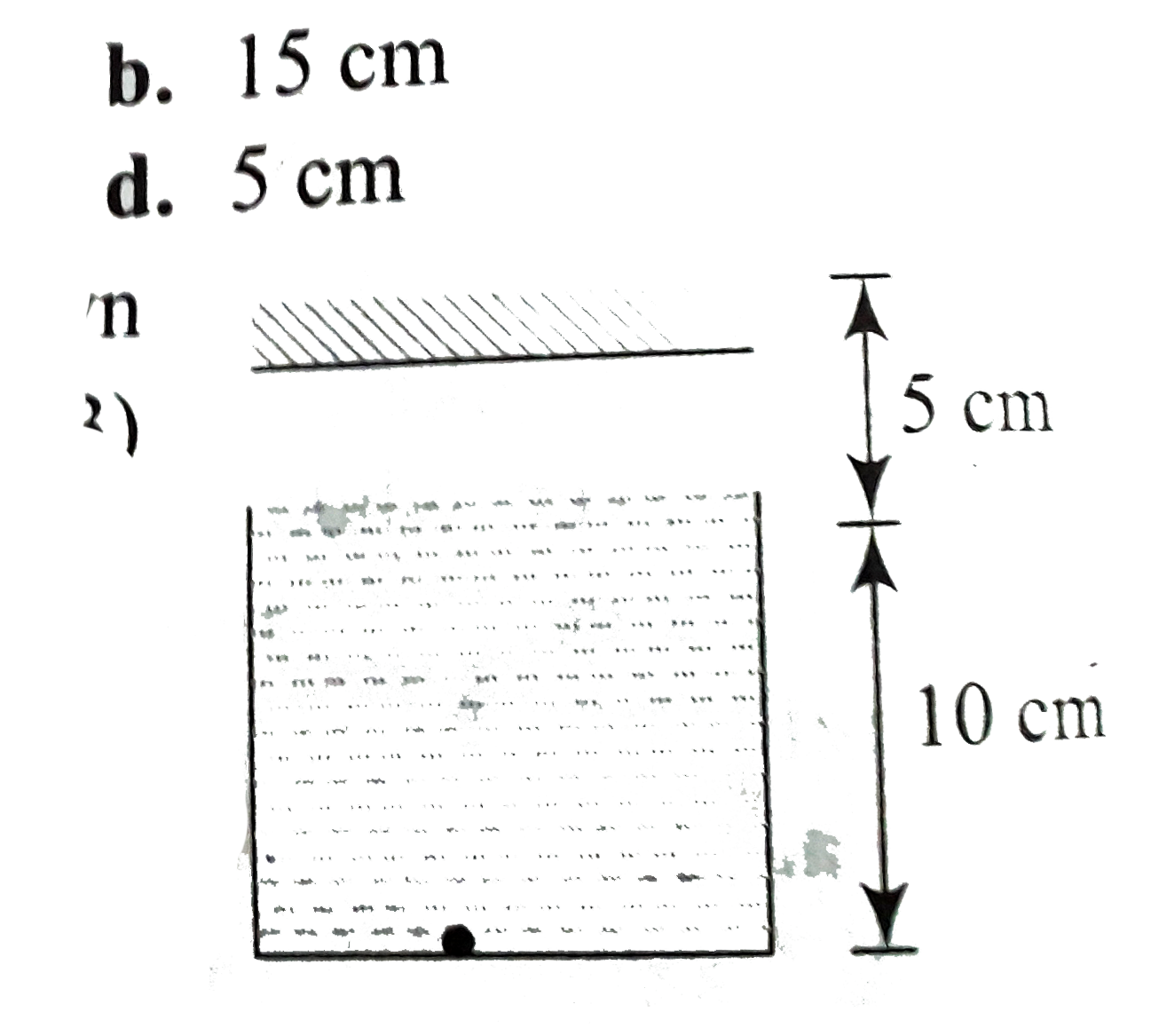
View SolutionA tank is filled with water up to a height H. Water is allowed to come out of a hole P in one of the walls at a depth D below the surface of water. Express the horizontal distance x in terms of H and D
 .
.
View Solution