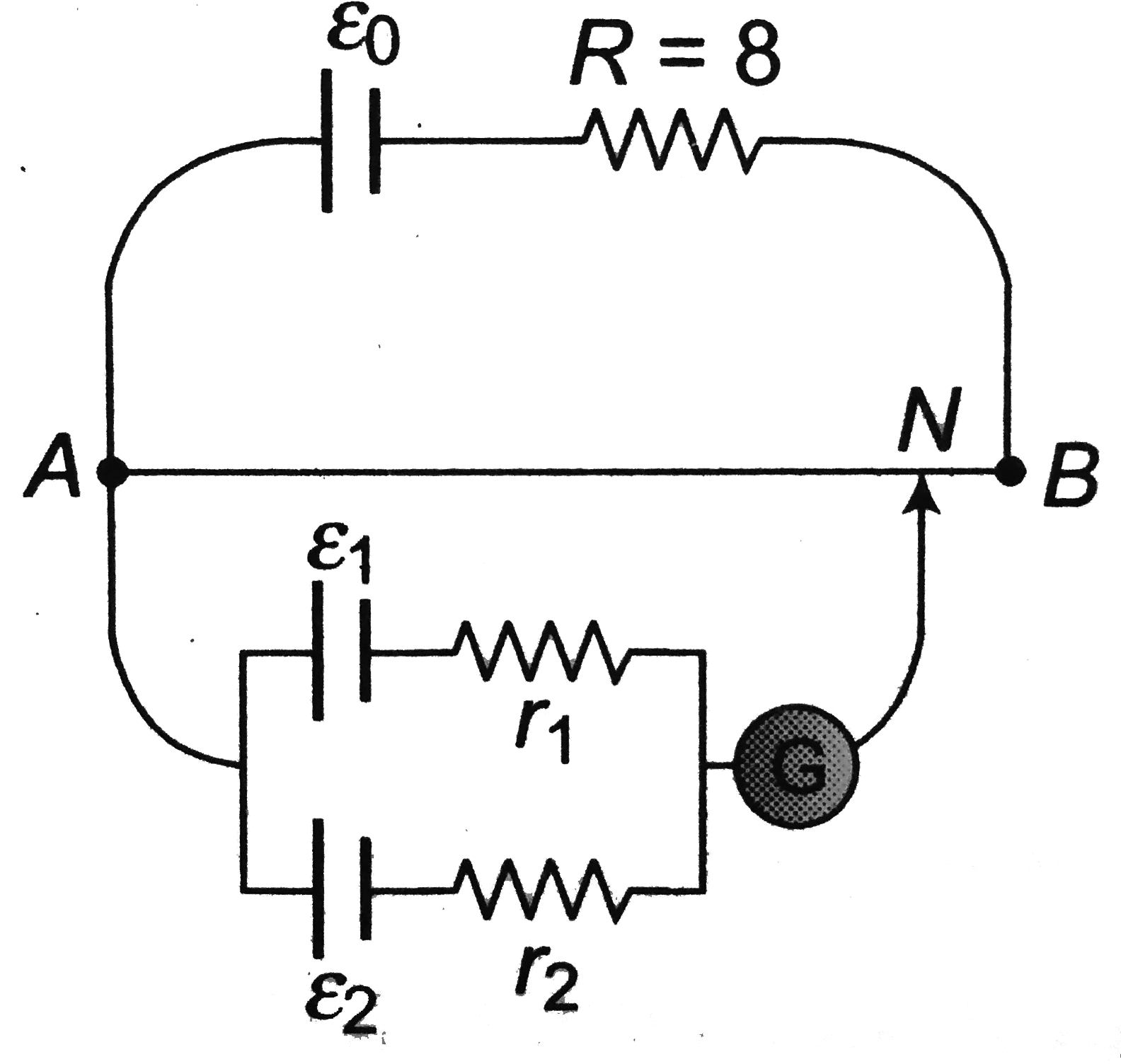
A battery of emf E0=12V is connected across a 4m long uniform wire having resistance 4Ωm. The cells of small
emfs ε1=2V and ε2−4V having internal resistance 2Ω and 6Ω respectively, are connected as shown in the figure. If galvanometer shows no deflection at the point N, the distance of point N from teh point A is equal to
1/6m
1/3m
25cm
50cm
The correct Answer is:C
c. RAB=16Ω,VAB=RABε0R+RAB=16×128+16=8V.
Current in the loop of ε1andε2 :
I=ε2+ε1r1+r2=4+22+6=34A
ε1−Ir1=VAB=ANAB(VAB)or2−34×2=AN4×8
or AN=14m=25cm.
Similar Questions
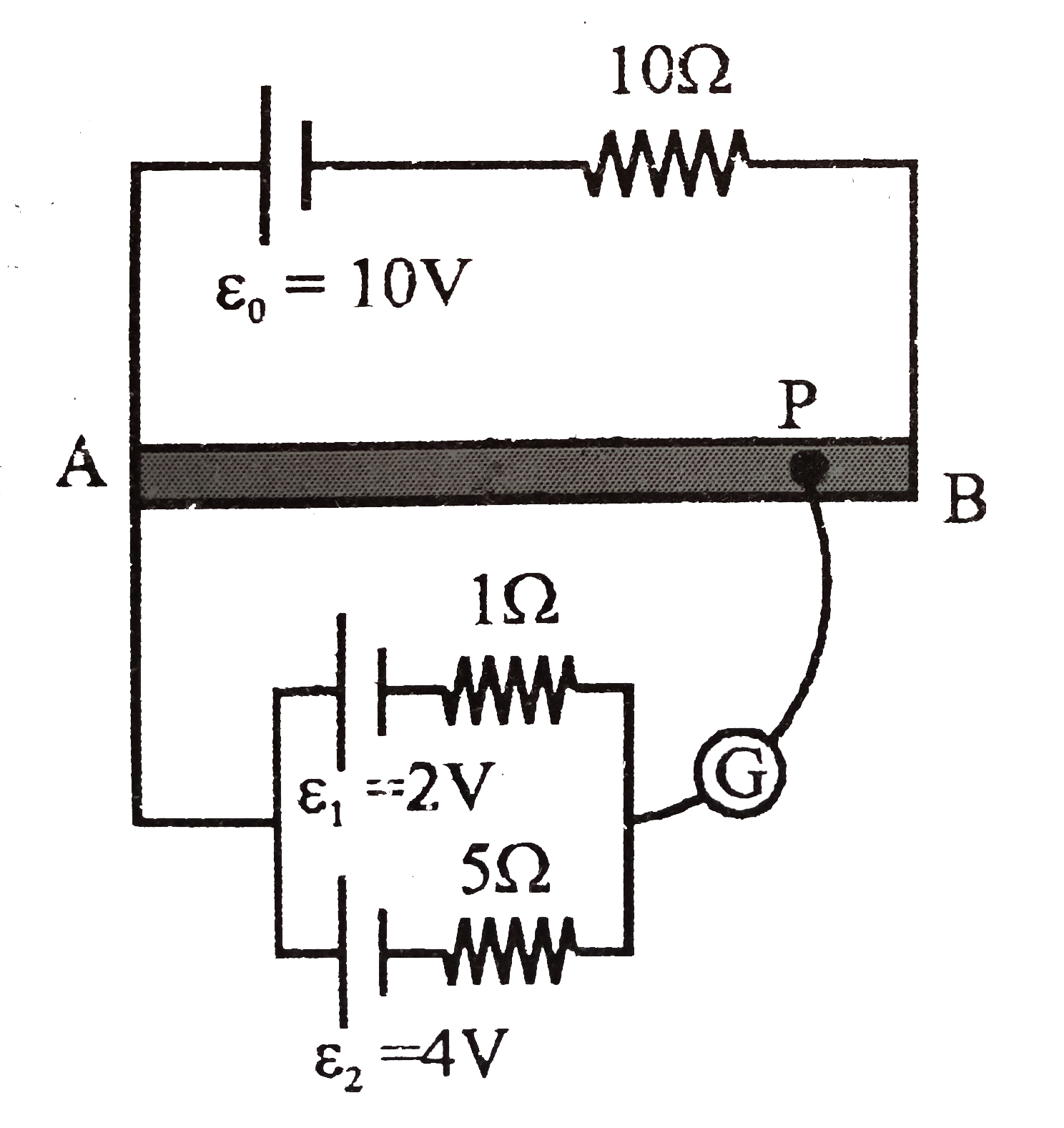
A battery of emf ε0=10V is connected across a 1m long uniform wire having resistance 10Ω/m. Two cells of emf ε1=2V and ε2=4V having internal resistance 1Ω and 5Ω respectively are connected as shown in the figure. If a galvanometer shows no deflection at the point P, find the distance of point P from the point a.
View SolutionTwo cells of emfs 1.5 V and 2.0v having internal resistance 0.2Σ and 0.3 Σ respectively are connected in parallel . Calculate the emf and internal resistance of the equivalent cell .
View Solution
Knowledge Check
A battery of emf E0=12V is connected across a 4m long uniform wire having resistance 4Ω/m. The cell of small emfs ε1=2V and ε2=4V having internal resistance 2Ω and 6Ω respectivley are connected as shown in the figure. If galvanometer shows no diflection at the point N the distance of points N from the point A is equal to
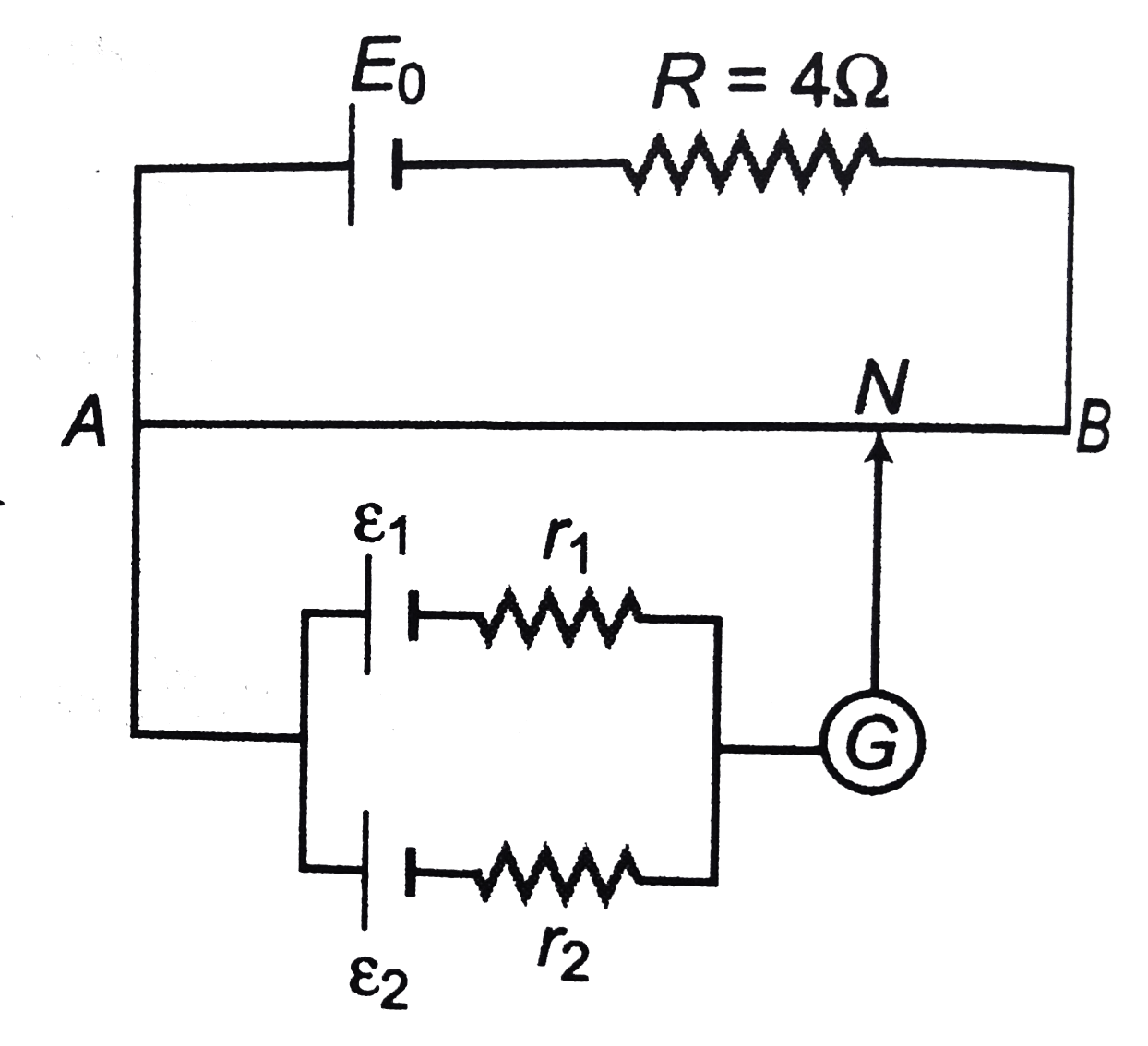 A53mB43mC32mDnone of these
A53mB43mC32mDnone of theseTwo batteries of emfs 2 V and 1 V of internal resistances 1Ωand2Ω respectively are connected in parallel. The effective emf of the combination is
A32VB53VC35VD2VA circuit consists of three batteries of emf E1 = 1 V, E2=2VandE3=3V and internal resistances 1Ω,2Ωand1Ω respectively which are connected in parallel as shown in the figure. The potential difference between points P and Q is
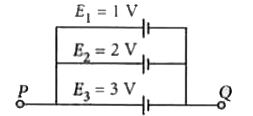 A1.0VB2.0VC2.2VD3.0V
A1.0VB2.0VC2.2VD3.0V
Two cells of emfs 1.5 V and 2.0 V having internal resistances 0.2Ω and 0.3Ω respectively are connected in parallel. Calculate the emf and internal resistance of the equivalent cell.
View SolutionIn the circuit shown in Fig. 4.68, the cells ε1 and ε2 have emf's of 4 V and 8 V and internal resistances of 0.5Ω and 1Ω respectively. Calculate the current in each resistance.
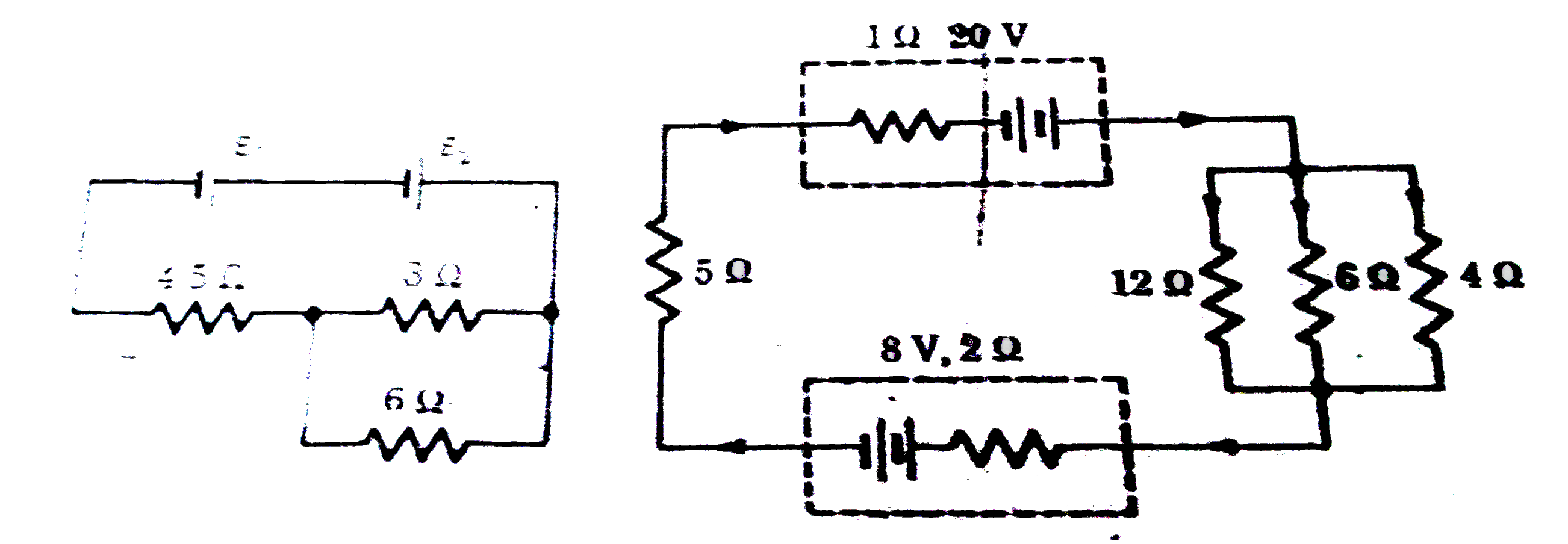
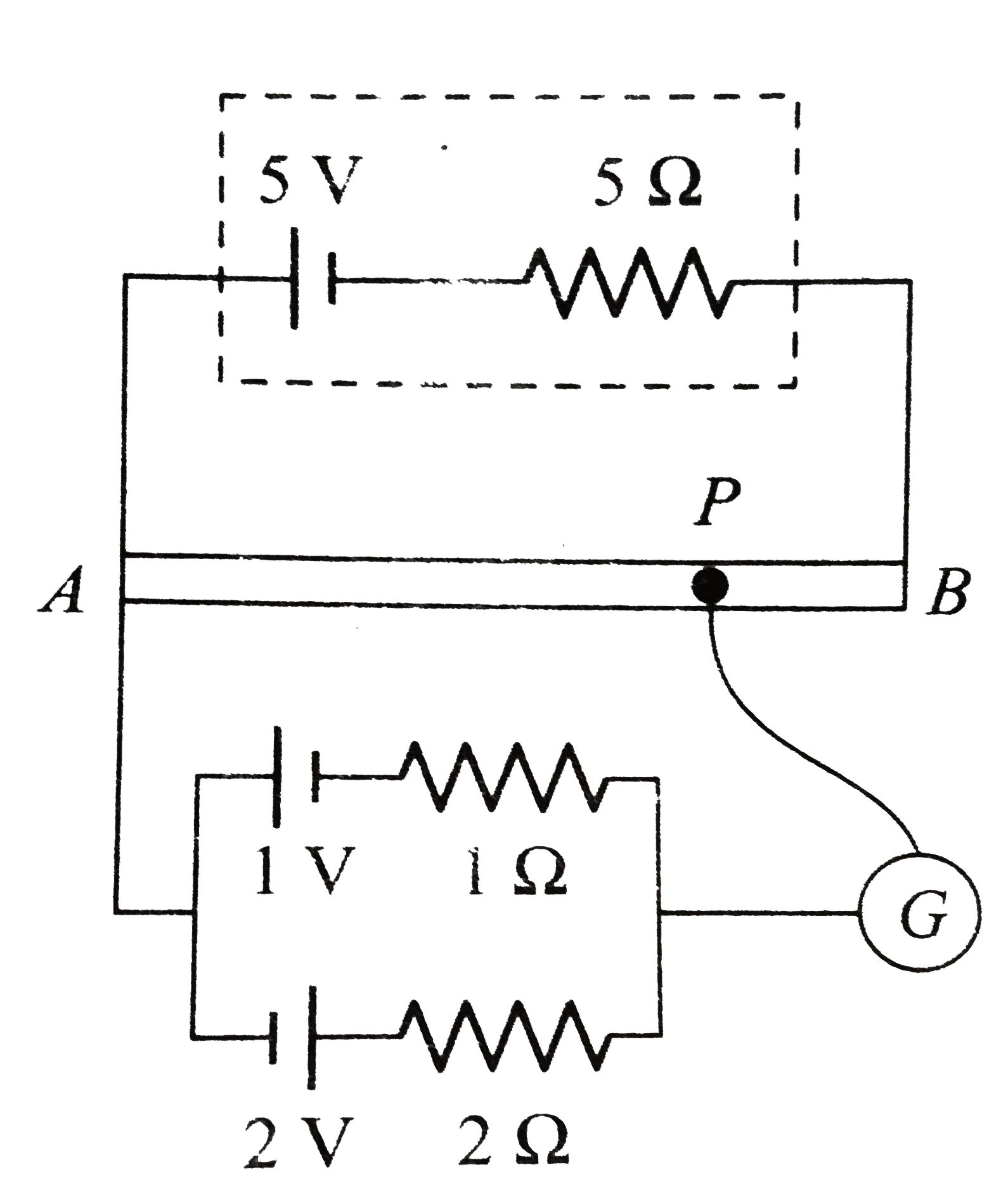
View SolutionA battery of emf ε0=5V and internal resistance 5Ω is connected across a long uniform wire AB of length 1m and resistance per unit length 5Ωm−1. Two cells of ε1=1Vandε2=2V are connected as shown in the figure.
View SolutionTwo cells of e.m.f. 2.5 V and 2.0 V having internal resistance of 1Ω and 2Ω respectively are connected in parallel with similar poles connected together so as to send the current in the same direction through an external resistance of 2Ω. The current in the external resistance is
View SolutionIn the figure, the potentiometer wire of length l=100cm and resistance 9Ω is joined to a cell of emf cell of emf E2=5V and internal resistance r2=2V is connected as shown. The galvanometer is show no deflection when the length AC is

View Solution
