Two mutually perpendicular infinite wires along x-axis and y-axis carry charge densities λ1andλ2. The electric line of force at P is along the line y=x/(√3), where P is also a point lying on the same line. Find λ2/λ1.
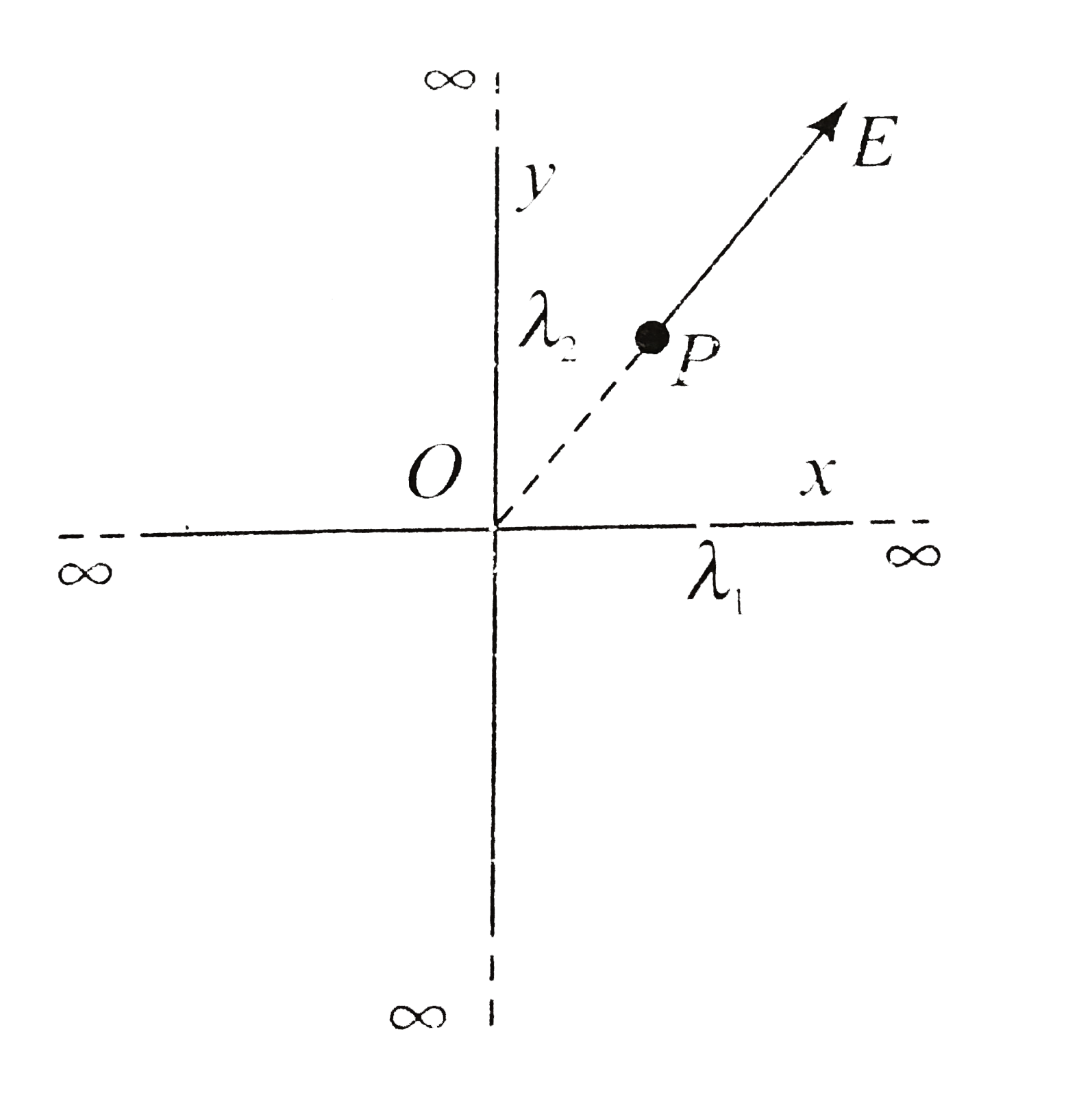
Net electric field is →P
→E=→E1+→E2 due to vertical wire
→E1=λ12πε0y I +y-direction
→E2=λ22πε0x due to vertical wire in x-direction
tanα=E1E2=λ1/2πε0yλ2/2πε0x
or 1√3=λ1xλ2y or λ1λ2=xy√3 or λ1λ2=3
Topper's Solved these Questions
- MISCELLANEOUS VOLUME 3BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - Fill in the blanks
10 Videos
- MISCELLANEOUS VOLUME 3BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - True and False Type
5 Videos
- MISCELLANEOUS VOLUME 3BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - Comprehension Type
94 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - CENGAGE PHYSICSCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Question Bank
4 Videos
- MISCELLANEOUS VOLUME 5BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS VOLUME 5EXERCISE - Integer
12 Videos
Similar Questions
Two mutually perpendicular infinite wires along x -axis and y -axis carry charge densities λ1 and λ2, respectively. The electric line of force at P is along, the line y=1√3x, where P is also a point lying on the same line. Find λ2λ1.
'(##CEN_KSR_PHY_JEE_C18_E01_025_Q12##)'
View SolutionTwo mutually perpendicular infinite wires along x-axis and y-axis carry charge densities λ1 and λ2 (see figure). The 1 electric line of force at P is along the line y=1√3x, where P is also a point lying on the same line, then find λ1/λ2

View Solution
Knowledge Check
Two mutually perpendicular wire carry charge densities λ1andλ2. The electric lines of force makes angle α with second wire, then λ1/λ2 is
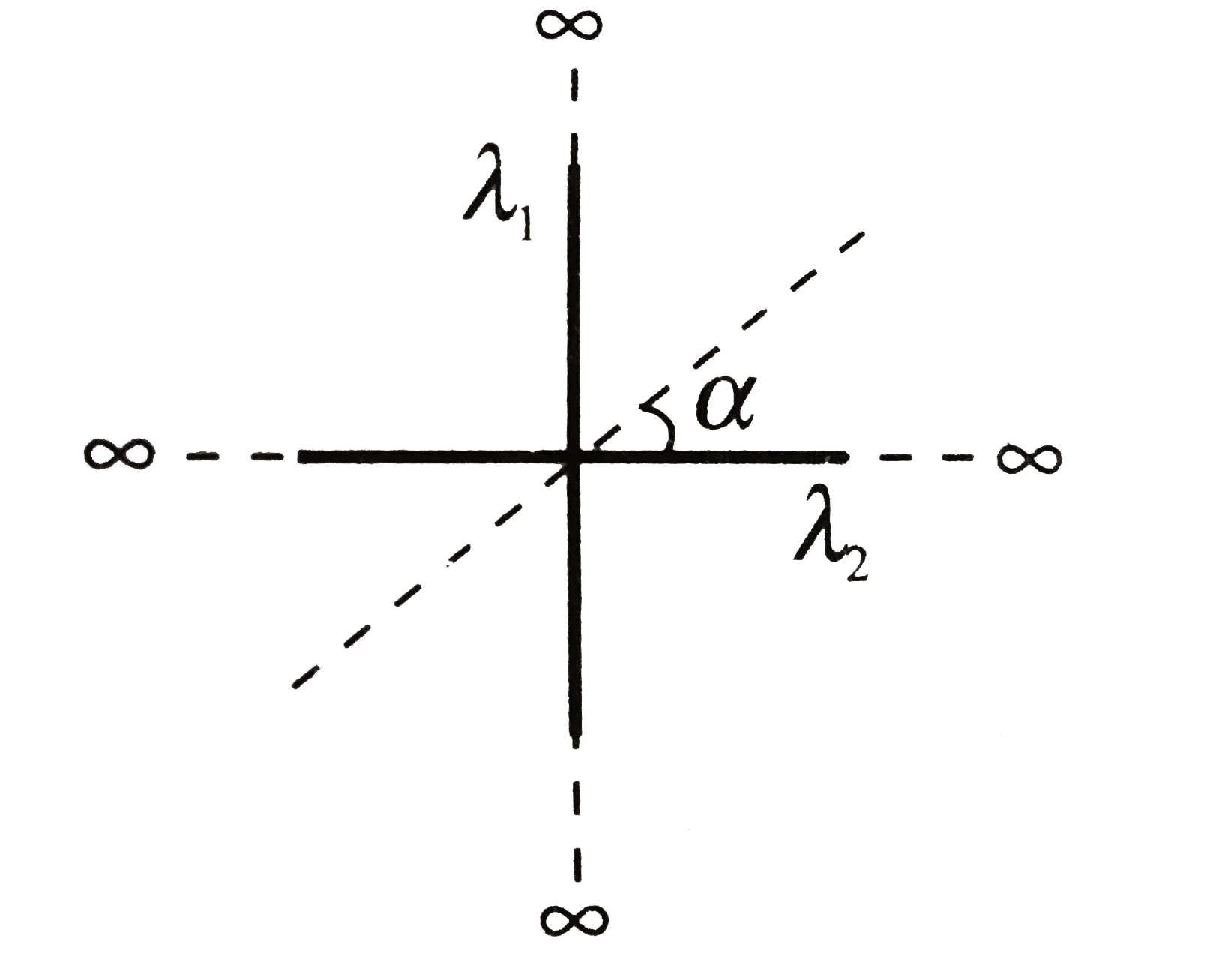 Atan2αBcot2αCsin2αDcos2α
Atan2αBcot2αCsin2αDcos2αFor an infinite line of charge having linear charge density λ lying along x-axis the work done in moving a charge q form C to A along CA is
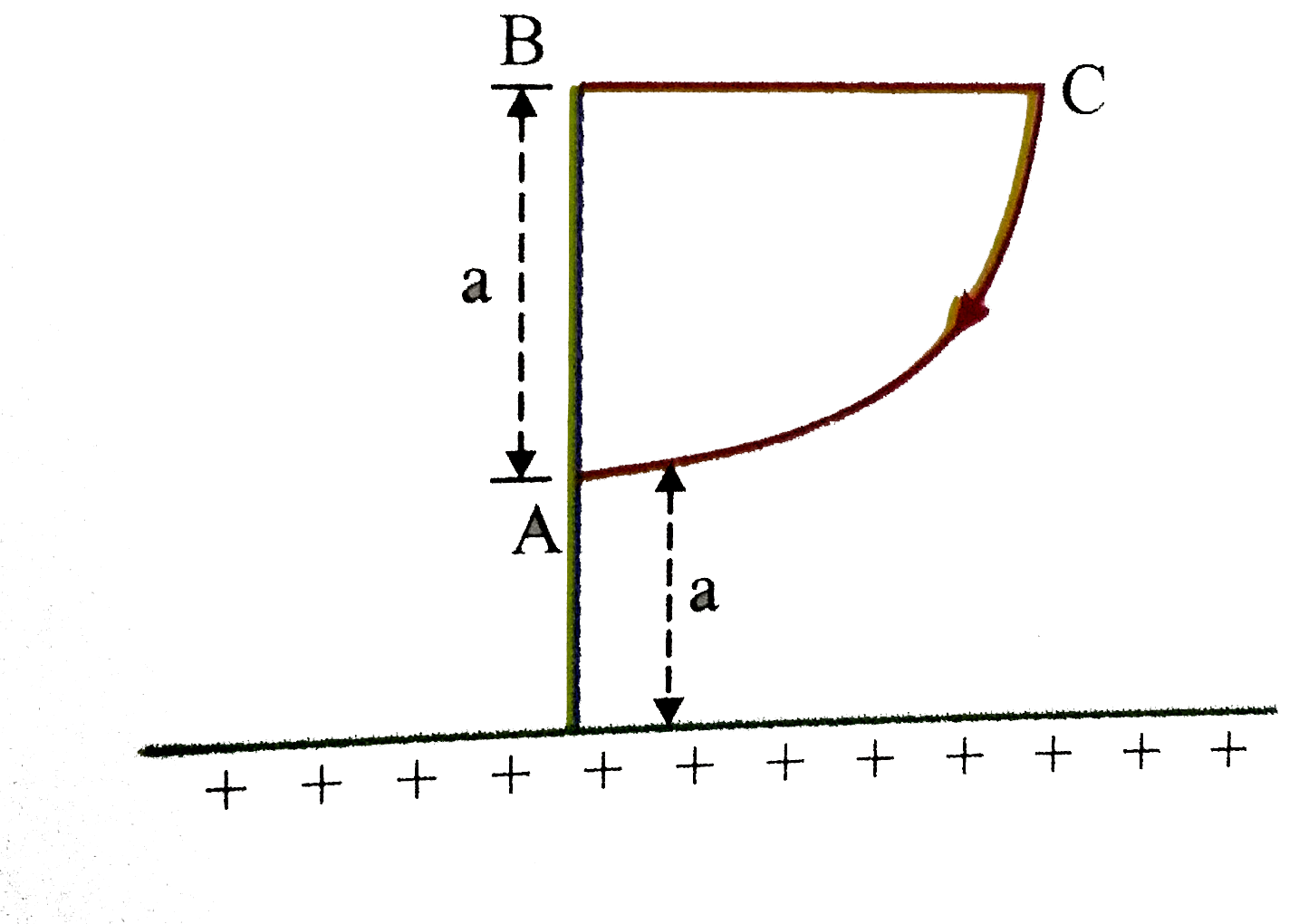 Aqλ2πε0In2Bqλ2πε0In√2Cqλ4πε0In2Dqλ2πε0In(0.5)
Aqλ2πε0In2Bqλ2πε0In√2Cqλ4πε0In2Dqλ2πε0In(0.5)Two uniformly long charged wires with linear densities λ and 3λ are placed along X and Y axis respectively. Determined the slope of electric field at any point on the l ine y=√3x
A3√3B√33√2C13√3D√3
Let there be two lines 2x+3y+λ=0 and λx−3y−1=0 . If the origin lies in the obtuse angle then
View SolutionIf λ1,λ2,λ3 are wavelengths of first 3 lines of balmer series. Find λ1λ3
View SolutionIf x-axis divides the line joining (3,4)and(5,6) in the ratio λ:1 then λ is
View SolutionIf the line passing through the points (4,3)and(2,λ) is perpendicular to the line y=2x+3, then λ is equal to
View SolutionLocus of the image of the point (1,1) in the line (5x–2y−7)+λ(2x−3y+6)=0, λ∈R, is
View Solution