Two point charges of magnitude +qand−q are placed at (−d/2,0,0)and(d/2,0,0) are respectively. Find the equation of the euipotential surface where the potential is zero.
z2+(y−a)2=2a
z2+(y−a)2=27a2/4
z2+y2=15a2/4
none of these
The correct Answer is:C
c. As in none of the options, the x−cordinate appears, so we have to find the locus in the yz plane.
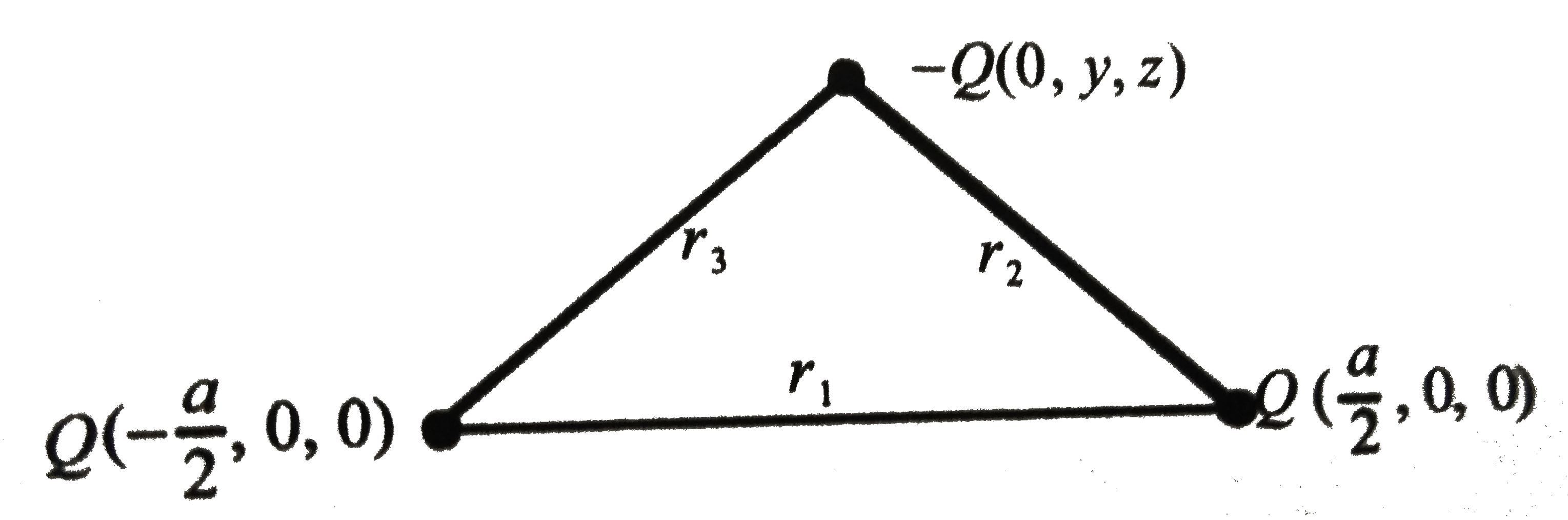
U=kQ2r1−kQ2r2−kQ2r3=0
kQ2⎡⎢
⎢⎣1a−1√(a/2)2+y2+z3−1√(a/2)2+y2+z3⎤⎥
⎥⎦=0
1a=2√a2/4+y2+z2 or y2+z3=15a2/4
Topper's Solved these Questions
- MISCELLANEOUS VOLUME 3Book:CENGAGE PHYSICSChapter:MISCELLANEOUS VOLUME 3Exercise:Multiple Correct Answer type
Explore 109 Videos
- MISCELLANEOUS VOLUME 3Book:CENGAGE PHYSICSChapter:MISCELLANEOUS VOLUME 3Exercise:Assertion and Reason Type
Explore 8 Videos
- MECHANICAL PROPERTIES OF SOLIDSBook:CENGAGE PHYSICSChapter:MECHANICAL PROPERTIES OF SOLIDSExercise:Question Bank
Explore 4 Videos
- MISCELLANEOUS VOLUME 5Book:CENGAGE PHYSICSChapter:MISCELLANEOUS VOLUME 5Exercise:Integer
Explore 12 Videos
Similar Questions
Knowledge Check
Two point charges +q and −q are held fixed at (−d,o) and (d,0) respectively of a x-y coordinate system. Then
A(a) The electric field E at all points on the x-axis has the same directionB(b) Electric field at all points on y-axis is along x-axisC(c) Work has to be done in bringing a test charge from ∞ to the originD(d) The dipole moment is 2qd along the x-axisThree point charge q1=1μC,q2=−2μC and q3=3μC are placed at (1m,0,0),(0,2m,0) and (0,0,3m) respectively. Find the electric potential at the origin.
A2.0×103VB9.0×103VC1.0×103VD10×103V
Two charges q1andq2 are placed at (0,0d)and(0,0−d) respectively. Find locus of points where the potential is zero.
View Solution" Two point charges "+2q" and "-q" are placed on "x" -axis at "(0,0,0)" and "(3,0,0)" respectively,How many points of zero electric potential are there in the plane "X=5?
View SolutionConsider a spherical surface of radius 4 m cenred at the origin. Point charges +q and - 2q are fixed at points A( 2 m, 0,0) and B( 8 m, 0, 0), respectively. Show that every point on the shperical surface is at zero potential.
View SolutionThe point charges q1=1μC,q2=−2μC and q3=3μC are placed at (1m,0,0),(0,2m,0) and (0,0,3m) respectively. Find the electric potential at origin.
View SolutionTwo charges +2q and −3q are fixed at co-ordinates (4m, 0,0 ) and (9m,0,0) , respectively. The distance 'between two points on x -axis (in m ) where the potential is zero is z. Then the value of z2 is (Assume standard reference.point)
View SolutionTwo point charges q and -2q are kept on x-axis at ( -a,0,0) and ( 2a,0,0) . Find the locus of all points wher electric potential is zero. Assume that electric potential at infinite distance from a point charge is zero.
View Solution