Consider the situation shown in figure. We find electric field E at point P using Gauss's law and it comes out to be E=σ/ε0. This electric field is due to

all the amount of charges present on both the plates
all the charges present on positive plate only
positive charge present only inside the Gaussian surface.
positive charge present inside the Gaussian surface plus equal magnitude of negative charge present on negatice plate in front of Gaussian surface.
The correct Answer is:A
According to gauss's law, electric field at any point on gaussian surface is due to all the charges present inside or outside.
Topper's Solved these Questions
- MISCELLANEOUS VOLUME 3Book:CENGAGE PHYSICSChapter:MISCELLANEOUS VOLUME 3Exercise:Multiple Correct Answer type
Explore 109 Videos
- MISCELLANEOUS VOLUME 3Book:CENGAGE PHYSICSChapter:MISCELLANEOUS VOLUME 3Exercise:Assertion and Reason Type
Explore 8 Videos
- MECHANICAL PROPERTIES OF SOLIDSBook:CENGAGE PHYSICSChapter:MECHANICAL PROPERTIES OF SOLIDSExercise:Question Bank
Explore 4 Videos
- MISCELLANEOUS VOLUME 5Book:CENGAGE PHYSICSChapter:MISCELLANEOUS VOLUME 5Exercise:Integer
Explore 12 Videos
Similar Questions
Knowledge Check
Consider the situation when an electric field E is present in a conductor. Acceleration a of the free electrons of the conductor due to this field is
Aa=eEmBa=eEmCa=EemDa=−eEmFigure shows the electric field lines around an electric dipole. Which of the arrows best represents the electric field at point P ?
 A
A B
B C
C D
D
Electric field lines || Gauss law
View SolutionGauss's Law||Calculation OF Electric field using Gauss's Law
View SolutionElectric Field Lines|Electric Flux|Gauss Law
View SolutionMark the correct options about electric field and Gauss's law in a region of space :
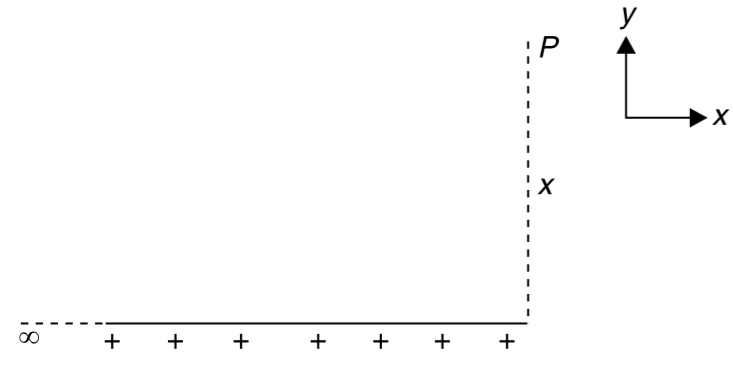
View Solution(a) There is an infinitely long thread uniformly charged with linear charge density λC/m. Using Gauss’ law, calculate the electric field (E0) at a distance x from the thread. (b) Now consider a semi-infinite uniformly charged thread (linear charge density =λ) as shown in figure. Find the y component of electric field at point P in terms of E0. Use simple qualitative argument. (c) For the situation described in (b) calculate the x component of electric field at point P using the method of integration. (d) Find the angle that the electric field at P makes with x direction.
View SolutionAn infinitely thin straight wire has uniform linear charge density λ . Obtain the expression. For the electric field (E) at a point lying at a distance x from the wire, using Gauss' law.
(b) Show graphically the variation of this electric field E as a function of distance x from the wire .
View Solution