In each capacitance C1is6.0μF, and each capacitance C2 is 4.0μF.
 .
.
With 420V across a and b the value of Vc−Vd is.
24.6V
46.7V
18V
72V
The correct Answer is:B
Reduction of the farthest right leg yields
C=(16.0μF+16.0μF+16.0μF)−1=20.0μF=C13
It combines in parallel with C2,i.e.,
C=4.0μF+2.0μF=6.0μF=C1
So the next reduction is the same as the second, leaving 3C1's, in series.
So Ceq=2.0μF=C1/3. For the three capacitors nearest to points a and b:
QC1=CeqV=(2.0×10−6F)(420V)=8.4×10−4C
Vcd=13(4203V)=46.7V.
By symmetry, the total voltage drop over the equivalent capacitance of the part of the circuit from the junctions betweenc and d is (420/3)V and the equivalent capacitance is that of one of those capacitors, i.e., 1/3 of 420/3V.
Topper's Solved these Questions
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICSCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Integer
5 Videos
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICSCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Multile Correct
11 Videos
- ATOMSBOOK - CENGAGE PHYSICSCHAPTER - ATOMSEXERCISE - QUESTION BANK
40 Videos
- CENGAGE PHYSICS DPPBOOK - CENGAGE PHYSICSCHAPTER - CENGAGE PHYSICS DPPEXERCISE - subjective type
51 Videos
Similar Questions
A capacitor of capacitance C1=1μF withstand a maximum voltage of V1=6KV, and another capacitor of capacitance C2=2μF, can with stand a maximum voltage of V2=4KV. If they are connected in series, what maximum voltage will the system withstand?
View SolutionA capaitor of capacitance C1=1.0μF withstands teh maximum voltage V1=6.0kV while a capacitor of capacitance Cs=2.0μF, the maximum voltage Vs=4.0kV. What voltage will the system of these two capacitors withsatand if they are connected in sereis ?
View Solution
Knowledge Check
In each capacitance C1 is 6.0μF, and each capacitance C2 is 4.0μF.
 .
.
The charge on C1 nearest to a when Vab=420V is.A840μCB560μCC600μCD320μCIn each capacitance C1 is 6.0μF, and each capacitance C2 is 4.0μF.
 .
.
The equivalent capacitance of the netwok between ponts a and b is.A2μFB4μFC6μFD8μFA circuit shown in the figure consists of a battery of emf 10V and two capacitance C1 and C2 of capacitances 1.0μF and 2.0μF respectively. The potential difference VA−VB is 5V
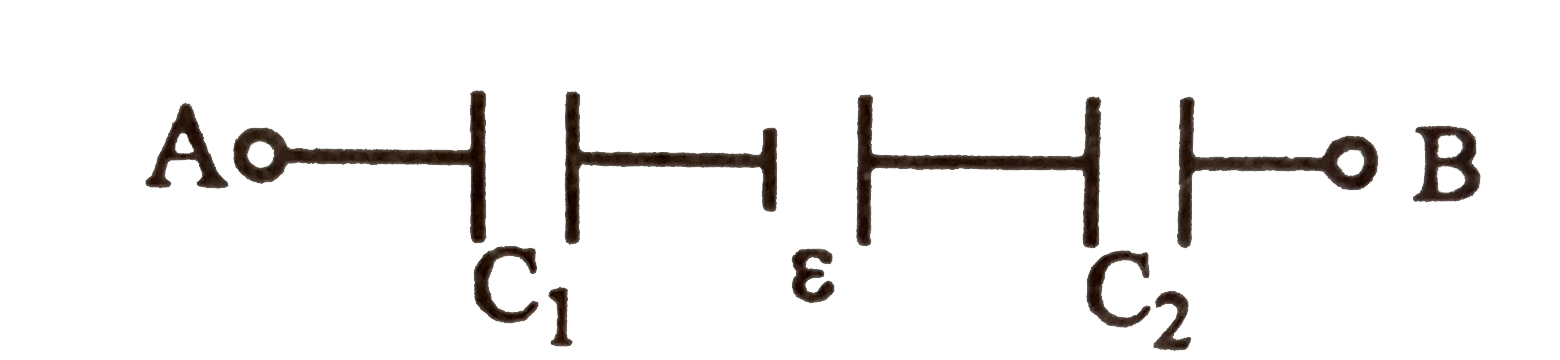 ACharge on capacitor C1 is equal to charge on capacitor C2BVoltage across capacitor C1 is 5V.CVoltage across capacitor C2 is 10VDEnegry stored in capacitor C1 is two times the enegry stored in capacitor C2.
ACharge on capacitor C1 is equal to charge on capacitor C2BVoltage across capacitor C1 is 5V.CVoltage across capacitor C2 is 10VDEnegry stored in capacitor C1 is two times the enegry stored in capacitor C2.
A circuit has a section Ab shown in fig. The emf of the source equals E=10V, the capacitances are equal to C1=1.0μF and C2=2.0μF, and the potential difference φA−φB=5.0V. Find the voltage across each capacitor.

View SolutionIn the given network capacitance, C1=10μF,C2=5μF and C3=4μF. What is the resultant capacitance between A and B?
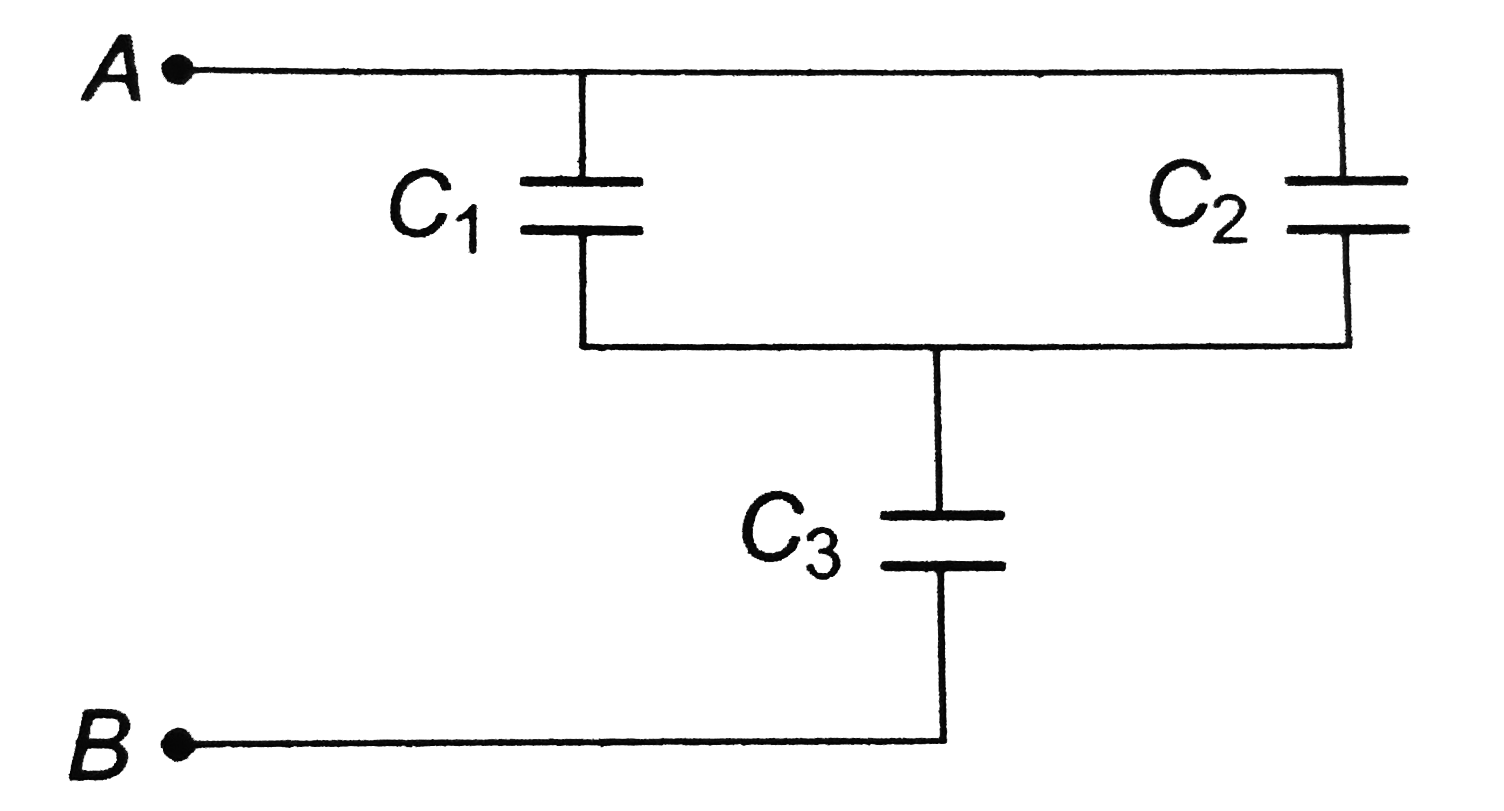
View SolutionA capacitor of capacitance C1=1μF can withstand maximum voltage V1=6kV and another capacitor of capacitance C2=3μF can withstand maximum voltage V24kV. When the two capacitors are connected in series, the combined system can withstand a maximum voltage of
View SolutionCondensers of capacity 4μF, 5μF and 6μF are connected first in series . The effective capacitance is C1. When they are connected in parallel, the effective capacitance is C2. Then the ratio C2/C1 will be
View SolutionA capacitor of capacitance C is charged to a potential difference V0. The charged battery is disconnected and the capacitor is connected to a capacitor of unknown capacitance Cx. The potential difference across the combination is V. The value of Cx should be
View Solution
