In the previous question, if V is the electric potential of the first sphere, what would be the electric potential of the second sphere ?
2 V
V/2
V/4
V
The correct Answer is:a
To find the electric potential of the second sphere given that the electric potential of the first sphere is V, we can follow these steps:
Step 1: Understand the relationship between electric field and potential
The electric potential V at a distance r from a charged sphere is related to the electric field E by the formula:
V=−∫E⋅dr
For a uniformly charged sphere, the electric field outside the sphere is given by:
E=σϵ0
where σ is the surface charge density and ϵ0 is the permittivity of free space.
Step 2: Determine the potential at the first sphere
Let’s denote the potential at the first sphere (radius r) as V1. The potential at this radius can be expressed as:
V1=Q4πϵ0r
where Q is the total charge on the sphere.
Step 3: Calculate the potential at the second sphere
Now, if the radius of the second sphere is 2r, the potential V2 at this radius can be calculated similarly:
V2=Q4πϵ0(2r)=12⋅Q4πϵ0r=12V1
Step 4: Relate V2 to V
Since we know V1=V (the electric potential of the first sphere), we can express the potential of the second sphere as:
V2=12V
Conclusion
Thus, the electric potential of the second sphere is:
V2=12V
---
The electric potential V at a distance r from a charged sphere is related to the electric field E by the formula:
V=−∫E⋅dr
For a uniformly charged sphere, the electric field outside the sphere is given by:
E=σϵ0
where σ is the surface charge density and ϵ0 is the permittivity of free space.
Let’s denote the potential at the first sphere (radius r) as V1. The potential at this radius can be expressed as:
V1=Q4πϵ0r
where Q is the total charge on the sphere.
Now, if the radius of the second sphere is 2r, the potential V2 at this radius can be calculated similarly:
V2=Q4πϵ0(2r)=12⋅Q4πϵ0r=12V1
Since we know V1=V (the electric potential of the first sphere), we can express the potential of the second sphere as:
V2=12V
Thus, the electric potential of the second sphere is:
V2=12V
Topper's Solved these Questions
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC POTENTIALEXERCISE - Multiple Correct
10 Videos
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC POTENTIALEXERCISE - Comprehension
31 Videos
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC POTENTIALEXERCISE - Subjective
28 Videos
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - MCQ s
38 Videos
- ELECTRICAL MEASURING INSTRUMENTSBOOK - CENGAGE PHYSICSCHAPTER - ELECTRICAL MEASURING INSTRUMENTSEXERCISE - M.C.Q
2 Videos
Similar Questions
Knowledge Check
The electric potential inside a conducting sphere
Aincreases from cente to surfaceBdecreases from cente to surfaceCremians constant from centre to surfaceDis zero at every point insideThere is a uniformly charged non conducting solid sphere made of material of dielectric constant one. If electric potential at infinity be zero. Then the potential at its surface is V. if we take electric potential at its surface to be zero. Then the potential at the centre will be
A3V2BV2CVDzeroElectric potential V and electric flux ϕ are
Aboth vectorsBboth scalarsCV is scalar, ϕ is vectorDV is vectoe, ϕ is scalar
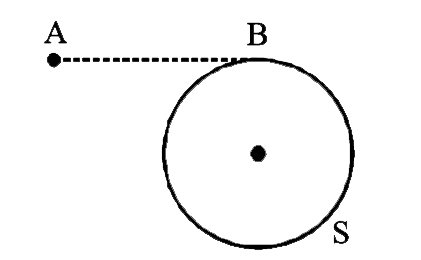
7S is a solid neutral conducting sphere. A point charge q of 1 × 10–6 C is placed at point A. C is the centre of sphere and AB is a tangent. BC = 3m and AB = 4m.
(1) The electric potential of the conductor is 1.8 kV
(2) The electric potential of the conductor is 2.25 kV
(3) The electric potential at B due to induced charges on the sphere is – 0.45 kV
(4) The electric potential at B due to induced charges on the sphere is 0.45 kV
View SolutionElectric potential V and electric flux ϕ are
View SolutionIf the radius of a sphere is doubled, what is the ratio of the volume of the first sphere to that of the second?
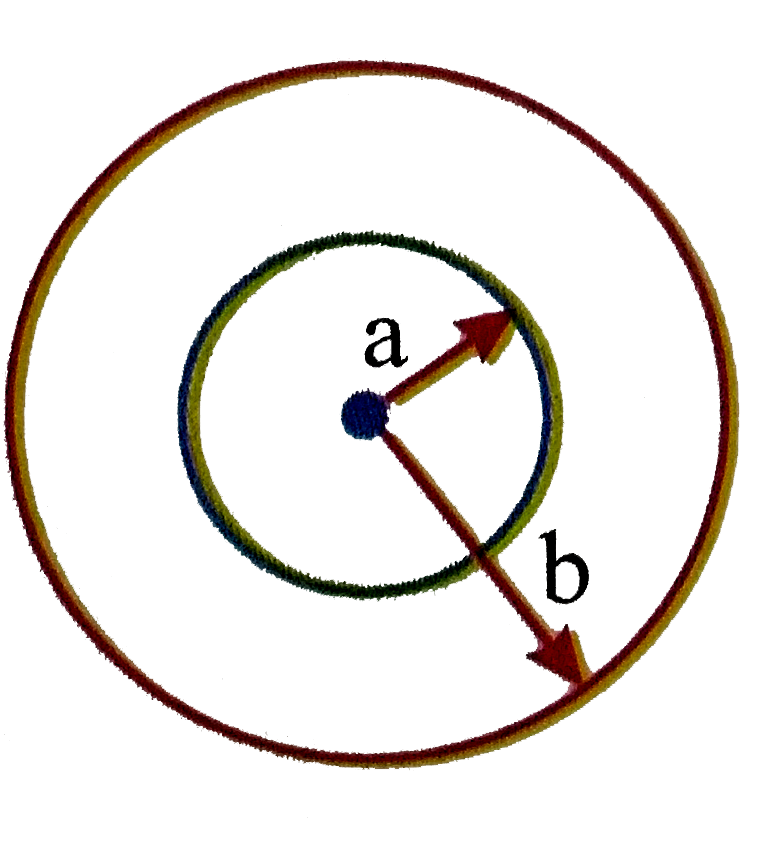
View SolutionIf the electric potential of the inner metal sphere is 10 volt & that of the outer shell is 5 volt then the potential at the centre will be
View SolutionStatement :-A:-Electrical potential may exist at a point where the electrical potential field is zero.
Statement B:- Electrical field may exist at a point where the electircal potential is zero.
Statement C:- The electric potential inside a charge conducting sphere is constant .
View Solution