Two fixed point charges $+ 4 e$ and $+ e$ units are separated by a distance a. Where should a third point charge be placed for it to be in equilibrium?

Video Solution

Text Solution

Generated By DoubtnutGPT

To find the position where a third point charge should be placed for it to be in equilibrium between two fixed point charges $+ 4 e$ and $+ e$ separated by a distance $a$ , we can follow these steps:
Step 1: Understand the Configuration
We have two fixed charges:
- Charge $Q_{1} = + 4 e$ located at point A.
- Charge $Q_{2} = + e$ located at point B, a distance $a$ away from $Q_{1}$ .
We need to place a third charge $Q$ such that the net force acting on it due to $Q_{1}$ and $Q_{2}$ is zero.
Step 2: Identify Possible Positions for Charge $Q$
The third charge $Q$ can be placed:
1. Between $Q_{1}$ and $Q_{2}$ .
2. To the left of $Q_{1}$ .
3. To the right of $Q_{2}$ .
Step 3: Analyze the Forces
1. Between $Q_{1}$ and $Q_{2}$ :
- Let the distance from $Q_{1}$ to $Q$ be $x$ .
- Then the distance from $Q_{2}$ to $Q$ will be $a - x$ .
2. Force Calculations:
- The force $F_{1}$ acting on $Q$ due to $Q_{1}$ is given by:
$F_{1} = k \frac{| Q \cdot Q_{1} |}{x^{2}} = k \frac{Q \cdot 4 e}{x^{2}}$
- The force $F_{2}$ acting on $Q$ due to $Q_{2}$ is given by:
$F_{2} = k \frac{| Q \cdot Q_{2} |}{(a - x)^{2}} = k \frac{Q \cdot e}{(a - x)^{2}}$
Step 4: Set Forces Equal for Equilibrium
For the charge $Q$ to be in equilibrium, the magnitudes of the forces must be equal:
$F_{1} = F_{2}$
This gives us:
$k \frac{Q \cdot 4 e}{x^{2}} = k \frac{Q \cdot e}{(a - x)^{2}}$
We can cancel $k$ and $Q$ (assuming $Q \neq 0$ ):
$\frac{4 e}{x^{2}} = \frac{e}{(a - x)^{2}}$
Dividing both sides by $e$ :
$\frac{4}{x^{2}} = \frac{1}{(a - x)^{2}}$
Step 5: Cross Multiply and Solve
Cross multiplying gives:
$4 (a - x)^{2} = x^{2}$
Expanding the left side:
$4 (a^{2} - 2 a x + x^{2}) = x^{2}$
This simplifies to:
$4 a^{2} - 8 a x + 4 x^{2} = x^{2}$
Rearranging gives:
$3 x^{2} - 8 a x + 4 a^{2} = 0$
Step 6: Apply the Quadratic Formula
Using the quadratic formula $x = \frac{- b \pm \sqrt{b^{2} - 4 a c}}{2 a}$ :
Here, $a = 3, b = - 8 a, c = 4 a^{2}$ :
$x = \frac{8 a \pm \sqrt{(- 8 a)^{2} - 4 \cdot 3 \cdot 4 a^{2}}}{2 \cdot 3}$
Calculating the discriminant:
$x = \frac{8 a \pm \sqrt{64 a^{2} - 48 a^{2}}}{6} = \frac{8 a \pm \sqrt{16 a^{2}}}{6} = \frac{8 a \pm 4 a}{6}$
Step 7: Find the Two Possible Values for $x$
1. $x = \frac{12 a}{6} = 2 a$ (not valid as it exceeds $a$ )
2. $x = \frac{4 a}{6} = \frac{2 a}{3}$
Conclusion
The third charge should be placed at a distance $\frac{2 a}{3}$ from $Q_{1}$ (or $\frac{a}{3}$ from $Q_{2}$ ) for it to be in equilibrium.

Updated on:7/8/2024

Two points charges $+ 4 q$ and $+ q$ are separated by distance $r$ , where should a third point charge $Q$ be placed that the whole system remains in equilibrium
A $\frac{2 r}{3}$ from $q$
B $\frac{r}{3}$ from $q$
C $\frac{r}{2}$ from $q$
D $\frac{r}{3}$ from $4 q$
Two point charges Q and $- Q / 4$ are separated by a distance $X$ . Then .
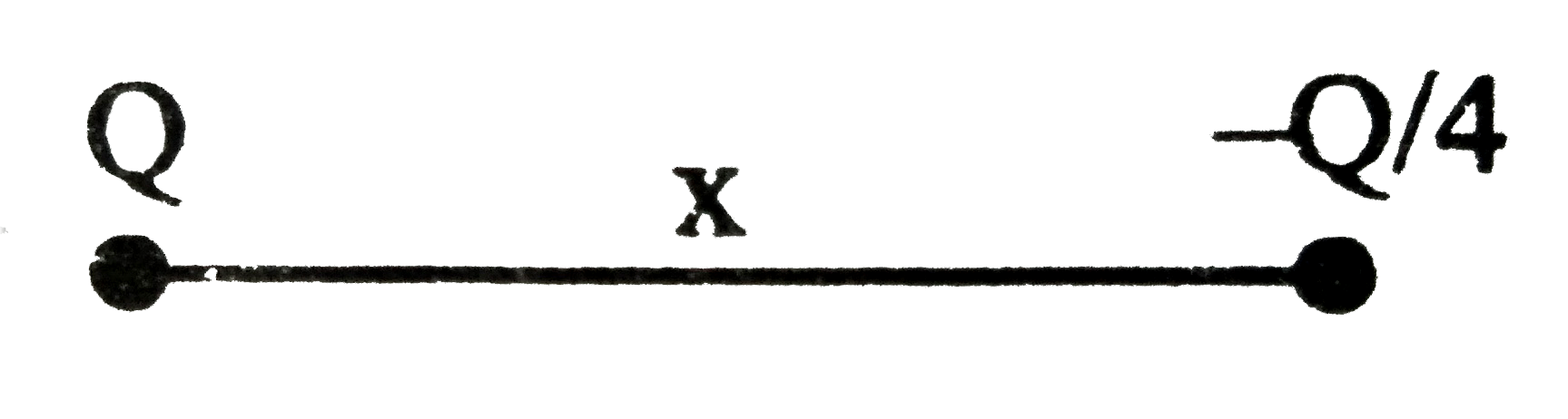Apotential is zero at a point on the axis which is $x / 3$ on the right side of the cahrge $- q / 4$
Bpotential is zero at a point on the axis which is $x / 5$ on the left side fo the charge $- Q / 4$
Celectric field is zero at a point on the axis which is at a distance $x$ on the right side fo the charge $- Q / 4$
Dthere exist two points on the axis where electric field is zero.
Two point metal charges $- q$ and $+ 2 q$ are placed at a certain distance apart.Where should a third point charge be placed so that it is in equilibrium?
Aon the line joining the two charges on the right of $+ 2 q$
Bon the line joining the two charge on the left of $- q$
Cbetween $- q$ and $+ 2 q$
Dat any point on the right bisector of the 1 line joining $- q$ and $+ 2 q$