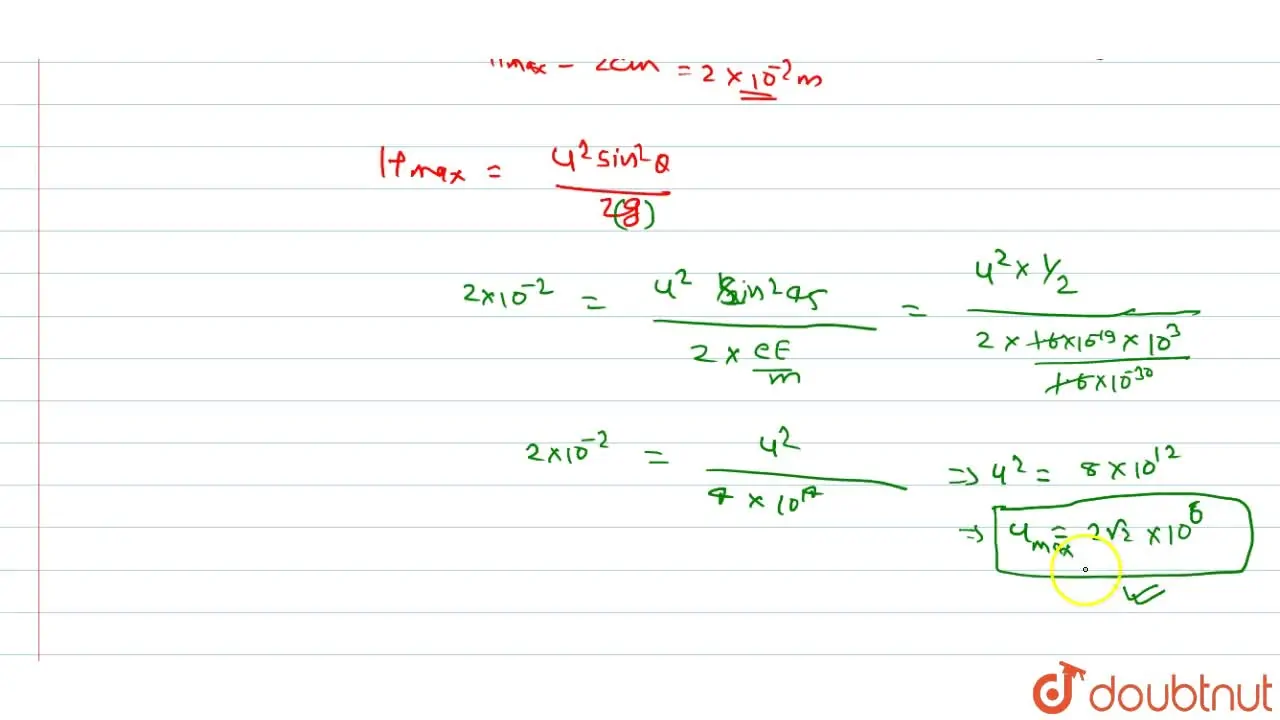
A partical having charge that of an electron and mass 1.6×10−30kg is projected with an initial speed u at the angle 45∘ to the horizontal from the lower plate of a parallel-plate capacitor as shown in fig. The plates are suffiecienly long and have a separation of 2 cm, find the maximum value of the velocity of he particle so that it does not hit the upper plate. Take the electric field between the plates as 103Vm−1 directed upward.

Resolving the velosity of the particle parallel and perpendicular to the plate, we get
u∣∣=ucos45∘=u√2andu⊥=usin45∘=u√2
force on the charged particle in the downward direction and normal to the plate is eE. Therefore, acceleration a=eE/m, where m is the mass of the charged paricle. the particle will not hit the upper plate if the velocity component normal to hte plate becomes zero before reaching it . i.e. 0=−u2⊥−2ay with y≤d, where d is the distnce between the plates. therefore, the maximum velocity for the particle not to hit the upper plate (for this y=d=2cm)is
u⊥=√2ay=√2×1.6×10−19×103×2×10−21.6×10−30
=2×106ms−1
or, umax=u⊥/cos45∘=2√2×106ms−1.
Related Playlists
- BOOK - CENGAGE PHYSICSCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Solved examples10videos
COULOMB LAW AND ELECTRIC FIELD
- BOOK - CENGAGE PHYSICSCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Exercises58videos
COULOMB LAW AND ELECTRIC FIELD
- BOOK - CENGAGE PHYSICSCHAPTER - COMMUNICATION SYSTEMEXERCISE - QUESTION BANK19videos
COMMUNICATION SYSTEM
- BOOK - CENGAGE PHYSICSCHAPTER - Current ElectricityEXERCISE - QUESTION BANK40videos
Current Electricity
Similar Questions
A parallel plate capacitor is charged. If the plates are pulled apart
View SolutionA positive charge q is given to each plate of a parallel plate air capacitor having plate area A and plate separation d, then
View SolutionDeduce an expression for the capacitance of a parallel plate capacitor having plate area 'A' and plate separation 'd'.
View SolutionA parallel plate capacitor is charged and then isolated. On increasing the plate separation
View SolutionCalculate capacitance of a parallel plate capacitor with area of each plate 1 cm2 and separation 1 mm.
View SolutionThe plates of a parallel plate capacitance 1.0 F are separated by a distance d =1 cm. Find the plate area .
View SolutionA parallel plate capacitor is charged and then isolated. On increasing the plate separation
View SolutionAn electron enters the region between the plates of a parallel plate capacitor at an angle θ to the plates. The plate width is l, the plate separation is d. The electron follows the path shown just missing the upper plate. Neglect gravity. Then,
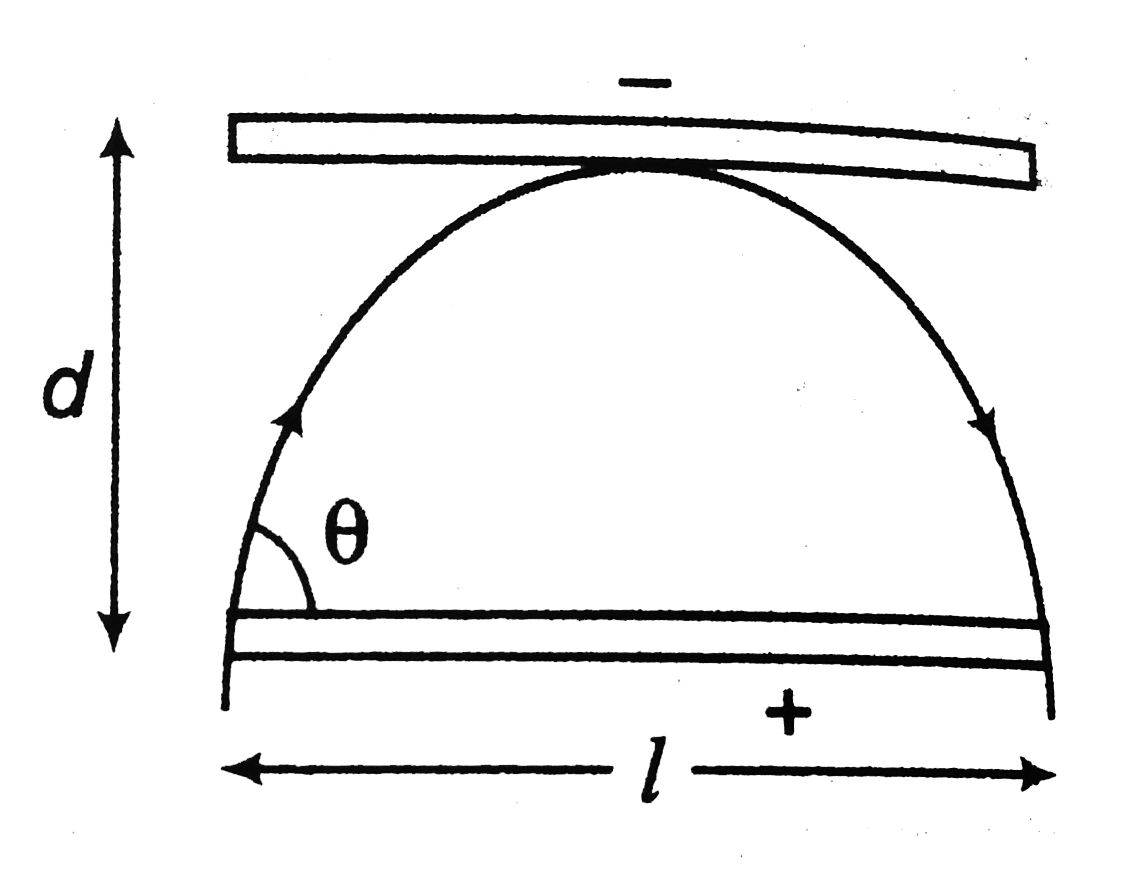 View Solution
View SolutionA parallel plate capacitor is constructed with plates of area 0.0280m2 and separation 0.550mm. Find the magnitude of the charge on each plate of this capacitor when the potential between the plates is 20.1V
 View Solution
View SolutionThe separation between the plates of a charged parallel-plate capacitor is increased. The force between the plates
View Solution