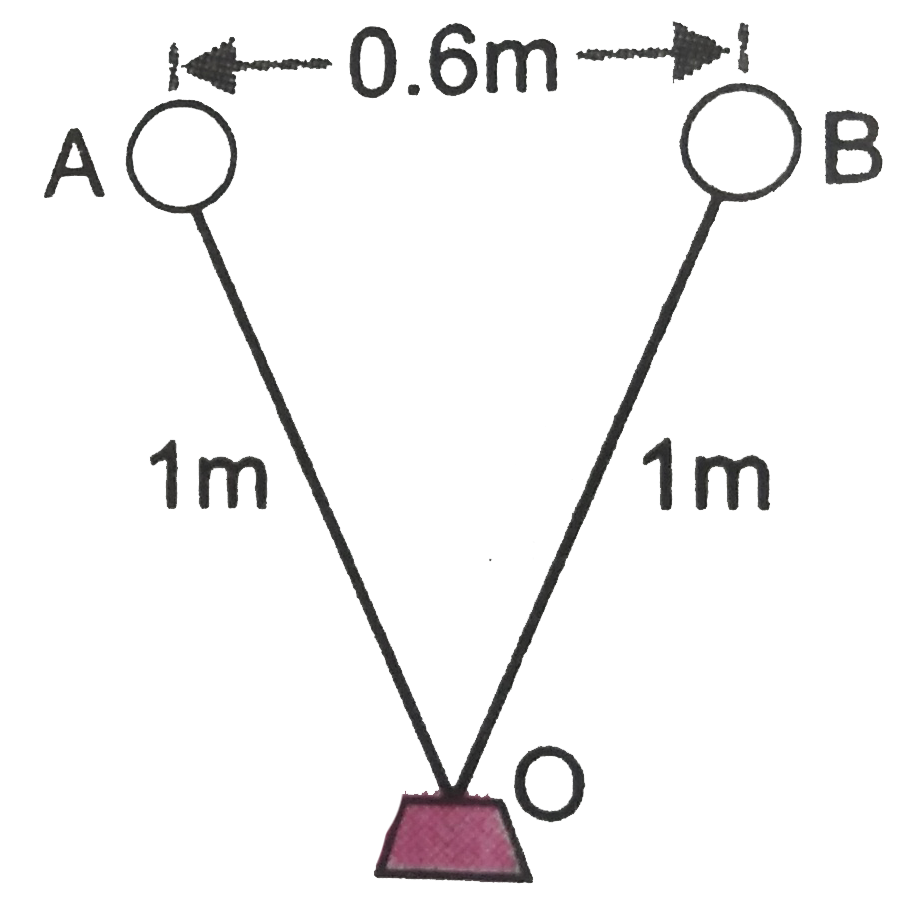
Two identical He-filled spherical balloons each carrying a charge q are tied to a weight W with strings and float in equlibrium, as shown in figure.
(i) Find the magnitude of q, assuming that the charge on each balloon acts as if it were concentrated at the center.
(ii) Find the volume of each balloon. Take the density of He as ρHe and the density of air as ρa. Ignore the weight of the unfilled balloons .
 ,
,
(i) 2Tcosθ=W,Tsinθ=F
or tanθ2=FWorF=Wtanθ2
or, q24πε0(2x)2=Wtanθ2 or q=√8Wtanθπε0x2
(ii) Tcosθ+mg=B⇒W2+V(ρHe)g=Vρag
or V=W2(ρa−ρHe)g.
Topper's Solved these Questions
- COULOMB LAW AND ELECTRIC FIELDBook:CENGAGE PHYSICSChapter:COULOMB LAW AND ELECTRIC FIELDExercise:Solved examples
Explore 10 Videos
- COULOMB LAW AND ELECTRIC FIELDBook:CENGAGE PHYSICSChapter:COULOMB LAW AND ELECTRIC FIELDExercise:Exercises
Explore 58 Videos
- COMMUNICATION SYSTEMBook:CENGAGE PHYSICSChapter:COMMUNICATION SYSTEMExercise:QUESTION BANK
Explore 19 Videos
- Current ElectricityBook:CENGAGE PHYSICSChapter:Current ElectricityExercise:QUESTION BANK
Explore 40 Videos
Similar Questions
Two similar helium-filled spherical balloons tied to a 5g weight with strings and each carrying a charge q floats in equilibrium as shown in figure−1.457. Find (a) the magnitude of q, assuming that the charge on each balloon acts as ifit were concentrated at the centre and (b) the volume of each balloon. Neglect weight of the unfilled balloons and take density ofair 1.29kg/m3 and the density of helium in the balloons 0.2kg/m3:

View SolutionTwo identical helium filled balloons A and B fastened to a weight of 5 g by threads floats in equilibrium as shown in figure. Calculate the charge on each balloons, assuming that they carry equal charge.
View Solution
Knowledge Check
_E01_093_Q01.png)
A ballong filled with air is weighted so that it barely floats in water as shown in figure. When it is pushed down so that it gets submerged a short distance in water then the balloonAwill come up again to its former positionBwill remain in the position it is leftqCwill sink to the bottomDwill emerge out of liquid.Statement-1: A gas filled balloon stops rising after it has attained a certain height in the sky.
Statement-2: At the highest point the density of air is such that the buoyant force on the balloon just equals its weight.AStatement-1, is true, Statement-2 is true, Statement-2 is correct explanation for statement-1BStatement-1 is true, statement-2 is true, statement-2 is NOT a correct explantion for statement-1Cstatement-1 is true, statement-2 is falseDstatement-1 is false statement-2 is true
Two identical helium filled ballons A and B fastended to a weight of 5 gram by threads float in equilibrium as shown in fig. Calculate the charge on each ballon, assuming that they carry equal charges.
View SolutionTwo identical small charged spheres, each having a mass m, hang in equilibrium as shown in fig. the ligth of each string is l, and the angle made by any string with the vertical is θ. Find the magnitude of the charge on each sphere.
View SolutionTwo indentical pith balls, each carrying charge q, are suspended from a common point by two strings of equal length l. Find the mass of each ball if the angle between the strings is 2θ in equilibrium.
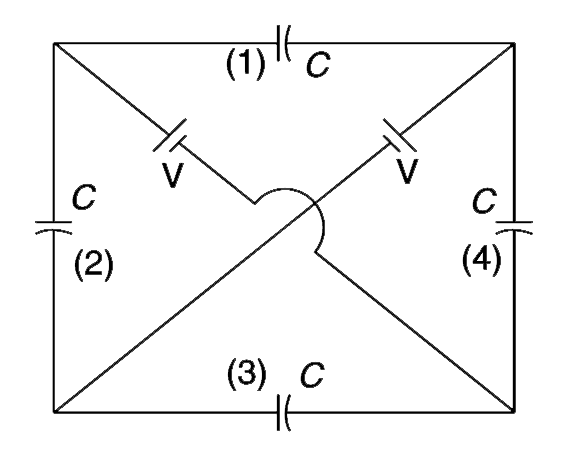
View SolutionIn the circuit shown in the figure, find charge on each capacitor.
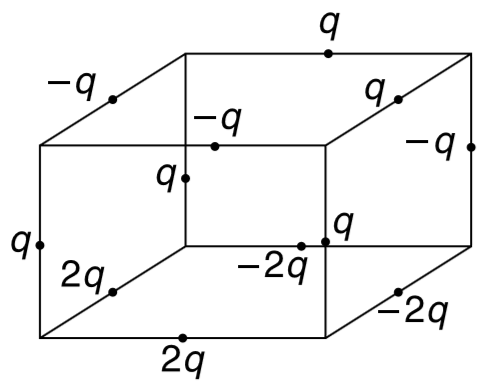
View SolutionTwelve charges have been placed at the centre of each side of a cube as shown in the figure. Find the magnitude of Electric force acting on a charge Q placed at the centre of the cube. Take the side length of the cube to be r.
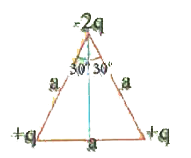
View SolutionThree points charges =q, -2q, +q are arranged on the vertices of an equilaterial triangle as shown in the figure. Find he dipole moment of the system.
View Solution