Four identical point charges are placed at the corners of a square, A fifth point charge placed at the center of the square experiences zero net force . Is this a stable equilirium for the fifth charge? Explain.
To determine whether the fifth charge placed at the center of the square formed by four identical point charges experiences stable equilibrium, we can analyze the forces acting on it and the potential energy associated with its position.
1. Understanding the Setup:
- We have four identical point charges placed at the corners of a square.
- A fifth charge is placed at the center of the square.
2. Analyzing Forces:
- Due to symmetry, the forces exerted by the four corner charges on the fifth charge will cancel out.
- Therefore, the net force acting on the fifth charge at the center is zero.
3. Definition of Stable Equilibrium:
- A position is considered a stable equilibrium if, when the charge is slightly displaced, it experiences a net force that acts to restore it to its original position.
- This is often associated with the potential energy being at a minimum at that point.
4. Displacing the Charge:
- If the fifth charge is displaced slightly in the plane of the square (up, down, left, or right), the forces from the corner charges will adjust.
- The two charges that are farther away will exert less force, while the two closer charges will exert more force, leading to a net force that pushes the charge back towards the center.
5. Potential Energy Consideration:
- As the charge moves away from the center, the potential energy of the system increases.
- Therefore, when displaced within the plane, the charge will experience a restoring force that brings it back to the center, indicating stable equilibrium.
6. Considering Out-of-Plane Displacement:
- If the charge is displaced vertically (above or below the plane of the square), the situation changes.
- The vertical components of the forces from the corner charges will not cancel out, leading to a net force that pushes the charge further away from the center.
- This indicates that there is no restoring force in the vertical direction.
7. Conclusion:
- The fifth charge experiences stable equilibrium when displaced in the plane of the square but not when displaced out of the plane.
- Therefore, the overall conclusion is that the equilibrium is not stable due to the lack of restoring force in the vertical direction.
- We have four identical point charges placed at the corners of a square.
- A fifth charge is placed at the center of the square.
- Due to symmetry, the forces exerted by the four corner charges on the fifth charge will cancel out.
- Therefore, the net force acting on the fifth charge at the center is zero.
- A position is considered a stable equilibrium if, when the charge is slightly displaced, it experiences a net force that acts to restore it to its original position.
- This is often associated with the potential energy being at a minimum at that point.
- If the fifth charge is displaced slightly in the plane of the square (up, down, left, or right), the forces from the corner charges will adjust.
- The two charges that are farther away will exert less force, while the two closer charges will exert more force, leading to a net force that pushes the charge back towards the center.
- As the charge moves away from the center, the potential energy of the system increases.
- Therefore, when displaced within the plane, the charge will experience a restoring force that brings it back to the center, indicating stable equilibrium.
- If the charge is displaced vertically (above or below the plane of the square), the situation changes.
- The vertical components of the forces from the corner charges will not cancel out, leading to a net force that pushes the charge further away from the center.
- This indicates that there is no restoring force in the vertical direction.
- The fifth charge experiences stable equilibrium when displaced in the plane of the square but not when displaced out of the plane.
- Therefore, the overall conclusion is that the equilibrium is not stable due to the lack of restoring force in the vertical direction.
Topper's Solved these Questions
- COULOMB LAW AND ELECTRIC FIELDBOOK - CENGAGE PHYSICSCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Solved examples
10 Videos
- COULOMB LAW AND ELECTRIC FIELDBOOK - CENGAGE PHYSICSCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Exercises
58 Videos
- COMMUNICATION SYSTEMBOOK - CENGAGE PHYSICSCHAPTER - COMMUNICATION SYSTEMEXERCISE - QUESTION BANK
19 Videos
- Current ElectricityBOOK - CENGAGE PHYSICSCHAPTER - Current ElectricityEXERCISE - QUESTION BANK
40 Videos
Similar Questions
.Knowledge Check
Four charges +Q,−Q,+Q,−Q are placed at the corners of a square taken in order. At the centre of the square
AE=0,V=0BE=0,V≠0CE≠0,V=0DE=0,V≠0Four charges are arranged at the corners of a square ABCD, as shown. The force on a positive charge kept at the centre of the square is
AzeroBalong diagonal ACCalong diagonal BDDperpendicular to the side ABFour point charge are placed at the corners of a square with diagonal 2a as shown. What is the total electric field at the center of the squre?
Akq/a2 at an angle 45∘ above the +x-axisBkq/a2 at an angle 45∘ above the -x-axisC3kq/a2 at an angle 45∘ above the -x-axisD3kq/a2 at an angle 45∘ above the +x-axis
A point charge Q is placed at the corner of a square of side a, then find the flux through the square.
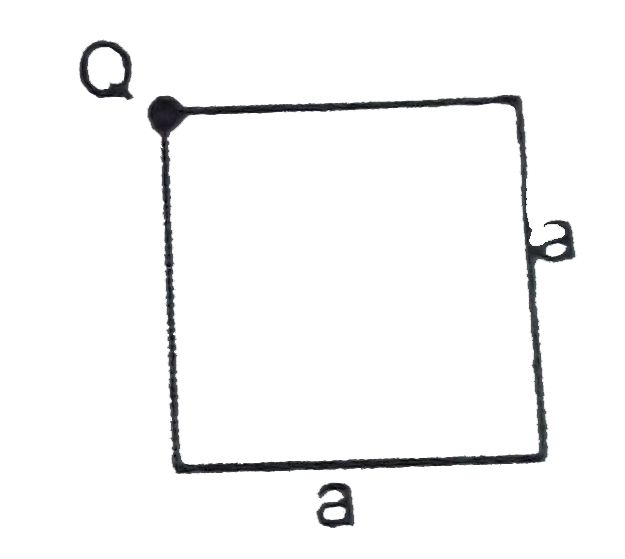
View SolutionA point charge Q is placed at the corner of a square of side a. Find the flux through the square.
View SolutionFour point charges are placed at the corners of a square ABCD of side 10 cm, as shown in figure. The force on a charge of 1μC placed at the centre of square is
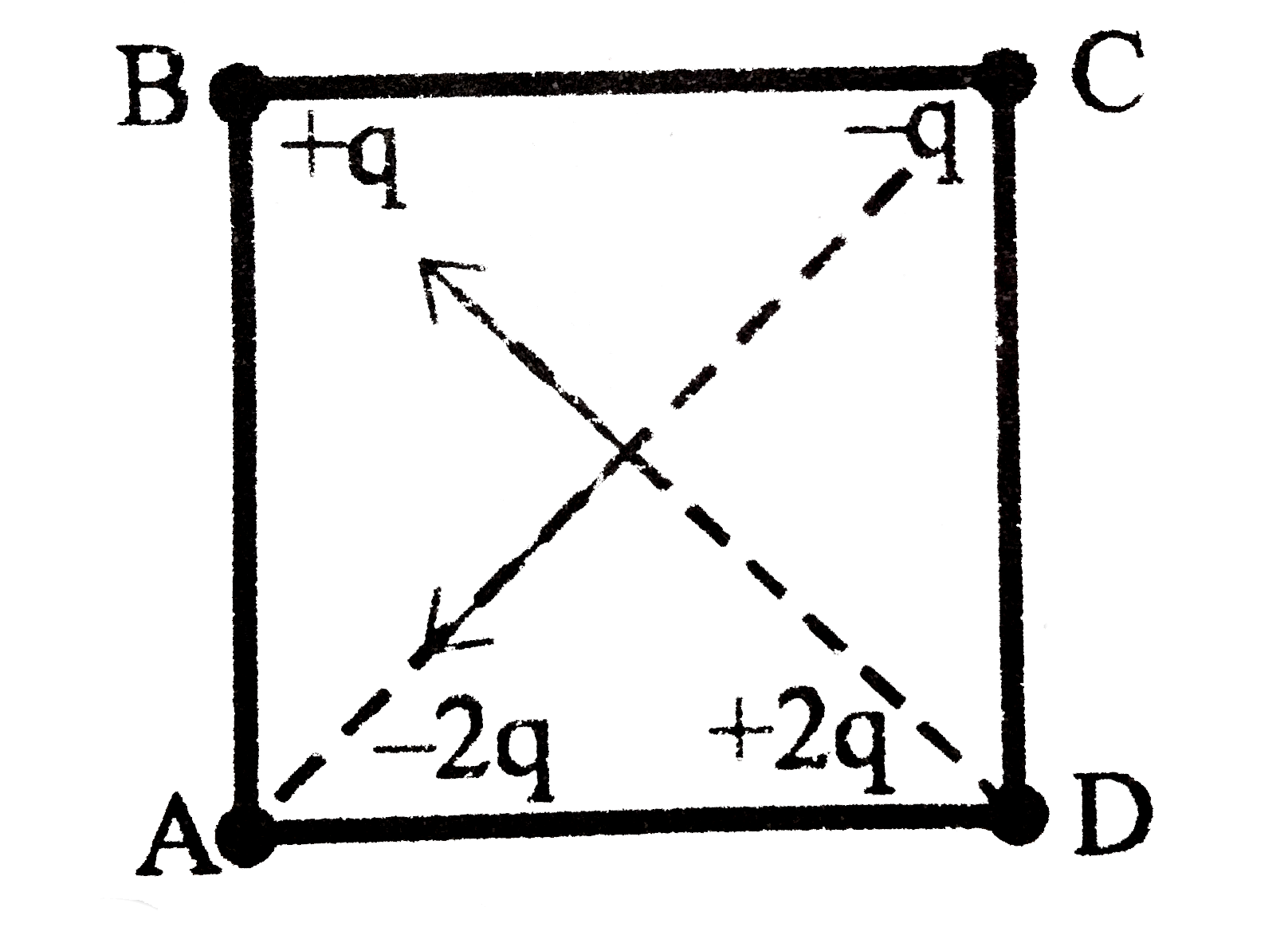
View SolutionFour charges are arranged at the corners of a square ABCD, as shown. The force on a =ve charge kept at the centre of the square is -
View SolutionFour identical charges i.e. q is placed at the corners of a square of side a. The charge Q that must be placed at the centre of the square such that the whole system of charges in equilibrium is
View Solution