Obtain an expression for the current through an inductor when an AC emf is applied. Solution in Telugu
Circuit consists of pure inductor of inductance L. Let an ac e.m.f V=Vm sin ωt is applied to it. Let I be the instantaneous current.
Back e.m.f deceloped across the inductor =−Ldidt
Total e.m.f =Vmsinωt−Ldidt ....(1)
According to ohms law this must be equal to iR = 0
Vmsinωt−Ldidt=0(∵R=0)
Ldidt=Vmsinωt
di=(VmL)sinωt dt
Integrating we get, ∫di=∫VmLsinωt dt
i=VmωLcosωt+k ....(2)
Where k is integration constant. Its value k = 0
i=−VmωLcosωt=VmωLsin(ωt−π2)
i=i0sin(ωt−π2) ....(3)
∴i0=VmωL
This is the expression for the instantaneous current through inductor. Here current lags behind the e.m.f by π2 radian (or) 90∘.
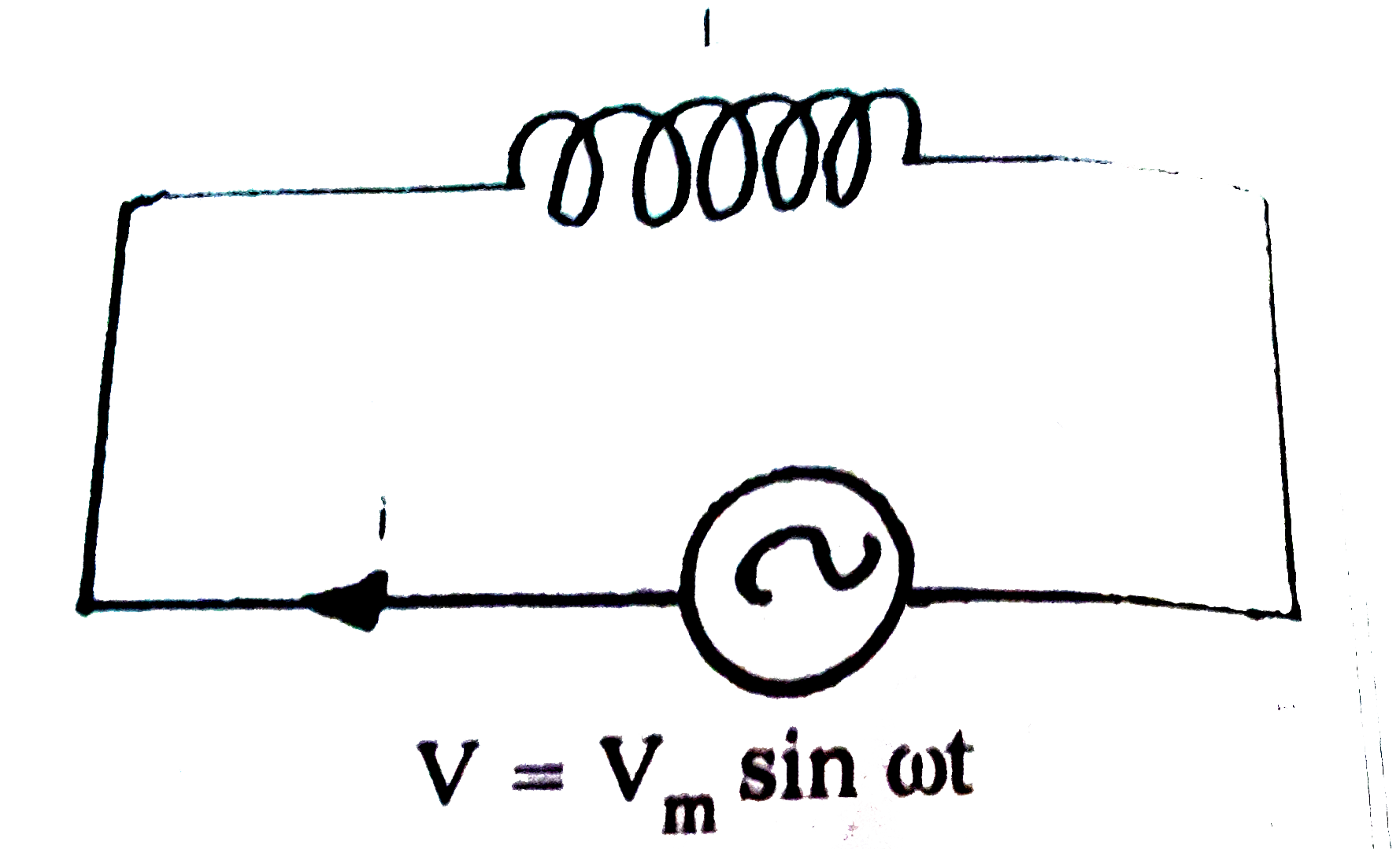
Topper's Solved these Questions
- ALTERNATING CURRENT Book:VIKRAM PUBLICATION ( ANDHRA PUBLICATION)Chapter:ALTERNATING CURRENT Exercise:LONG ANSWER QUESTIONS
Explore 1 Video
- ALTERNATING CURRENT Book:VIKRAM PUBLICATION ( ANDHRA PUBLICATION)Chapter:ALTERNATING CURRENT Exercise:TEXTUAL EXERCISES
Explore 15 Videos
- ALTERNATING CURRENT Book:VIKRAM PUBLICATION ( ANDHRA PUBLICATION)Chapter:ALTERNATING CURRENT Exercise:VERY SHORT ANSWER QUESTIONS
Explore 10 Videos
- ANDHRA PRADESH MARCH-2019Book:VIKRAM PUBLICATION ( ANDHRA PUBLICATION)Chapter:ANDHRA PRADESH MARCH-2019Exercise:SECTION-C
Explore 3 Videos
Similar Questions
Obtain on expression for impedance and current in series LCR circuit. Deduce an expression for the resonating frequency of an LCR series reasonating circuit.
View SolutionA 100Ω resistor is connected to a 220 V. 50 Hz ac supply.
What is the rms value of current in the circuit ?
View SolutionA transformer converts 200 V ac into 2000 V ac. Calculate the number of turns in the secondary if the primary has 10 turns.
View SolutionA steel wire 0.72 m long has a mass of 5.0×10_3kg.If the wire is under a tension of 60N , what is the speed of transverse waves on the wire?
View SolutionFor the circuit shown in Fig. the emf of the generator is E. The current through the inductor is 1.6 A. While the current through the condenser is 0.4 A. Then

View SolutionAn voltage, E=E0sinωt is applied acorss an inductor L. Obtain an expression for the current.
View SolutionObtain the expression for the magnetic energy stored in an ideal inductor of self inductance L when a current I passes through it.
Hence obtain the expression for the energy density of magentic field produced in the inductor.
View SolutionObtain an expression for the current through an inductor when an AC emf is applied.
View SolutionObtain the expression for current in case of AC applied to an inductor .
View SolutionAlternating Current | AC Voltage Applied To An Inductor
View SolutionObtain the expression for the magnetic energy stored in an ideal inductor of self inductance L when a current I passes through it. Hence obtain the expression for the energy density of magentic field produced in the inductor.
View SolutionAn a.c. voltage V=Vm sin ot is applied across an inductor L. Obtain an expression for current I.
View SolutionObtain an equation of current for AC voltage applied to an inductor and draw a graph of V and I.
View SolutionWhen an inductor coil is connected to an ideal battery of emf 10V, a constant current 2.5 A flows. When the same inductor coil is connected to an ac source of 10V and 50 HZ then the current is 2A. Find out the inductance of the coil.
View SolutionFor the circuit shown in Fig. the emf of the generator is E. The current through the inductor is 1.6 A. While the current through the condenser is 0.4 A. Then
View Solution