Deduce an expression for the force on a current carrying conductor placed in a magnetic field. Derive an expression for the force per unit length between two parallel current. Carrying conductors. Solution in Telugu

Video Solution

Text Solution

Verified by Experts

Expression for the Force acting ona current carrying conductor :
Consider a straight conductor (wire) of length 'l', area of cross section 'A', carrying a current 'I', which is placed ina uniform magnetic field of induction 'B' as shown in fig. We know the external magnetic field exerts a force on the conductor. The electrons in effect move with an average velocity called drift velocity $' V_{d}'$ . The direction of conventional current will be opposite to the direction of drift velocity. Let us assume that the current flows through the conductor from left 'B' in the plane of the paper makes an angle $' θ'$ with the direction of current 'i' as shown in fig.

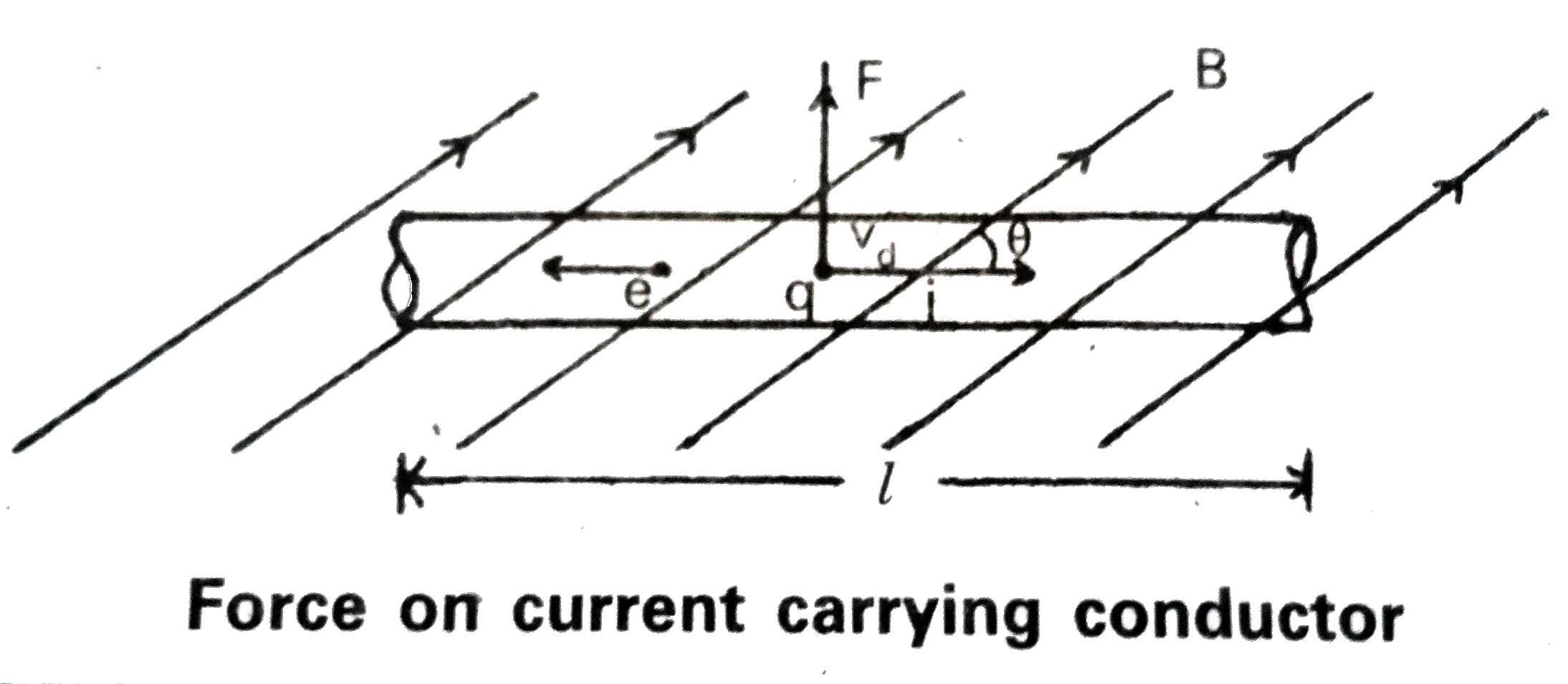
If F' is the force acting on the charge 'q' in B
$∴ F' = q V_{a} B sin θ$
If 'n' represents number of moving electrons per unit volume $(∵ n = \frac{N}{V})$
$∴ Current i = n q V_{d} A$ If 'N' is the number of electrons in the length 'l' N = nlA
Total force on conductor F= F'.N $(∵ N = n V = n \times A \times l)$
$= (q V_{d} B sin θ) (n l A)$
$= (n q V_{d} A) (l B sin θ)$
$∴ F = i l B sin θ$
Case (i) : If $θ = 0^{\circ}, F_{Min} = 0$
Case (ii) : If $θ = 90^{\circ}, F_{Max} = B i l$
Expression for the force between two Parallel conductors carrying conductors :
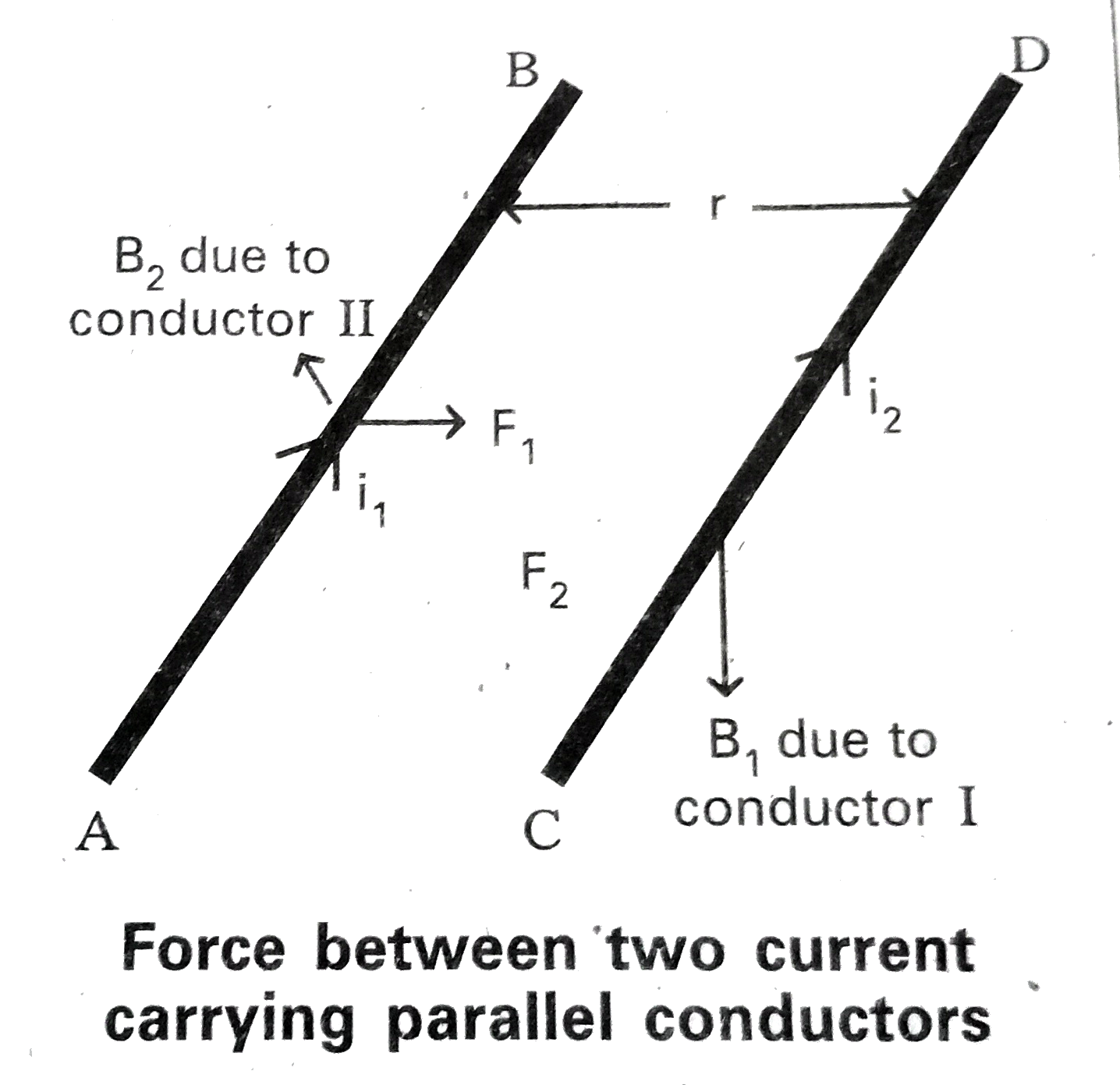
Consider two straight parallel conductors 'AB and 'CD' carrying currents $' i_{1} and' i_{2}$ and which are separated by a distance 'r' as shown in fig.
If $B_{1} and B_{2}$ are magnetic inductions produced by the current carrying conductors AB and CD. Magnetic induction $B_{1}$ at a distance 'r' from the conductor 'AB' can be written as $B_{1} = \frac{μ_{0} i_{1}}{2 π r}$
If 'F is force acting on 'CD' due to magnetic induction $' B_{1}'$ then $F_{C D} = i_{2} l B_{1}$ where l= length of the conductor
$F_{C D} = i_{2} l (\frac{μ_{0} i_{1}}{2 π r}) = \frac{μ_{0} i_{1} i_{2} l}{2 π r} - (1)$
The direction of the force can be determined by using Flemings left hand rule.
Similarly we can find the force acting on the conductor AB due to magnetic induction $B_{2}$ .
$F_{A B} = i_{1} l B_{2}$
$∴ F_{A B} = i_{1} l (\frac{μ_{0} i_{2}}{2 π r}) - (2) [∵ B_{2} = \frac{μ_{0} i_{2}}{2 π r}]$
From the equations (1) and (2) $F_{A B} = F_{C D} = \frac{μ_{0} i_{1} i_{2} l}{2 π r}$
$∴$ Force between two parallel, straight conductors carrying currents
$F = \frac{μ_{0} i_{1} i_{2} l}{2 π r}$
Force per unit length $\frac{F}{l} = \frac{μ_{0} i_{1} i_{2}}{2 π r}$

|

Updated on:21/07/2023

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Topper's Solved these Questions

Similar Questions

VIKRAM PUBLICATION ( ANDHRA PUBLICATION)-MOVING CHARGES AND MAGNETISHM-LONG ANSWER QUESTIONS

Home
Ask Doubt
Profile