Assume the radius of the earth to be 6.4×106m
a. Calculate the time period T of a satellite on equational orbit at 1.4×10em above the surface of the earth.
b. What is the speed of the satellite in this orbit?
c. If the satellite is travelling in the same direction as the rotation of the earth i.e. west to east, what is the interval between two successie times at which it will appear vertically overhead to an observed at a fixed point on the equator?
a. r=Rearth+h=6.4×106+1.4×106=7.8×106m
⇒r=7.8×106m⇒T=(4π2r3GMearth)=6831s
b. Speed of satellite
V=√GMearthr=7174ms−1
c. Relative angular velocity =ωsatellite−ωearth
Thus T=2πωs−ωe=2π[2πTs−2πTe]
⇒T=TsTeTe−Ts=7471s
Topper's Solved these Questions
- GRAVITATIONBook:CENGAGE PHYSICSChapter:GRAVITATIONExercise:Exercise 6.1
Explore 13 Videos
- GRAVITATIONBook:CENGAGE PHYSICSChapter:GRAVITATIONExercise:Exercise 6.2
Explore 15 Videos
- FLUID MECHANICSBook:CENGAGE PHYSICSChapter:FLUID MECHANICSExercise:INTEGER_TYPE
Explore 1 Video
- KINEMATICS-1Book:CENGAGE PHYSICSChapter:KINEMATICS-1Exercise:Integer
Explore 9 Videos
Similar Questions
If a satellite is travelling in the same direction as the rotation of earth i.e., west to east, what is the interval between two successive times at which it will appear vertically overhead to an observer at a fixed point on the equator? (R = 6400 km, h = 1400 km)
View SolutionAn artificial satellite is describing an eqatorial orbit at 1600 km above the surface of the earth. Calculate its orbital speed and the period of revolution. If the satellite is travelling in the same direction as the rotateion of the earth (i.e., from west to east), calculate the interval between two successive times at which it will appear vertically overhead to an observer at a fixed point on the equator. Radius of earth = 6400km.
View Solution
Knowledge Check
Assuming the radius of the earth to be 6.4×106 m calculate the time period T of a satellite for equatorial orbit at 1.4×103 km above the surface of the earth and the speed of the satellite in this orbit ?
A6831 and 7200 ms−1B6850 s and 7151 ms−1C68321 s and 7190 ms−1D6850 and 7400 ms−1A satellite having time period of revolution 6830 sec is travelling in the same direction as the rotation of the earth, i.e. from west to east, the time interval between the two successive times at which it will appear vertically overhead to an observer at a fixed point on the equator is
A86400 secB5000secC7416 secD84 secAssuming the radius of the earth to be 6.5×106 m. What is the time period T and speed of satellite for equatorial orbit at 1.4×103 km above the surface of the earth.
A6831sand7174 ms−1B34155sand3204 ms−1C6831sand2144 ms−1D2431sand3514ms−1
An artificial satellite is describing an equatorial orbit at 1600 km above the surface earth. Calculate its orbital speed and the period of revolution. If satellite is travelling in the same direction as the rotation of the earth (i.e., from west to east), calculate the interval between two successive time at wich it will appear verically overhead to an observer at a fixed point on the equator. Radius of earth = 6400 km.
View SolutionCalculate the period of revolution of a satellite orbiting above the surface of the Earth at a distance equal to 9 times the radius of the Earth.
View SolutionLet the time period of a satellite is 20 hours which is revolving in equatorial plane rotating in the direction of earth's rotation (i.e. west to east). Then the time interval between two consecutive overhead appearances observed by an observer at a fixed point on the Earth is 10x hours. What is the value of x?
View SolutionA geostationary satellite is orbiting the earth at a height of 6R above the surface of the earth, where R is the radius of the earth. What is the time period of another satellite orbiting at a height of 2.5 R from the surface of the earth ?
View SolutionMatch the Column I with Column II
For a satellite in circular orbit
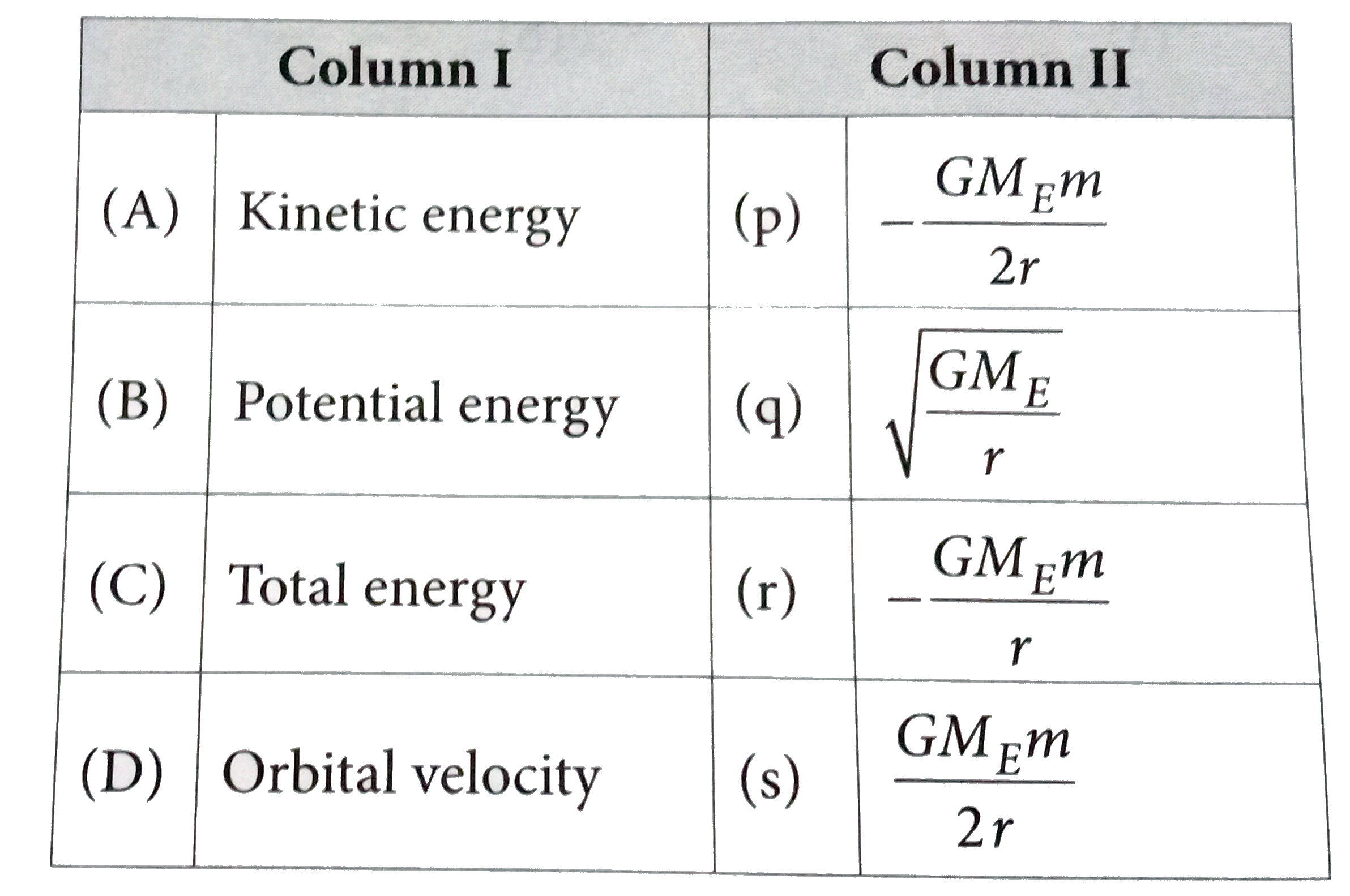
(where ME is the mass of the earth , m is the mass of the satellite and r is the radius of the orbit )
View Solution
