Find the relation between the gravitational field on the surface of tw

Two homogeneous spheres A and B of masses m and 2m having radii 2a and a respectively are placed in touch . The distance of the centre of mass from the first sphere is
Aa
B $2 a$
C $3 a$
Dnone of these
Two homogeneous spheres A and B of masses m and 2m having radii 2a and a respectively are placed in touch . The distance of the centre of mass from the first sphere is
Aa
B2a
C3a
DNone of these
The wires $A$ and $B$ shown in Fig. are made of the same material and have radii $r_{A}$ and $r_{B}$ , respectively. The block between them has a mass $m$ . When the force $F$ is $m g / 3$ , one of the wires breaks. Then

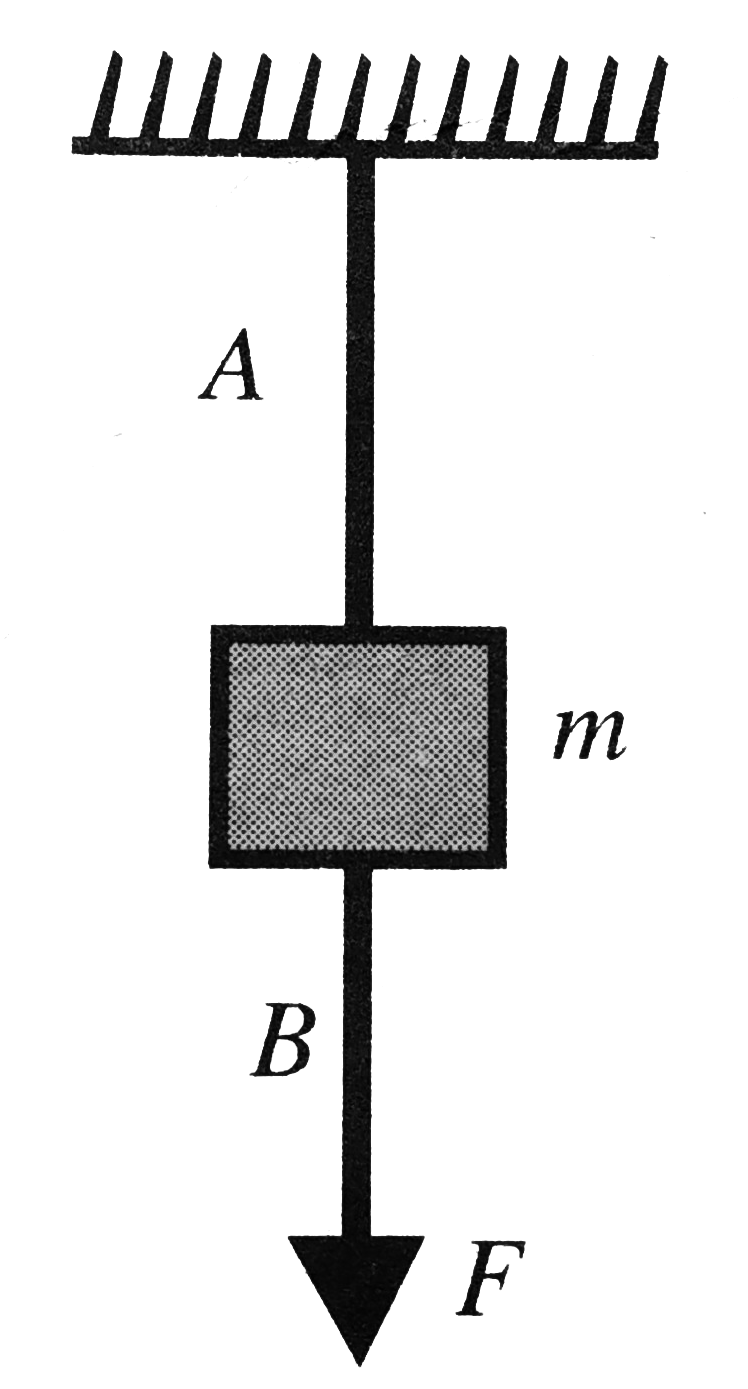A $A$ will break before $B$ if $r_{A} = r_{B}$
B $A$ will break before $B$ if $r_{A} < 2 r_{B}$
Ceither $A$ or $B$ may break if $r_{A} = 2 r_{B}$
Dthe lengths of $A$ and $B$ must be known to predict which wire will break.

Find the relation between the gravitational field on the surface of two planets $A$ and $B$ of masses $m_{A}, m_{B}$ and radii $R_{A}$ and $R_{B},$ respectively if
a. they have equal mass.
b. they have equal (uniform)density.