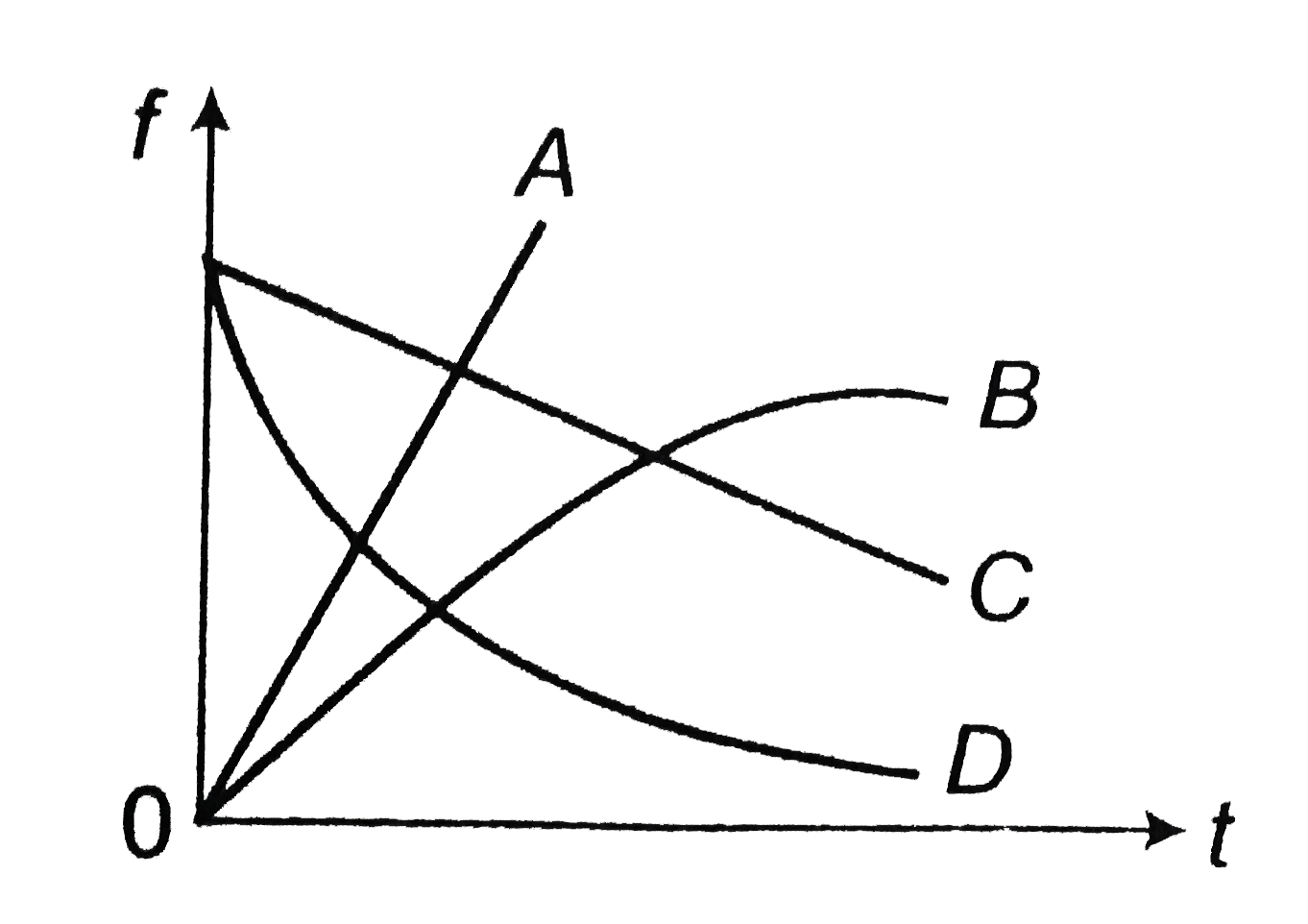
The fraction $f$ of radioactive material that has decayed in time $t$ , varies with time $t$ . The correct variation id given by the curve.
$The fraction f of radioactive material that has decayed in time t, varies with time t. The correct variation id given by the curve. .$ .

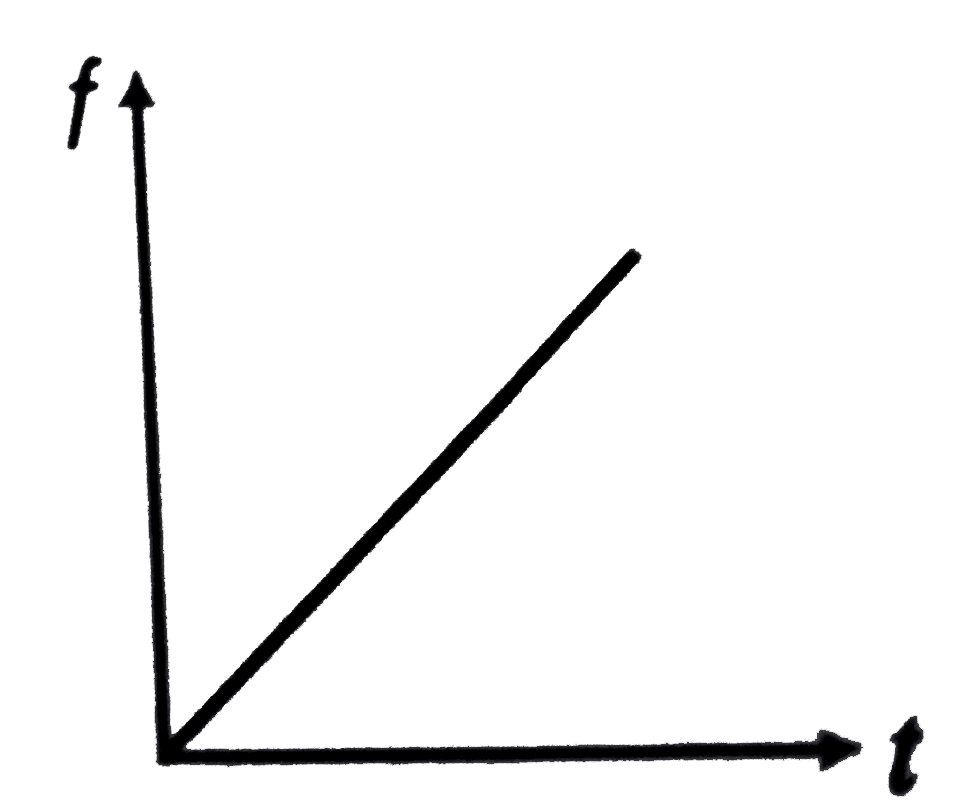
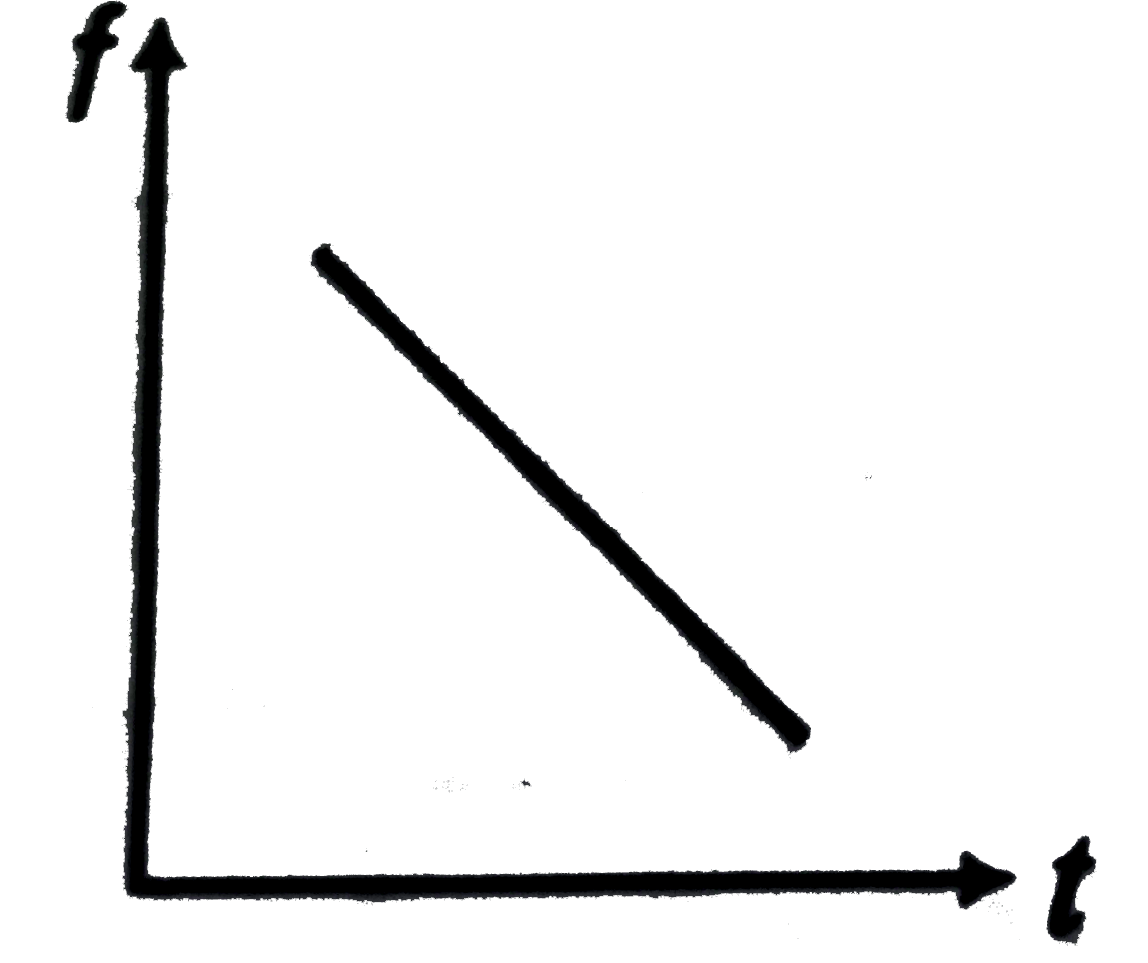
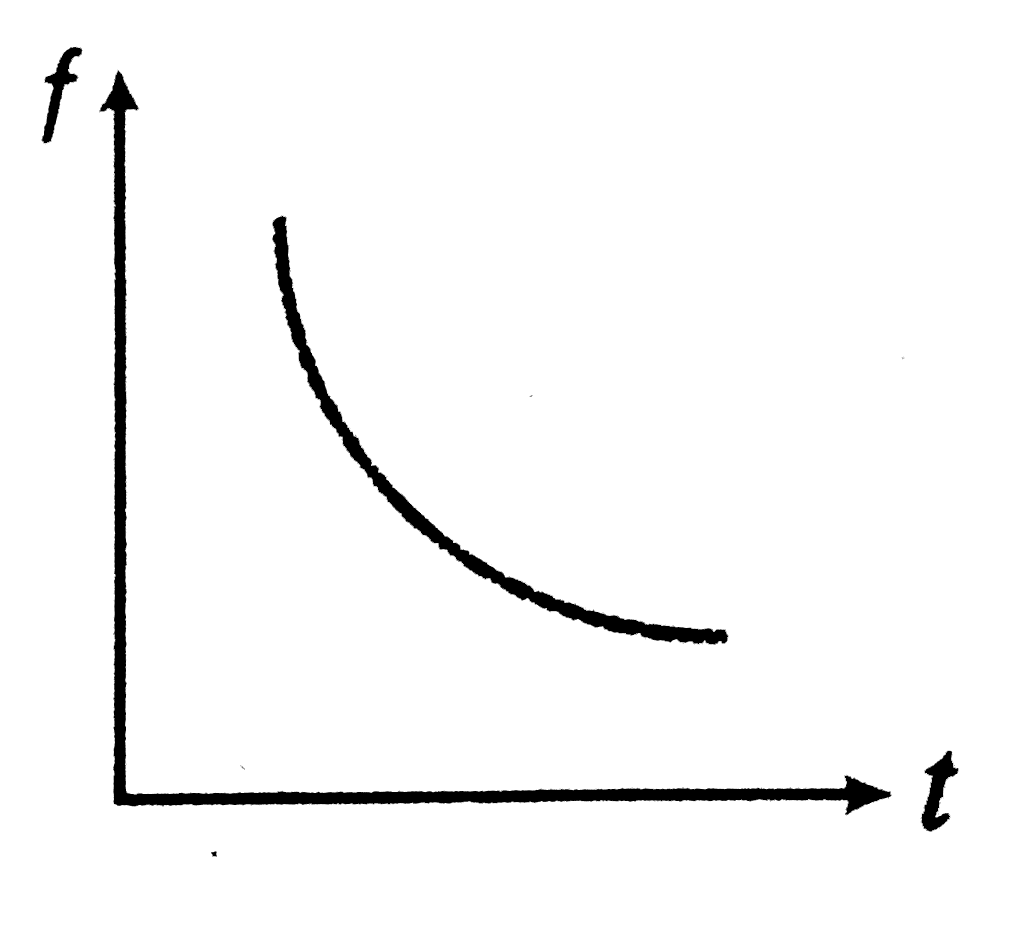
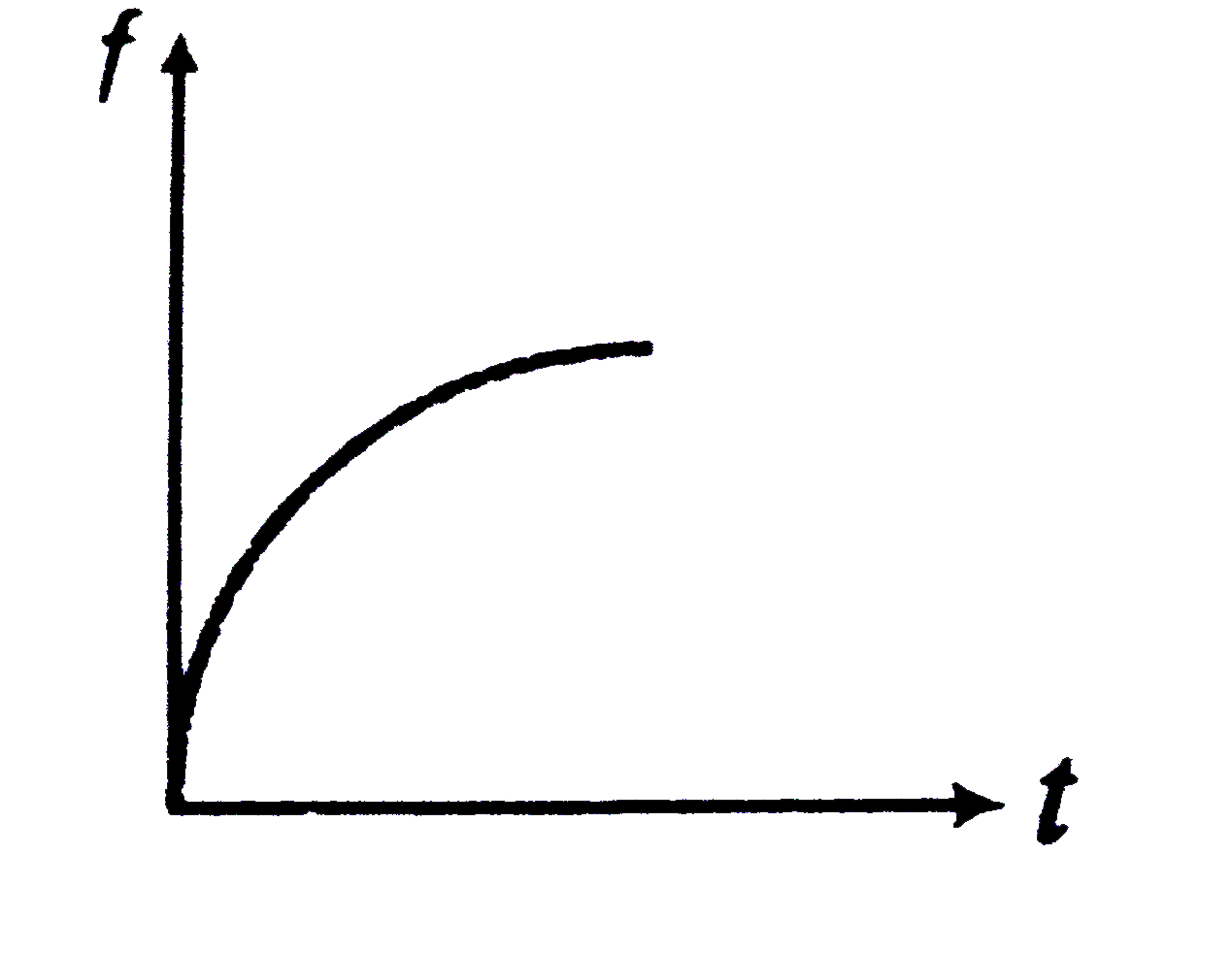
ocr_image

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Updated on:21/07/2023

Answer

Step by step video, text & image solution for The fraction f of radioactive material that has decayed in time t, varies with time t. The correct variation id given by the curve. . by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 PHYSICS MOCK TEST 5

Topper's Solved these Questions

Knowledge Check

The fraction of a radioactive material which remains active after time t is $9 / 16$ . The fraction which remains active after time $t / 2$ will be .
A $\frac{4}{5}$
B $\frac{7}{8}$
C $\frac{3}{5}$
D $\frac{3}{4}$
$20 %$ radioactive sample decay in time t. How much sample decay in time 2t ?
A $40 %$
B $36 %$
C $44 %$
D $80 %$
The equations $\frac{d N}{d t} = - λ N$ and $N = N_{0} e^{- λ t}$ describes how the number N of undecayed atoms in a sample of radioactive material, which initially (at $t = 0$ ) contained $N_{0}$ undecayed atoms, varies with time t. Which one of the following statements about $λ$ is correct ?
A $λ δ t$ gives the fraction of atoms present which will decay in the next small time interval $δ t$
B $λ$ is the time needed for N to fall from N0 to the value $N_{0} / e$
C $λ$ is the number of atoms left after a time equal to e second.
D $λ$ is the chance that any one atom will still be undecayed after one second.

MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERS-MOCK TEST 5-MCQs

Home
Ask Doubt
Profile

The fraction f of radioactive material that has decayed in time t, varies with time t. The correct variation id given by the curve. .

The correct Answer is:C

Topper's Solved these Questions

Similar Questions

Knowledge Check

The fraction of a radioactive material which remains active after time t is 9/16. The fraction which remains active after time t/2 will be .

20% radioactive sample decay in time t. How much sample decay in time 2t ?

The equations dNdt=−λN and N=N0e−λt describes how the number N of undecayed atoms in a sample of radioactive material, which initially (at t=0) contained N0 undecayed atoms, varies with time t. Which one of the following statements about λ is correct ?

MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERS-MOCK TEST 5-MCQs

The fraction $f$ of radioactive material that has decayed in time $t$ , varies with time $t$ . The correct variation id given by the curve.
$The fraction f of radioactive material that has decayed in time t, varies with time t. The correct variation id given by the curve. .$ .

The fraction of a radioactive material which remains active after time t is $9 / 16$ . The fraction which remains active after time $t / 2$ will be .

$20 %$ radioactive sample decay in time t. How much sample decay in time 2t ?

The equations $\frac{d N}{d t} = - λ N$ and $N = N_{0} e^{- λ t}$ describes how the number N of undecayed atoms in a sample of radioactive material, which initially (at $t = 0$ ) contained $N_{0}$ undecayed atoms, varies with time t. Which one of the following statements about $λ$ is correct ?