A particle of mass m is kept on a fixed , smooth sphere of radius R at a position, where the radius through the particle makes an angle of 300 with the vertical. The particle is releases from this position. a. What is the force exerted by the sphere on the particle just after the release? b. Find the distance travelled by the particle before it leaves contact with the sphere.

a. When the particle is released from rest, the centripetal force is zero.
N=mgcosθ
=mgcos30∘
=√32mg
b. When the particle leaves contact with surface N=0,
mv2R=mgcosθ
v2=Rgcosθ (i)
(12)mv2=mgR(cos30∘−cosθ)
v2=2Rg(√32−cosθ) (ii)
From Eqs. (i) and (ii),

Rgcosθ=2Rg[√32−cosθ]
3cosθ=√3
cosθ=1√3⇒θ=cos−11√3
So the distance travelled the particle before leaving contact.
L=R(θ−π6) [because 30∘=π/6]
Topper's Solved these Questions
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Solved Examples
15 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Exercise 8.1
25 Videos
- VECTORSBOOK - CENGAGE PHYSICSCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
A particle of mass m is kept on a fixed , smooth sphere of radius R at a position, where the rdius thrugh the particle makes an angle of 300 with the vertical. The particle is releases from this positon. a. What is the force exerted by the sphere on teh particle just after the release? b. Find the distance travelled by the particle before it leaves contact with the sphere.
View SolutionA small box of mass m is kept on a fixed, smooth sphere of radius R at a position where the radius through the box makes an angle of 30∘ with the vertical. The box is released from this position
(a) What is the force exerted by the sphere on the box just after the release ?
(b) Find the distance travelled by the box before it leaves contact with the sphere.
View Solution
Knowledge Check
A particle of mass m is kept at the top of a smooth fixed sphere. It is given a horizontal velocity v then:
Ait will start moving along a circular path if v<√gRBit will start moving along a circular path if v>√gRCit will start moving along a parabolic path if v<√gRDit will start moving along a parabolic path if v>√gRA particle is released from the top of the smooth hemisphere R as shown.
the normal contact between the particle and the hemisphere in position θ is
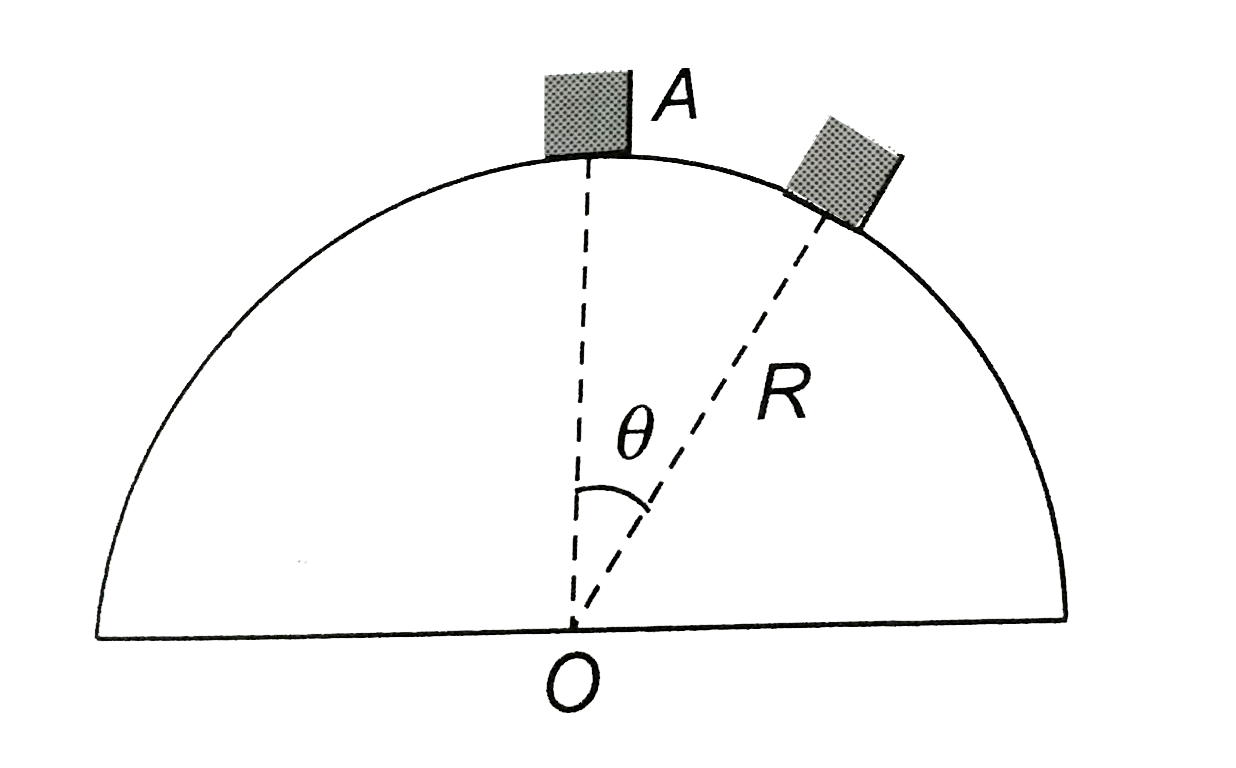 Amg(3−2cosθ)Bmg(3cosθ−2)Cmg(4cosθ−3)Dmg(4−3cosθ)
Amg(3−2cosθ)Bmg(3cosθ−2)Cmg(4cosθ−3)Dmg(4−3cosθ)In the figure shown, a particle is released from the position A on a smooth track. When the particle reaches at B, then normal reaction on it by the track. When the particle reaches at B, then normal reaction on it by the track is
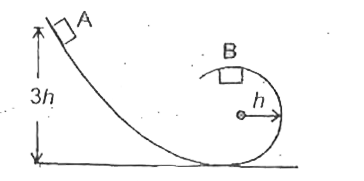 AmgB2mgC23mgDm2gh
AmgB2mgC23mgDm2gh
A particle of mass m is released on a fixed. Smooth sphere of radius R at a position, where the radius through the particle makes an angle of α with the verticle. What is the angle made by radius through the particle when the particle loses contact with sphere? (cosα=3/4)
View SolutionA small box of mass m is placed on the outer surface of a smooth fixed sphere of radius R at a point where the radius makes a angle Pse with the vertical. The box is released from this position. Find the distance travelled by the box before it leaves contact with the sphere.
View SolutionA particle slides on the surface of a fixed smooth sphere starting from the topmost pont. Find the angle rotated by the radius through the particle, when it leaves contact with the sphere.
View SolutionA particle of mass m is released from the top of a smooth hemisphere of radius R with the horizontal speed μ. Calculate the angle with verticle where it loses contact with the hemisphere.
View SolutionA block of mass m is placed on a smooth sphere of radius R. It slides when pushed slightly. At what vertical distance h, from the top, will it leave the sphere?
View Solution
