A small block is connected to one of two identical massless strings of lengtth 1623cm each with their ends fixed to a verticle rod If the ratio of tensions T1/T2 is 4 : 1 ,then what will be the angular velocity of the block ? Take g=9.8ms−2
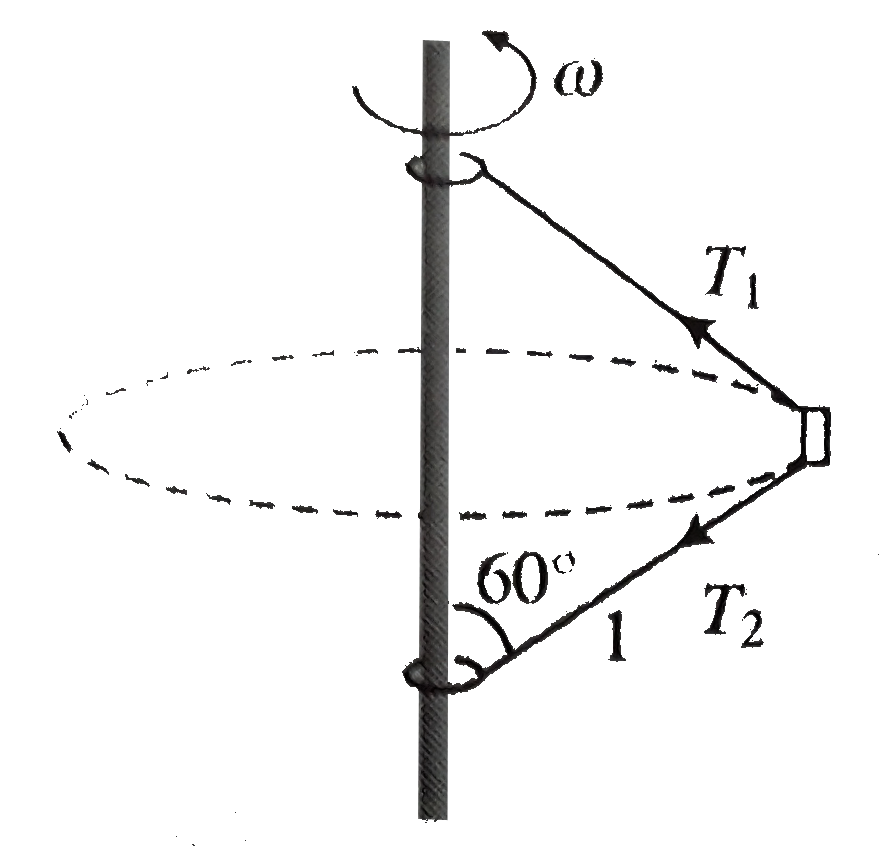
For horizontal,
T1sin60∘+T2sin60∘=mω2r=mω21sin60∘
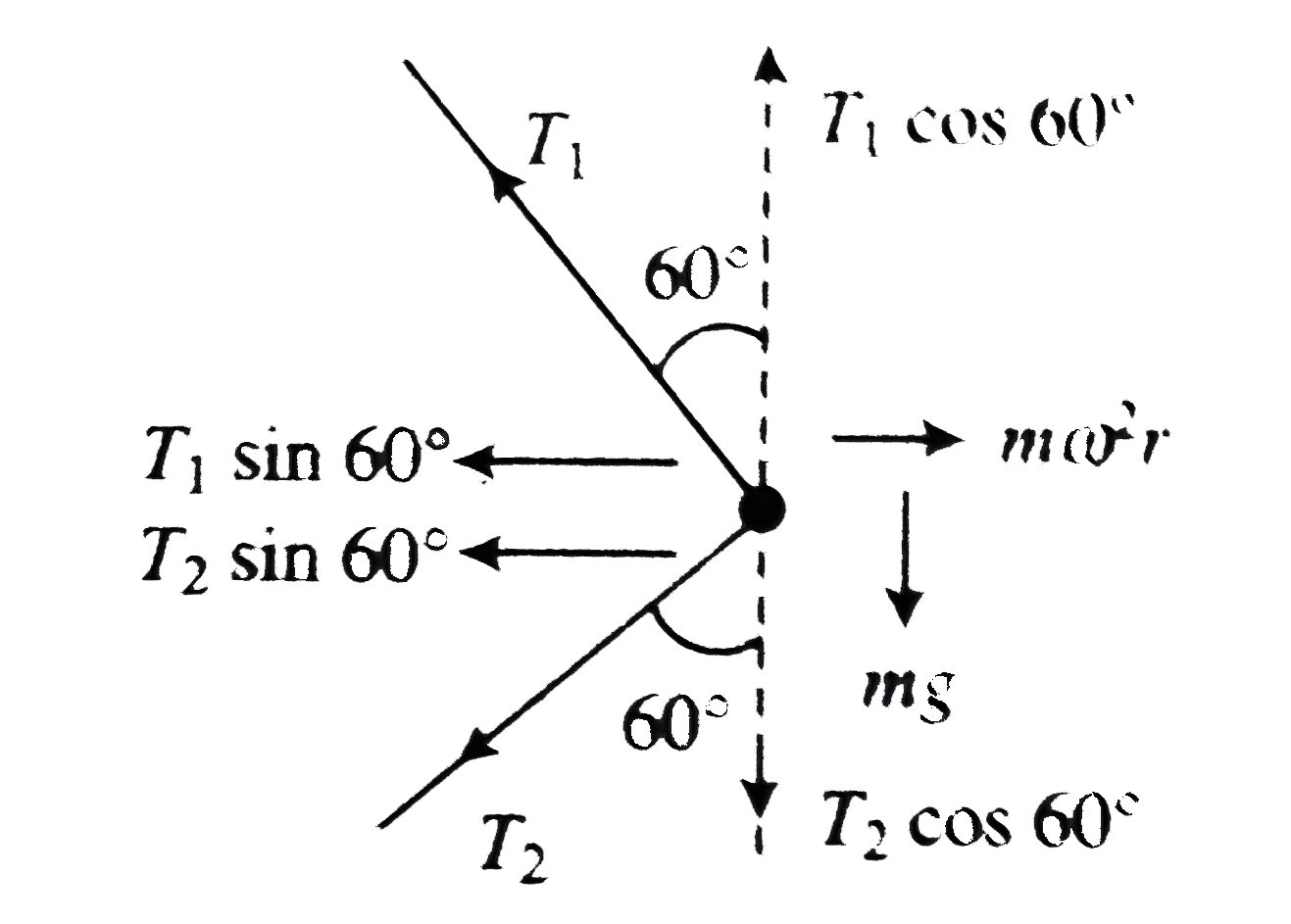
⇒T1+T2=mω21…(i)
For verticle equlibrium of the block
T1cos=T2cos60∘+mg
⇒T1−T2=mgcos60∘=2mg .....(ii)
Dividing (i) by (ii), T1+T2T1−T2=ω212g
⇒T1T2+1T1T2−1=ω212g⇒4+14−1=ω212g
or ω2=10g3l=10×9.8×33×50×10−2=196
or ω=14rads−1
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Solved Examples
12 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Exercise 7.1
25 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer
5 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICSCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
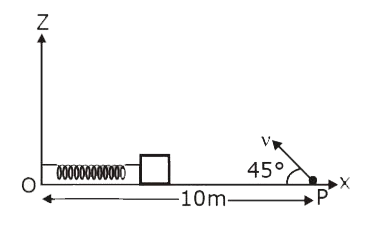
A small block is connected to one end of a massless spring of un-stretched length 4.9 m. The other end of the spring (see the figure) is fixed. The system lies on a horizontal frictionless surface. The block is stretched by 0.2 m and released from rest at t = 0. It then executes simple harmonic motion with angular frequency ω=π3rad/s . Simultaneously at t = 0, a small pebble is projected with speed ν from point P at an angle of 45∘ as shown in the figure. Point P is at a horizontal distance of 10 m from O. If the pebble hits the block at t = 1s, the value of ν is (take g = 10 m/s2 )
View SolutionA cubical block of mass 2 kg with each side of 2 cm is lying on the ground. What will be the pressure exerted by the block on the ground?
(Take g=9.8m/s2)
View Solution
Knowledge Check
A pendulum consisting of a small sphere of mass m, suspended by a inextensible and massless string of length 1 , is made to swing in a verticle plane. If the breaking strength of the string is 2 mg, then the maximum angular amplitude of the displacement from the verticle can be
A90∘B60∘C30∘D0∘A uniform rod of mass m and length L is suspended with two massless strings as shown in figure. If the rod is at rest in a horizontal position the ratio of tension in the two strings T1/T2 is
 A1:1B1:2C2:1D4:3
A1:1B1:2C2:1D4:3A uniform rod of mass m and length L is suspended with two massless strings as shown in the figure. If the rod is at rest in a horizontal position the ratio of tension in the two strings T1T2 is:
 A1:1B1:2C2:1D4:3
A1:1B1:2C2:1D4:3
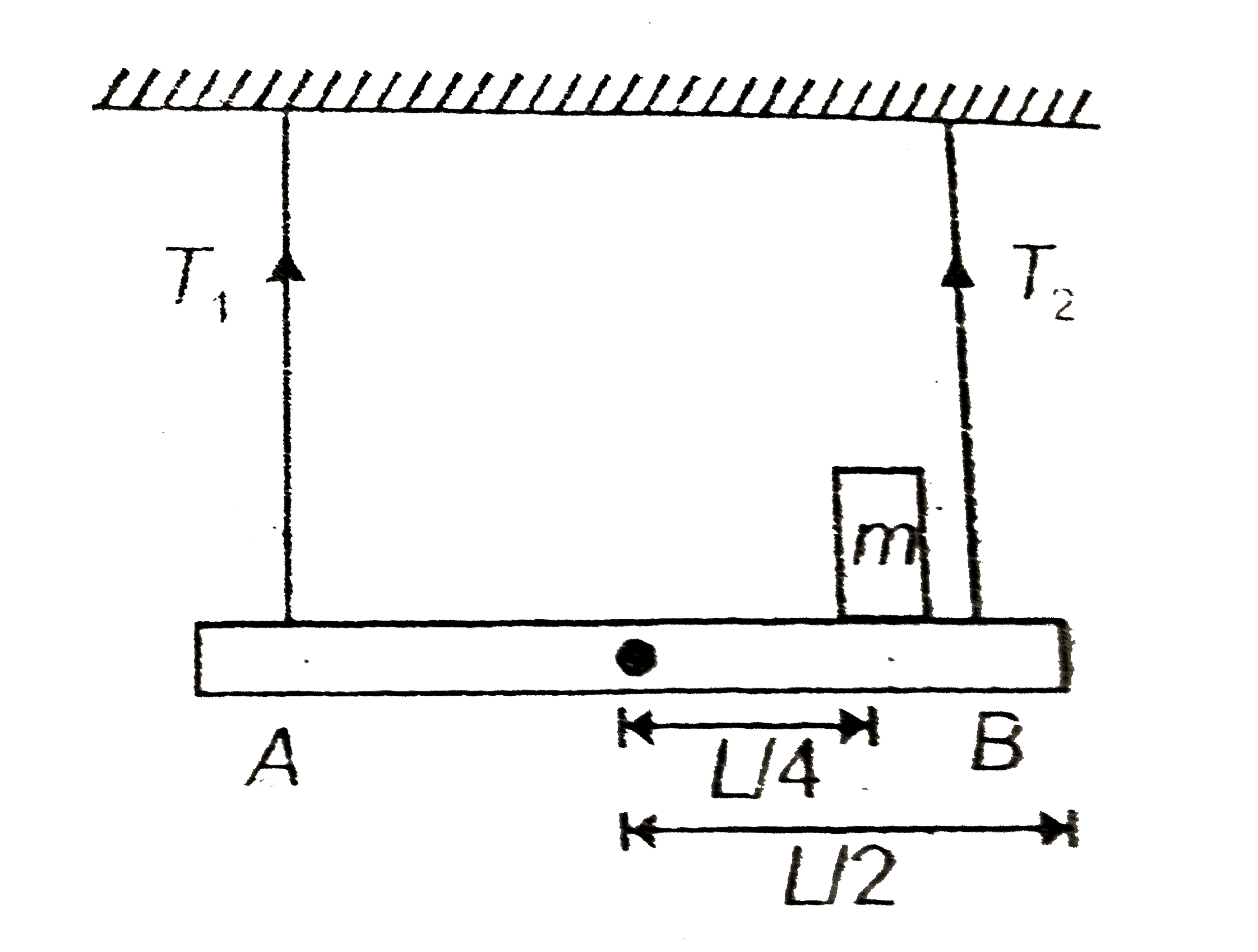
A rod AB of mass m is hung by two ideal threads. Find the ratio of tensions in the two threads (T1T2).
View SolutionTwo particles each of mass m are moving in horizontal circle with same angular speed. If both string are of same length then the ratio of tension in string T1T2 is
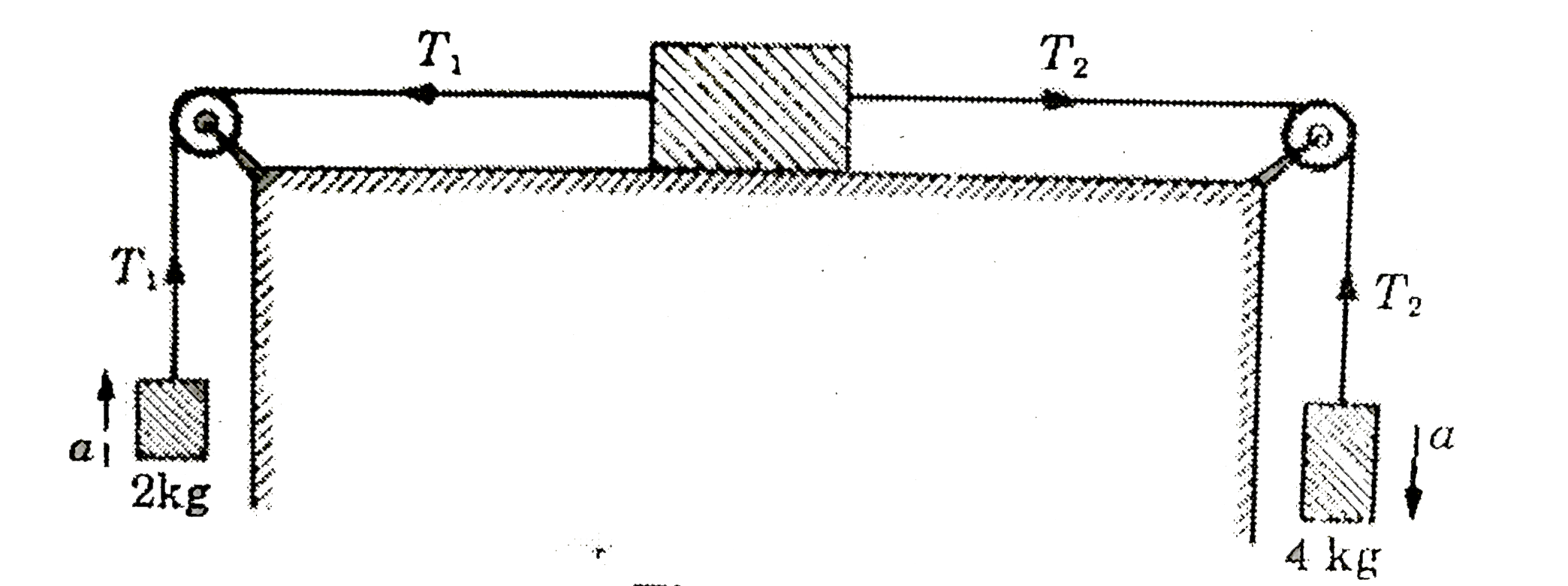
View SolutionIn Fig. find the acceleration a of the system and the tesnions T1 and T2 in the strings. Assume that the table and the pulleys are frictionless and the strings are massless. Take g = 9.8ms2
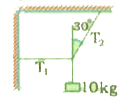
View SolutionA block of mass 10 kg is suspended with two strings as shown in the fig. Find the tensions T1andT2 in the two string.
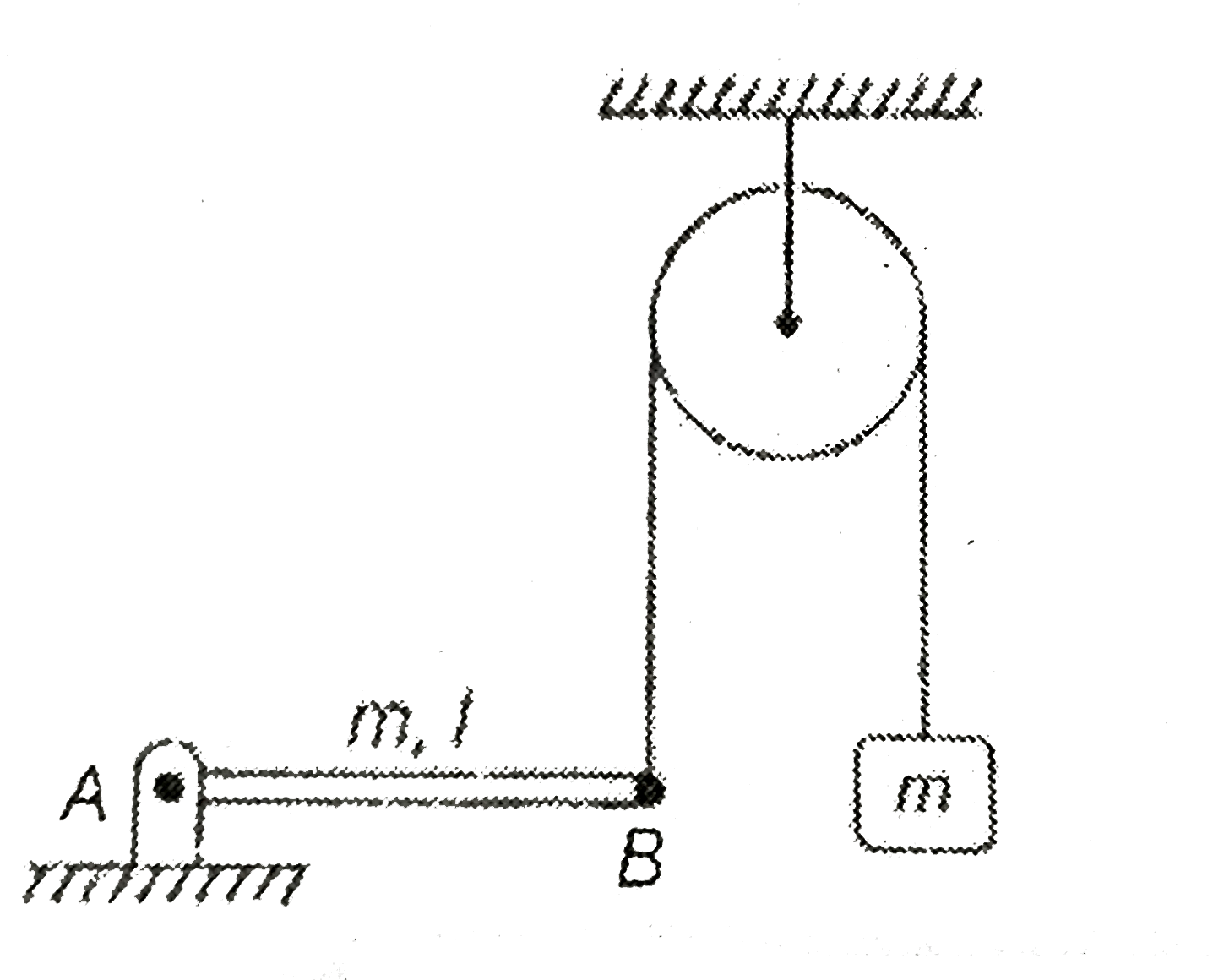
View SolutionUniform rod Ab is hinged at the end A in the figure. The other end of the rod is connected toa block through a massless string as shown. The pulley is smooth and massless. Masses of the block and the rod are same and are equal to 'm'. Acceleration due to gravity is g. The tension in the thread and angular acceleration of the rod just after releases of block from this position are
View Solution
