A particle moves on a circle of radius $r$ with centripetal acceleration as function of time as $a_{c} = k^{2} r t^{2}$ , where $k$ is a positive constant. Find the following quantities as function of time at an instant :
(a) The speed of the particle
(b) The tangential acceleration of the particle
( c) The resultant acceleration, and
(d) Angle made by the resultant with tangential direction.

Video Solution

Text Solution

Verified by Experts

(a) In the problem, it is given that $a_{c} = k^{2} r t^{2}$
Since $a_{c} = \frac{v^{2}}{r} \Rightarrow \frac{v^{2}}{r} = k^{2} r t^{2}$
$\Rightarrow v^{2} = k^{2} r^{2} t^{2}$
Taking square root on both sides, we get $v = k r t$
(b) Tangential acceleration,
$a_{t} = \frac{d v}{d t} = \frac{d}{d t} (k r t) = k r$
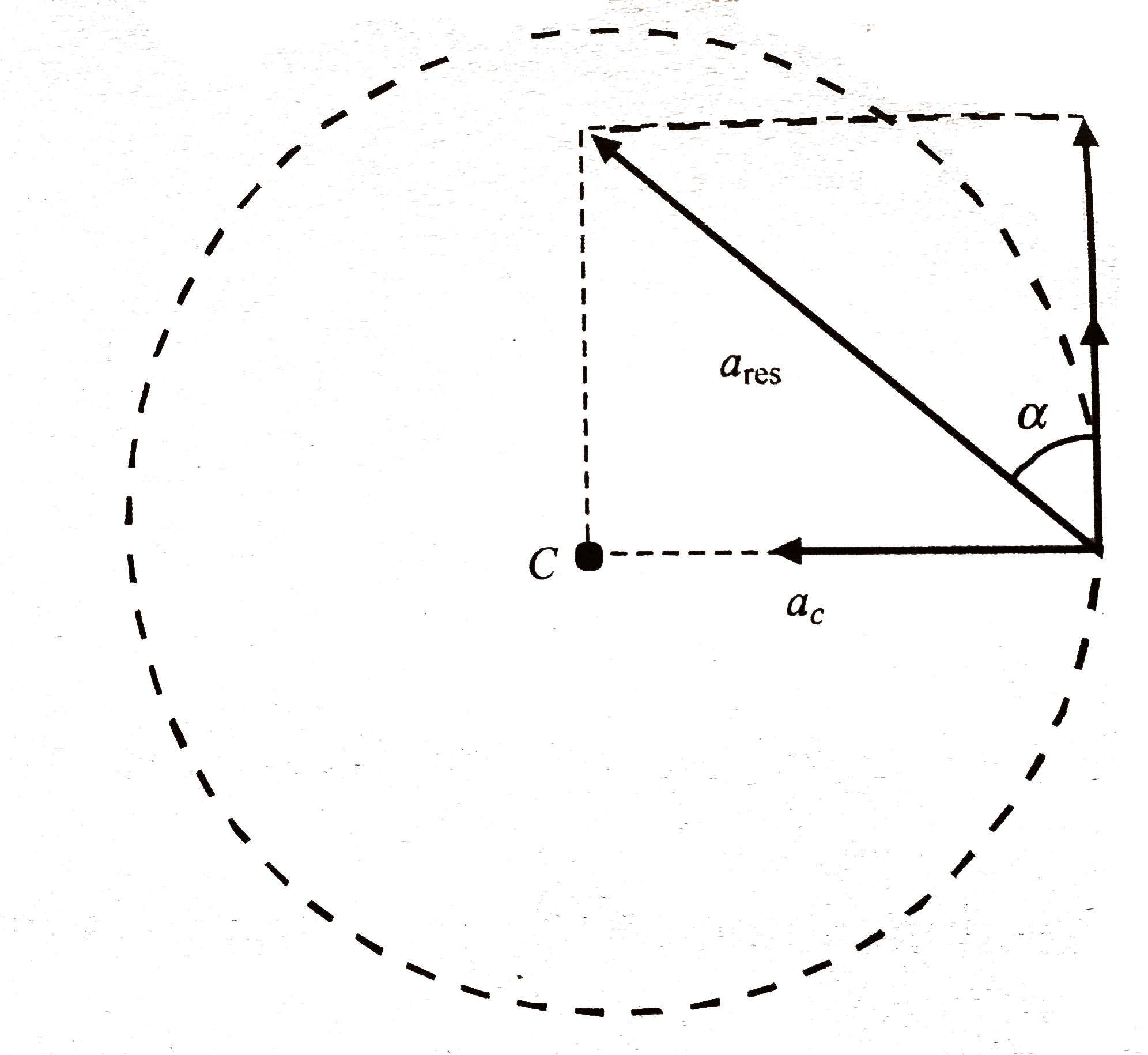
( c) Resultant acceleration,
$a_{r e s} = \sqrt{a_{c}^{2} + a_{t}^{2}}$
= $\sqrt{{(k^{2} r t^{2})}^{2} + {(k r)}^{2}}$
= $k r \sqrt{k^{2} t^{4} + 1}$ .
(d) From (Fig. 148), $tan \propto = \frac{a_{c}}{a_{t}} = \frac{k^{2} r t^{2}}{k r} = k t^{2}$
$\Rightarrow \propto = {tan}^{- 1} (k t^{2})$ .
.

Updated on:21/07/2023