At t=0, a particle starts from reat and moves along a straight line, whose acceleration-time graph is shown in .
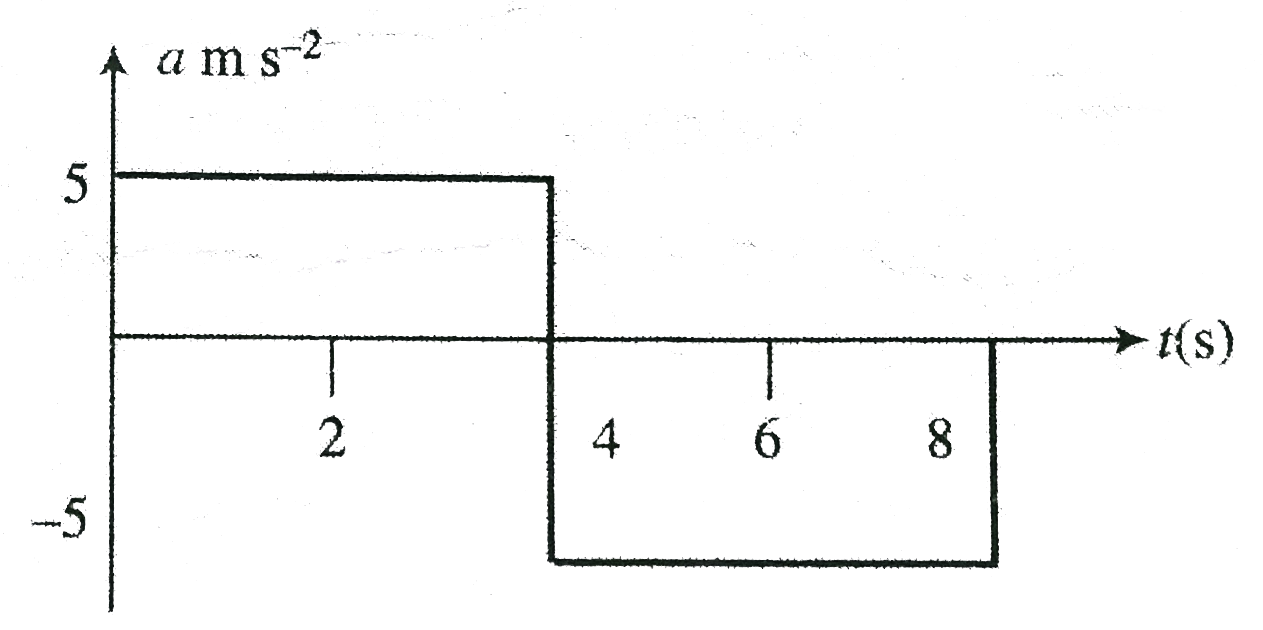 .
.
Convert this graph into velocity-time, From the velocity-time graph, find the maximum velocity attained by the particle. Also find from v−t graph, the sisplacement and distance travelled by the particle from 2 to 6s,
From 04s, at t=0,a=5ms−2
 .
.
From 4 to 8 s, a=−5ms−2
v2=v1+∗
⇒v2−5×4=0ms−1
Maximum velocity is 20ms−1
at t=4s.
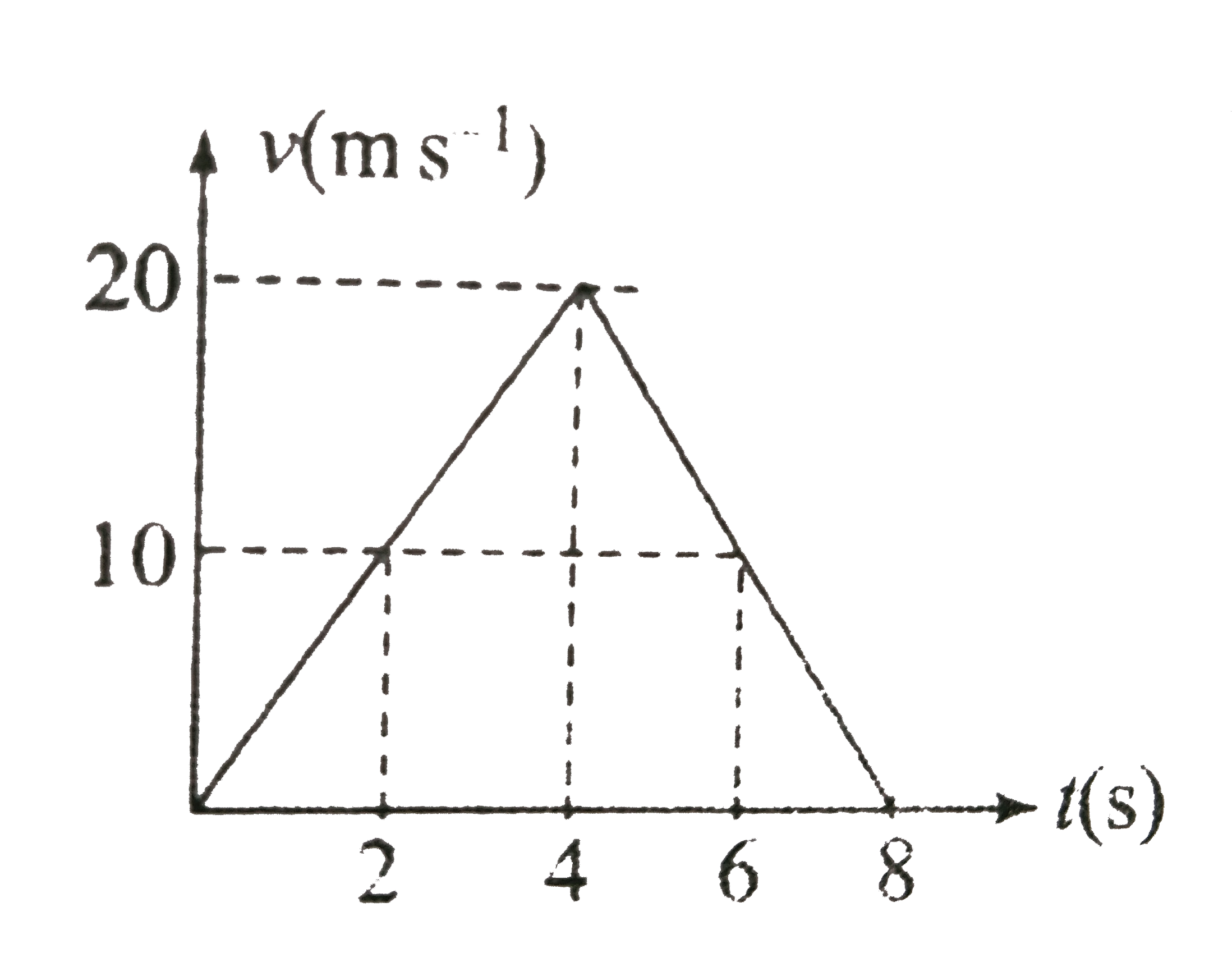 .
.
Desplacement form 2 to 6s is equal to area from2 to 6s, i.e.
10×4+(1/2)×4×10=60m
Since the motiontakes place along the same direction only
distance=displacement=60m.
Topper's Solved these Questions
- KINEMATICS-1BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Subjective
29 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Single Correct
52 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Exercise 4.3
15 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Video
- KINEMATICS-2BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise Integer
9 Videos
Similar Questions
A particle starts motion from rest and moves along a straight line. Its acceleration-time graph is shown. Find out speed of particle at t=2s and at t=3s.

View SolutionA particle starts motion from rest and moves along a straight line. Its acceleration-time graph is shown. Find out speed of particle at t=2s and at t=3s.

View Solution
Knowledge Check
A particle starts to move along a straight line. The acceleration versus time graph of particle is as shown in figure. The correct velocity versus time graph is :
 A
A B
B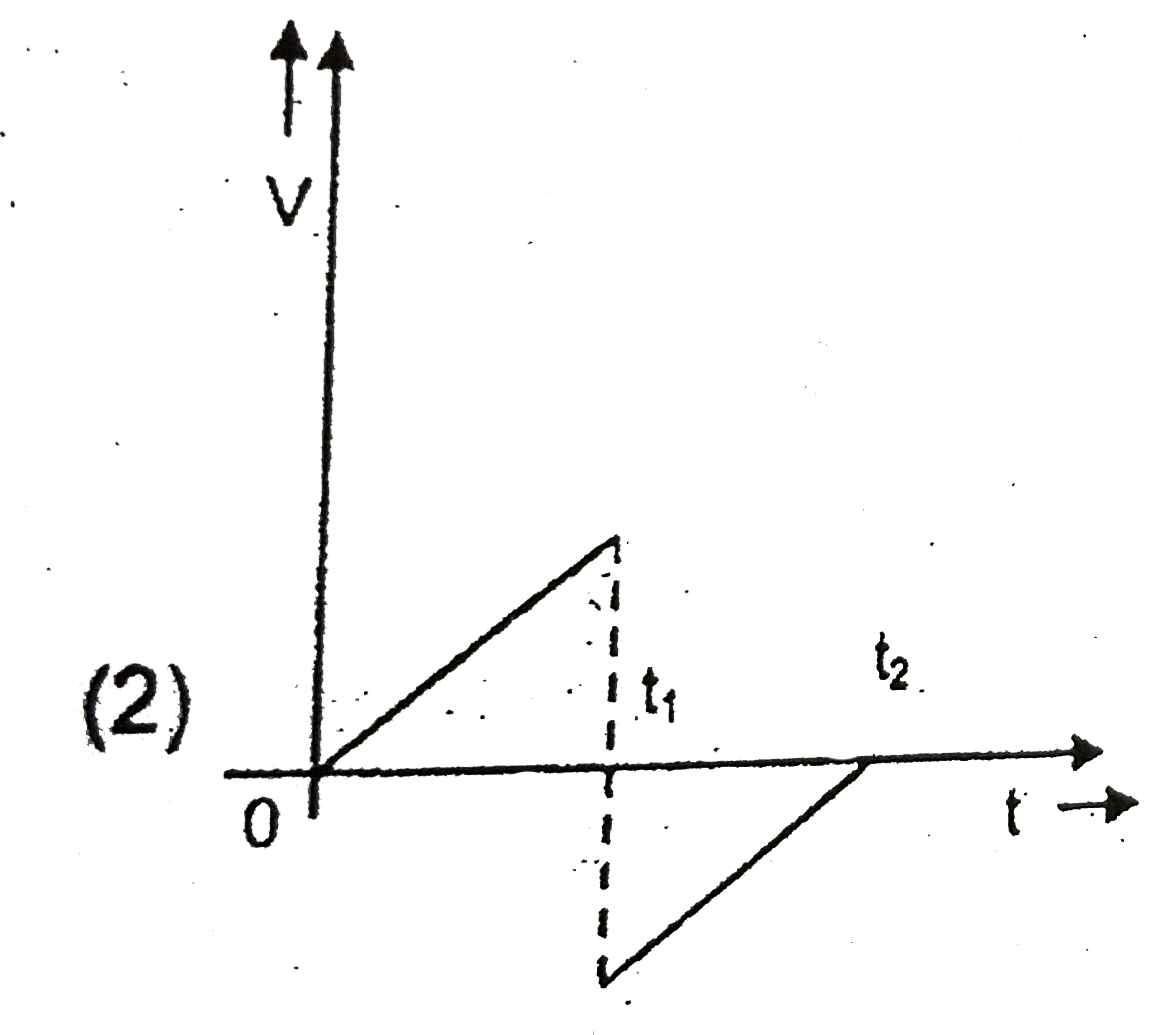 C
C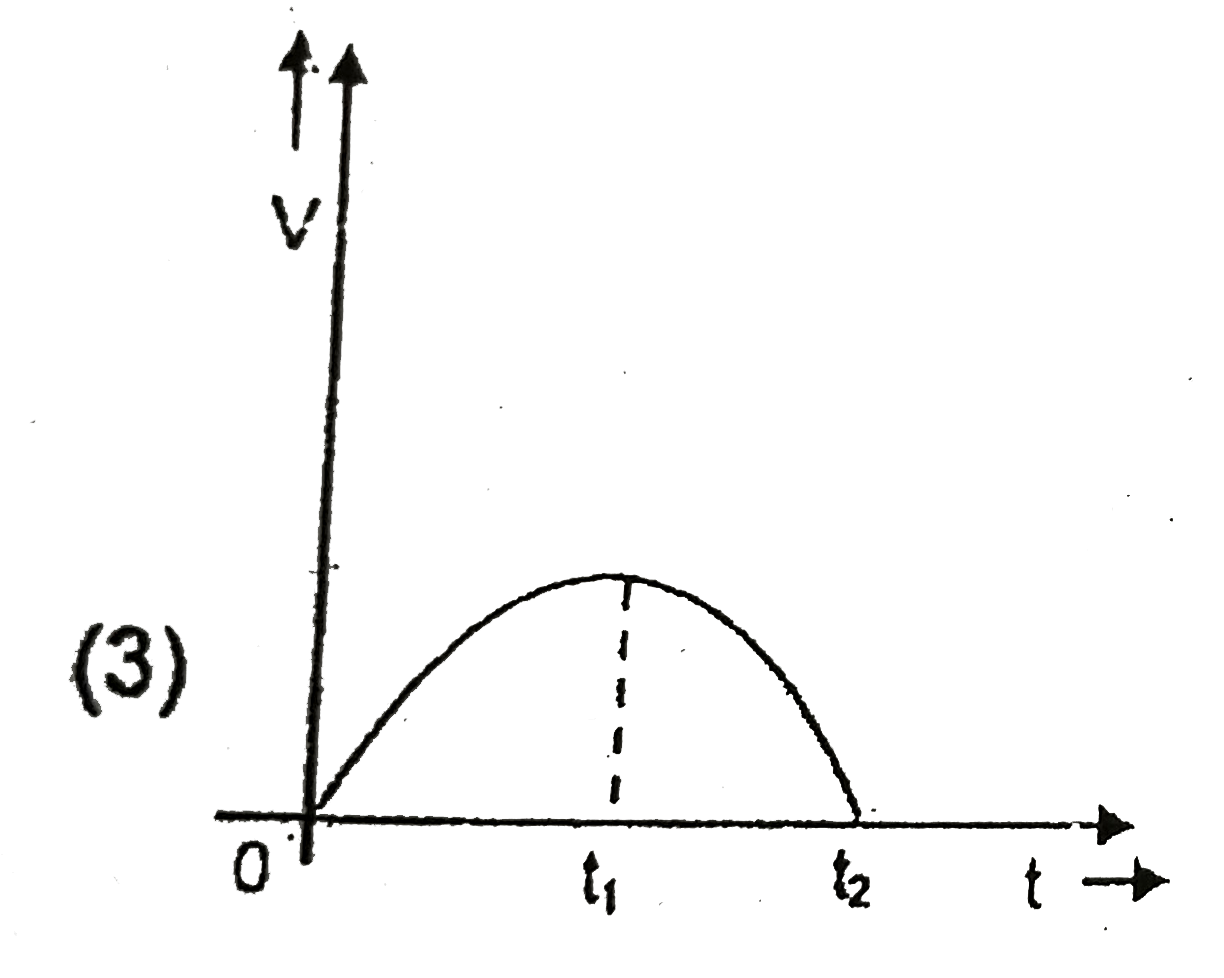 D
D
A particle starting from rest moves along a straight line with constant acceleration for this velocity displacement graph will have the form-
A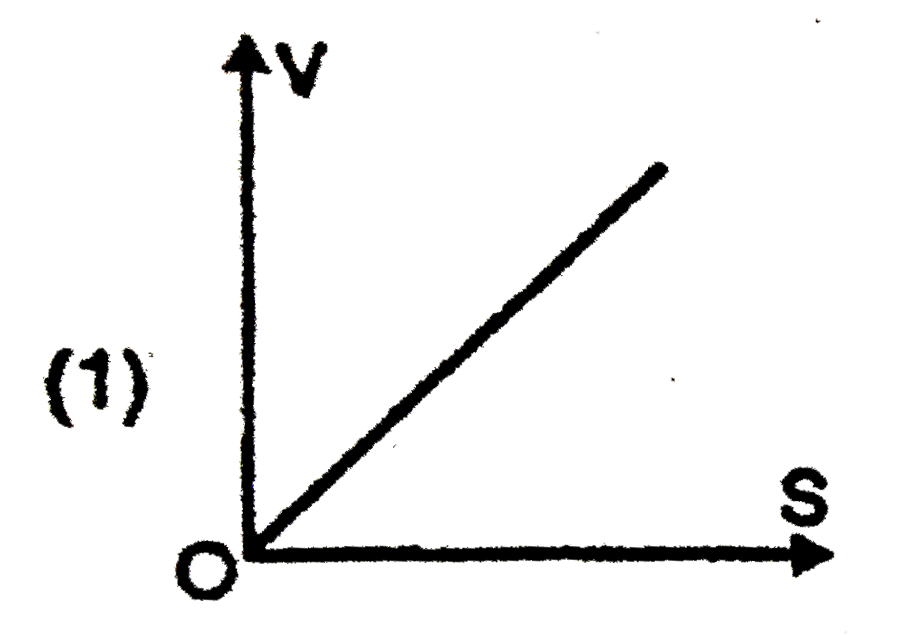 B
B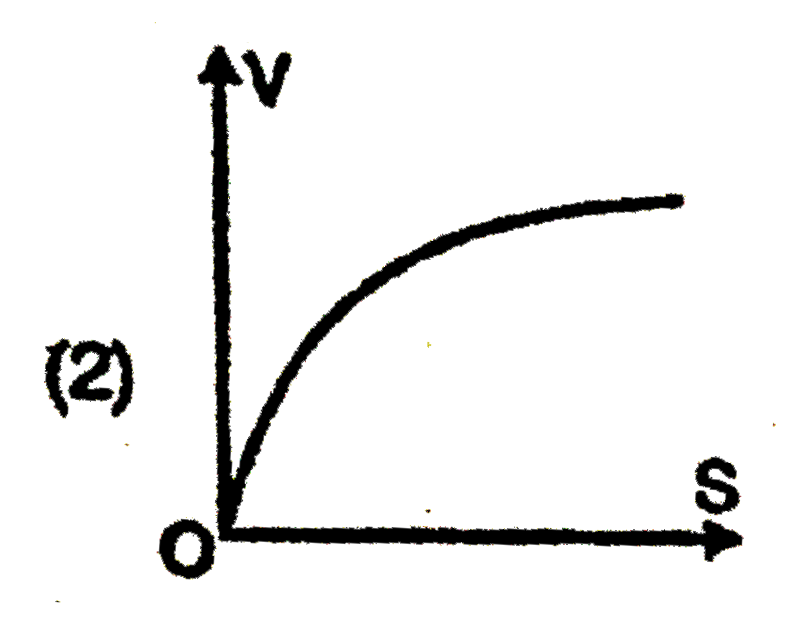 C
C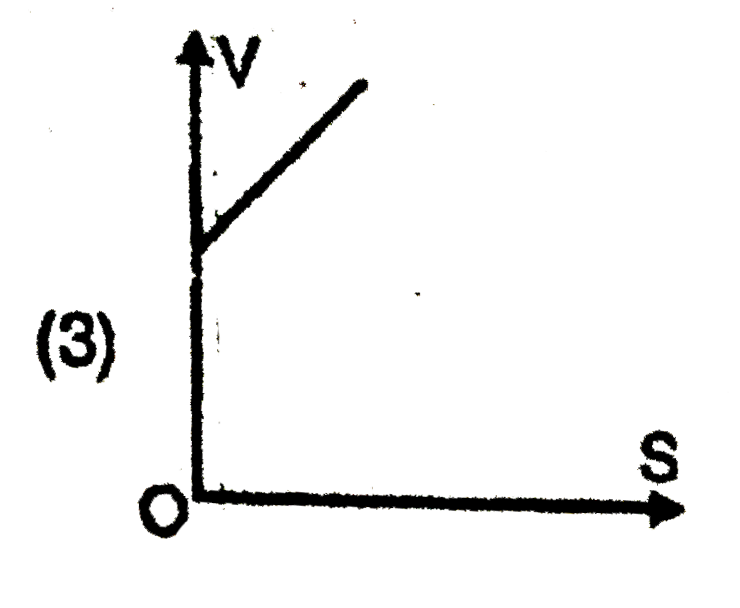 D
D
A particle starts moving from rest in a from rest in a straight line. Its acceleration vs time (a−t) graph is shown in figure. The speed of particle is maximum at.
 At1Bt2Ct1Dt4
At1Bt2Ct1Dt4
The velocity-time graph of a particle moving in a staight line is shown in the . Find the displacement and the distance trav elled by the particle in 6s.
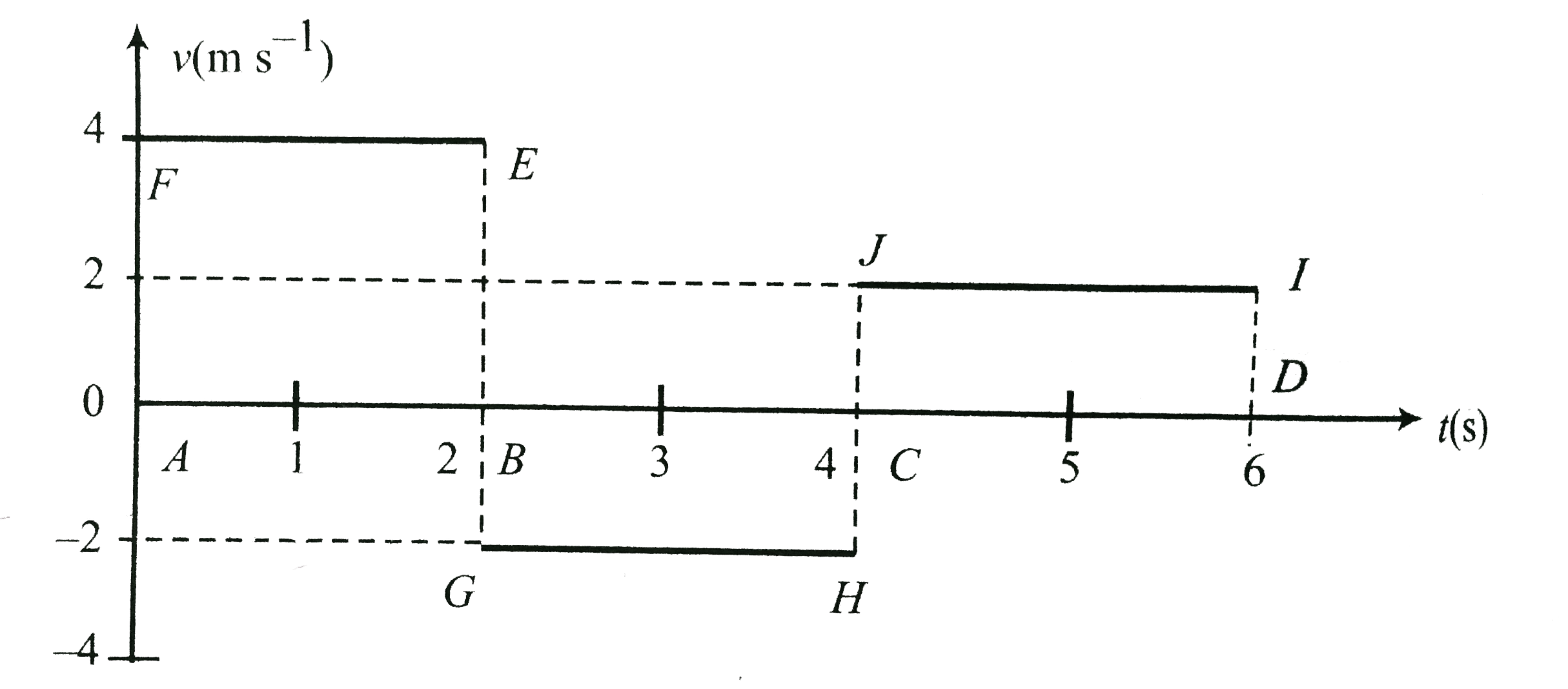 .
.
View SolutionThe figure shows a velocity-time graph of a particle moving along a straight line
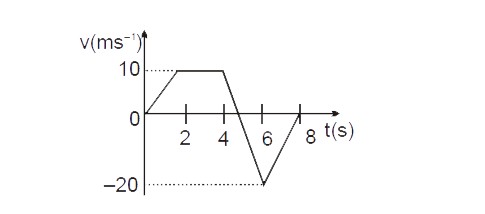
The total distance travelled by the particle is
View SolutionThe figure shows a velocity-time graph of a particle moving along a straight line
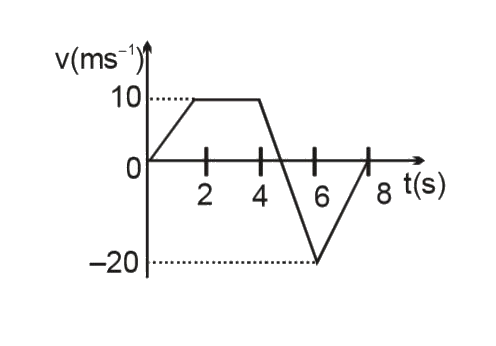
Q. The total distance travelled by the particle is
View SolutionThe figure shows a velocity-time graph of a particle moving along a straight line
The total distance4 travelled by the particel is
View SolutionA body starts from rest at time t=0 , the acceleration time graph is shown in the figure . The maximum velocity attained by the body will be
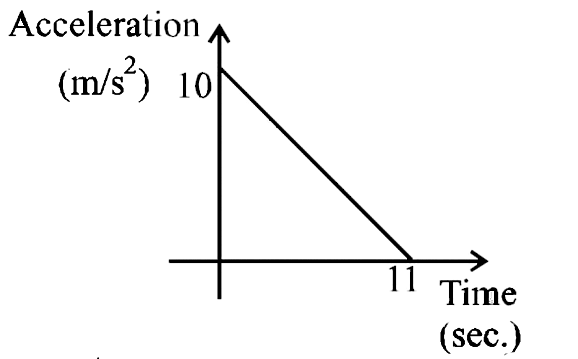
View Solution