PR is a diameter of a circle. A tangent is draewn at the point P and a point S is taken on the tangent of the circle is such a way that PR=PS. If RS intersects the circle at the point T., prove that ST =PT. Solution in Bengali
Let PR be a diameter of the circle with centre at O. A tangent AB is draen at P. The part PS from AB is cut equal to PR. RS intersects the circle at the point T.
To prove : ST=PT
Proof : ∵AB is a tangent at P on the circle with centre at O and OP is a radius passing through P.∴OP⊥AB.
∴∠OPS=90∘or,∠RPT+∠TPS=90∘.....(1)
Again, ∠PTR is a semicircular angle,∴∠PTR=90∘
∴∠TPR+∠TRP=90∘.....(2)
From (1) and (2) we get, ∠RPT+∠TPS=∠TPS=∠TPR+∠TRP
or, ∠TPS=∠TRP∵∠RPT=∠TPRor,∠TPS=∠TSP[∵PS=RP,
therefoer∠TRP=∠RSP]
∴ST=PT[∵ opposite side of equal angles ar equal.]
Hence ST=PT (Proved)

Topper's Solved these Questions
- THEOREMS RELATED TO TANGENT IN A CIRCLE BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO TANGENT IN A CIRCLE EXERCISE - EXERCISE 4.1
20 Videos
- THEOREMS RELATED TO TANGENT IN A CIRCLE BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO TANGENT IN A CIRCLE EXERCISE - EXERCISE 4.2
23 Videos
- THEOREMS RELATED TO ANGLES IN A CIRCLEBOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO ANGLES IN A CIRCLEEXERCISE - EXERCISE 2.3 (Long Answer Type Questions)
14 Videos
- TRIGONOMETRIC RATIOS AND TRIGONOMETRIC IDENTITIES BOOK - CALCUTTA BOOK HOUSECHAPTER - TRIGONOMETRIC RATIOS AND TRIGONOMETRIC IDENTITIES EXERCISE - EXERCISE
66 Videos
Similar Questions
PR and PS are two tangents drawn from a extental point P of the Circle with centre at O. If PR=8cmand∠RPO=60∘, then the length of PS=
View SolutionThe distance of the point from the centre of a circle with diameter of 16 cm is 17 cm. Then the length of the tangent from the point to the circle is
View SolutionProve that angle in the segment of a circle which is greater than a semi-circle is an acute angle.
View SolutionIf tanθ=pq, what is the value of psecθ−q cosecθpsecθ+q cosecθ=
View SolutionLet PQ and RS be tangents at the extremities of the diameter PR of a circle of radius r. PS and RQ intersect at a point X on the circumference of the circle. If PQ = 9 and RS = 4, then the diameter of the circle is :
View SolutionLet PQ and RS be tangent at the extremities of the diameter PR of a circle of radius r. If PS and RQ intersect at a point X on the circumference of the circle, then prove that 2r=√PQ×RS .
View SolutionLet PQ and RS be tangents at the extremities of the diameter PR of a circle of radius r. If PS and RQ intersect at a point X on the circumference of the circle,then 2r equals
View SolutionThree points P,Q ,R lie on a circle. The two perpendiculars PQ and PR and the point P intersect the circle at the points S and T respectively. Prove that RQ=ST
View SolutionPR and PS are two tangents drawn from a extental point P of the Circle with centre at O. If PR=8cmand∠RPO=60∘, then the length of PS=
View SolutionIn the figure, two circles intersect each other in points P and Q. If tangent from point R touch the circles at S and T, then prove that RS=RT.

View SolutionIn the adjoining figure, point Q is the point of contact of tangent and circle . If PQ = 12, PR = 8, then find Ps and RS .
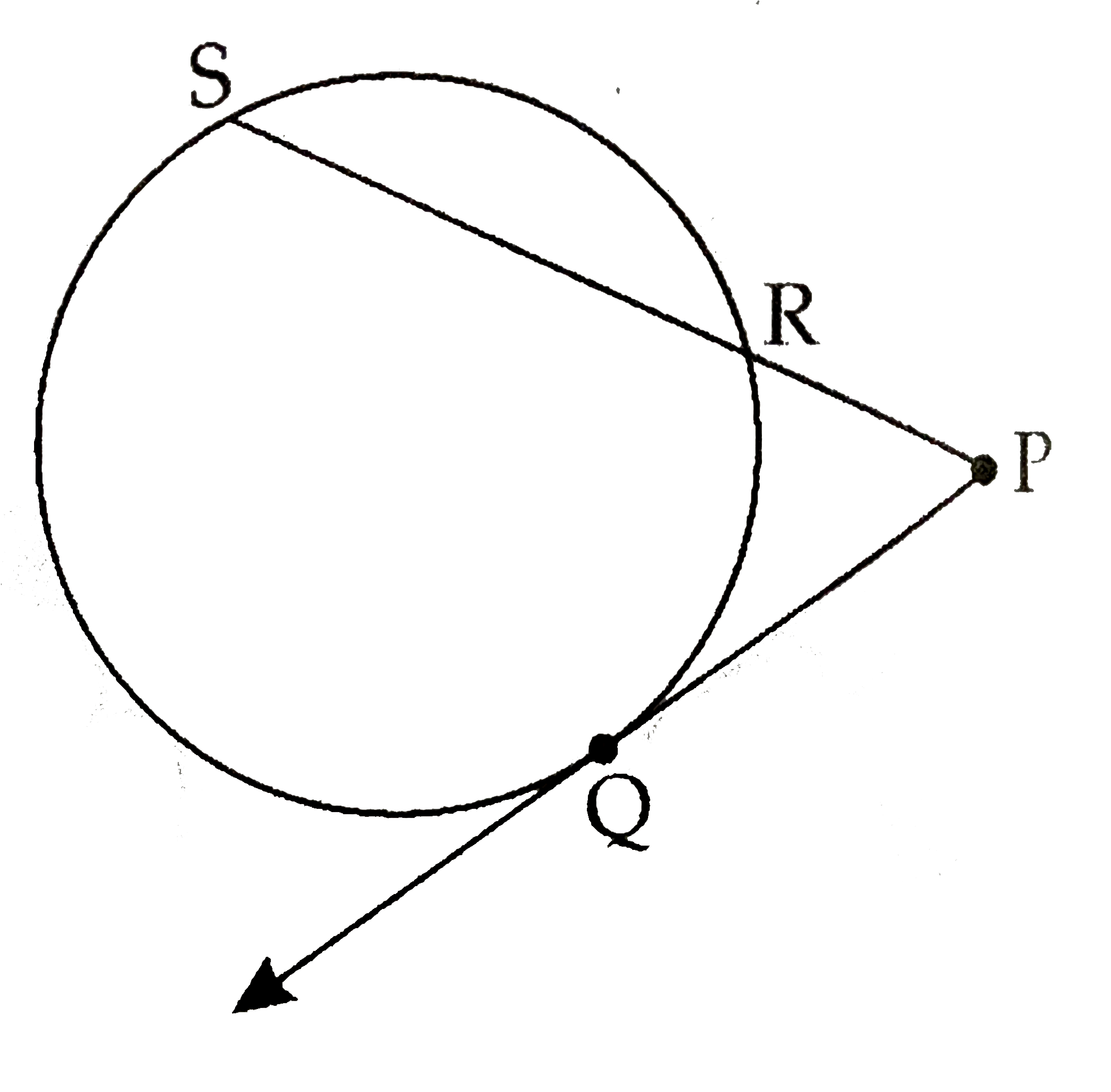
View SolutionLet PQ and RS be tangents at the extremities of the diameter PR of a circle of radius r. If PS and RQ intersect at a point X on the circumference of the circle, then 2r equals:
View SolutionSilver draws a straight line PQ with center R and he draws two circles with diameter PR and PQ. I have drawn a straight line along the point P which intersects the first circle at point S and the second circle at point T. Prove by argument that PS = ST
View SolutionDraw a circle whose PR is a diameter. Draw a tangent to the point P and take the point S above the tangent so that PR = PS. When RS intersects the circle at the point T, prove that ST = RT = PT.
View Solutionमाना त्रिज्या r के वृत्त के व्यास PR के सिरों पर स्पर्श रेखायें PO तथा RS हैं। यदि PS तथा RQ, वृत्त की परिधि के बिन्दु X पर प्रतिच्छेदित हो, तो 2r बराबर है
View Solution
