A particle oscillating under a force →F=−k→x−b→v is a (k and b are constants)
simple harmonic oscillator
linear oscillator
damped osillator
forced oscillator
The correct Answer is:C
A particle oscillating under a force →F=−k→x−b→v is damped oscillator. The first term −k→x represents the restoring force and second term −b→v represents the damping force.
Topper's Solved these Questions
- OSCILLATIONS BOOK - NCERT FINGERTIPSCHAPTER - OSCILLATIONS EXERCISE - Forced Oscillation And Resonance
6 Videos
- OSCILLATIONS BOOK - NCERT FINGERTIPSCHAPTER - OSCILLATIONS EXERCISE - Higher Order Thinking Skills
8 Videos
- OSCILLATIONS BOOK - NCERT FINGERTIPSCHAPTER - OSCILLATIONS EXERCISE - Some Systems Executing Simple Harmonic Motion
29 Videos
- MOTION IN A STRAIGHT LINEBOOK - NCERT FINGERTIPSCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Assertion And Reason
15 Videos
- PHYSICAL WORLDBOOK - NCERT FINGERTIPSCHAPTER - PHYSICAL WORLDEXERCISE - Assertion And Reason
10 Videos
Similar Questions
A particle of mass 0.1kg executes SHM under a force F=−10x(N). Speed of particle at mean position is 6m/s. Find its amplitude of oscillation.
View SolutionLet →u be the initial velocity of a particle and →F be the resultant force acting on it . Describe the path that the particle can take if
(a) →u×→F=0and→F=constant
(b) →u.→F=0and→F=constant
In which case can the particle retrace its path .
View Solution
Knowledge Check
Question Stimulus :-
If a=-bx is a particle motion equation where a is acceleration, x is displacement and b is constant then oscillation time period of particle is-A2√π/bB2π/bC2π/√bD2π√bAparticle of mass m oscillating as given by U(y)=K|y|3 with force constant K has an amplitude A. The maximum velocity during the oscillation is proportional to
AABA3CA3/2DA1/2A particle of mass m oscillates inside a smooth spherical shell of radius R and starts its motion from point B. At given instant the kinetic energy of the particle is K. Then the force applied by particle on the shell at this instant is :−
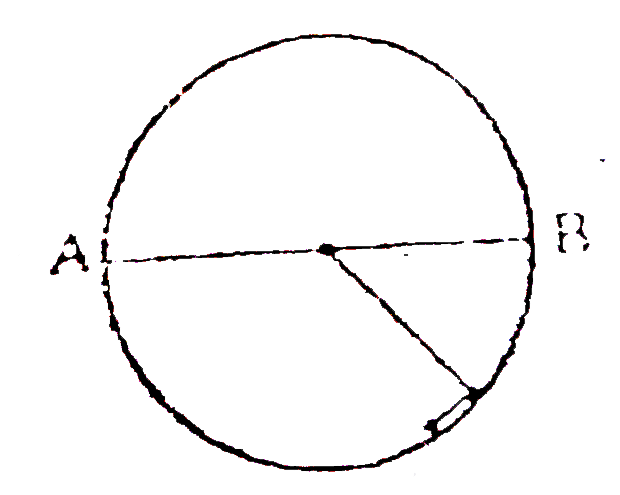 AKRB2KRC3KRDK2R
AKRB2KRC3KRDK2R
A particle is moving under constant acceleration a=kt. The motion starts from rest. The velocity and displacement as a function of time t is
View Solution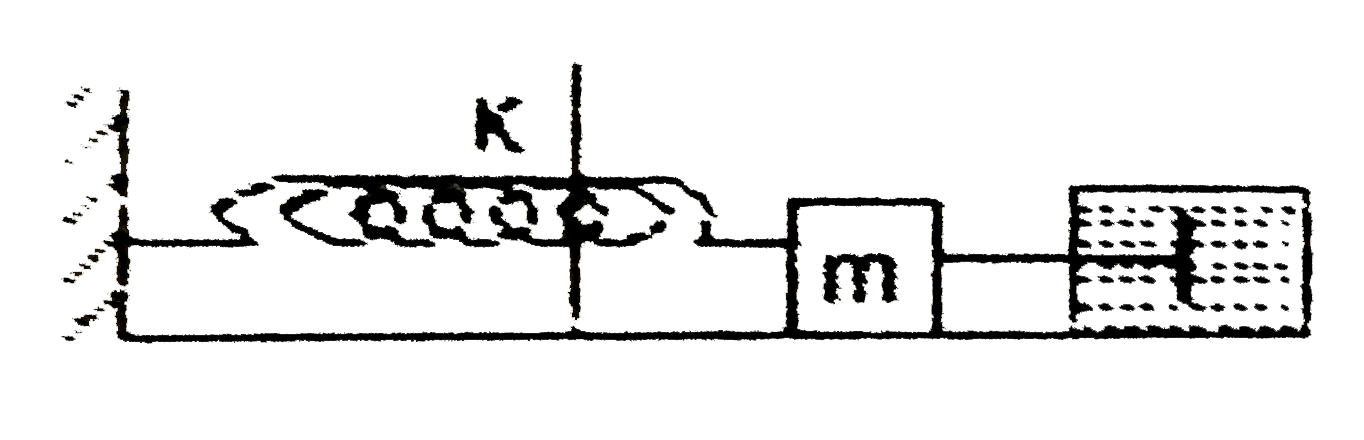
For a damped oscillator which follow the equation →F=−k→x−b→V
the mass of the block is 100 gm K=100N/M and the damping constant is 20 gm/sec. thhen find the time taken for its mechanical energy to drop to one-fourth of its initial value.
View SolutionA particle of mass m is under the influence of a force F which varies with the displacement x according to the relation F=−kx+F0 in which k and F0 are constants. The particle when disturbed will oscillate
View SolutionUnder the action of a force F=−kx3 , the motion of a particle is (k=a positive constant)
View SolutionA particle oscillation is given by (f0)=kpl2 with for constant k and an amplitude A The maximum velocity during the oscillation a preperitiaonal to :
View Solution
