The relation between linear speed v, angular speed $ω$ and angular acceleration $α$ in circular motion is

Video Solution

Text Solution

Verified by Experts

Consider a particle performing circular motion in an anticlockwise sense, along a circle of radius r as shown in the figure. In a very small time interval $δ t$ , the particle moves from point A to point B through a distance $δ s$ and its angular position changes by $δ θ$ .
$∴ δ θ = \frac{a r c A B}{r a d i u s} = \frac{δ S}{r} ∴ s = r δ θ$
THe linear speed v of the particle is the time rate at which the distance is covered by the particle. When $δ t \to 0$ , AB will be a straight line.
$v = \frac{d s}{d t} = lim δ t \to 0 \frac{δ S}{δ t} = lim δ t \to 0 r \frac{δ θ}{δ t}$
$= (lim δ t \to 0 \frac{δ θ}{δ t}) r$
$∴ v = \frac{d t h η}{d t} r ... (1)$

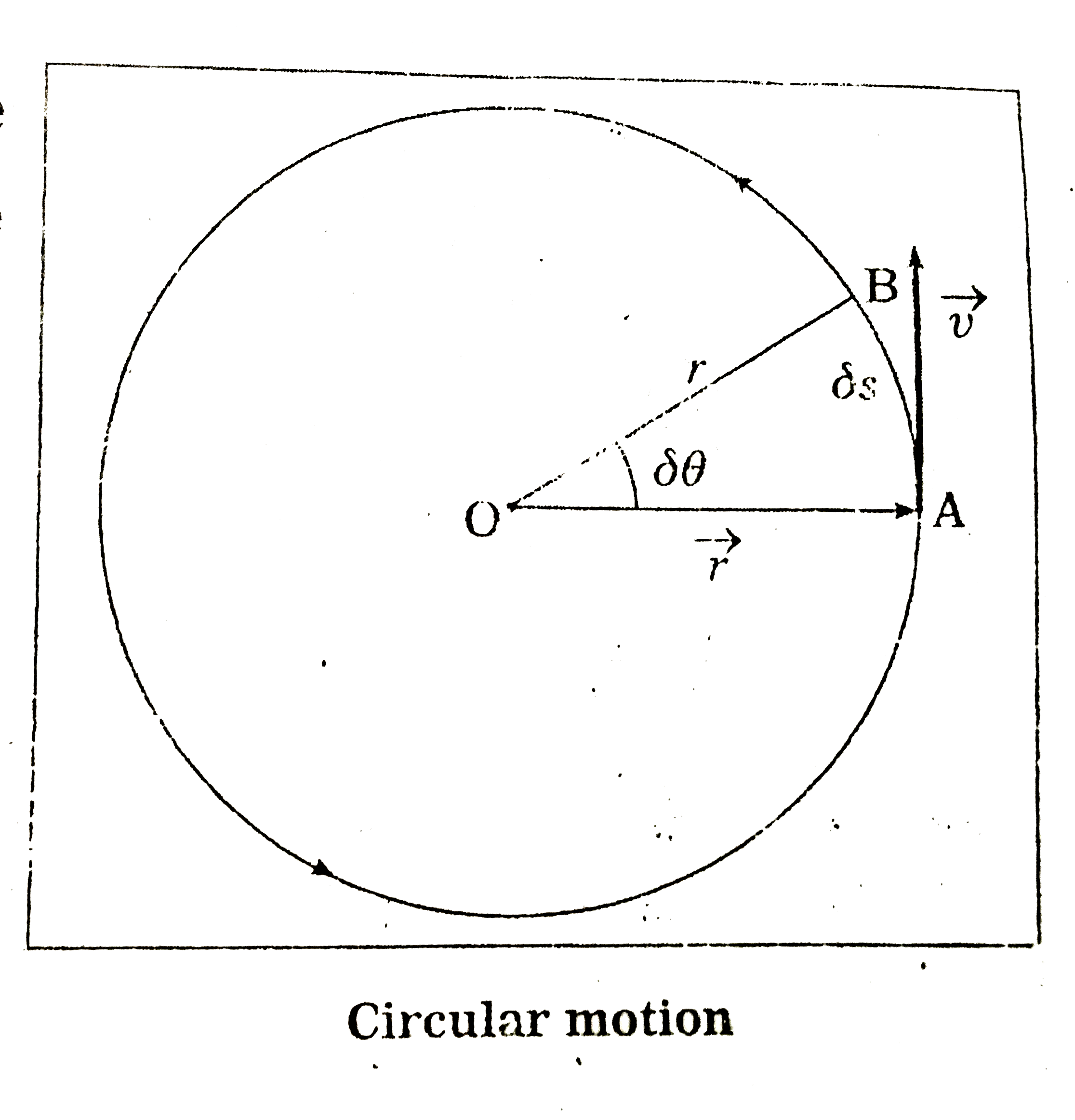
The angular speed $ω$ of the particle is the time rate at which the angle is swept out by the radius vector.
$∴ ω = \frac{d θ}{d t} ... (2)$
From Eqs. (1) and (2), we get,
$v = ω r ... (3)$
THus, linear speed = angular speed xx radius.

Updated on:21/07/2023

The angular speed $ω$ is given by
A $2 π n$
B $2 π / T$
C $2 π / n$
Dboth 'a' and 'b'
The angular displacement in circular motion is
Adimensional quantity
Bdimensionless quantity
Cunitless quantity and dimensionless quantity
Dunitless quantity
The relation between tangential or linear acceleration and angular acceleration of a body moving in circle is given by
A $\to a = \to r / \to α$
B $\to a = \to α \times \to r$
C $\to a = \to α / \to r$
D $\to a = \to r \times α \to$