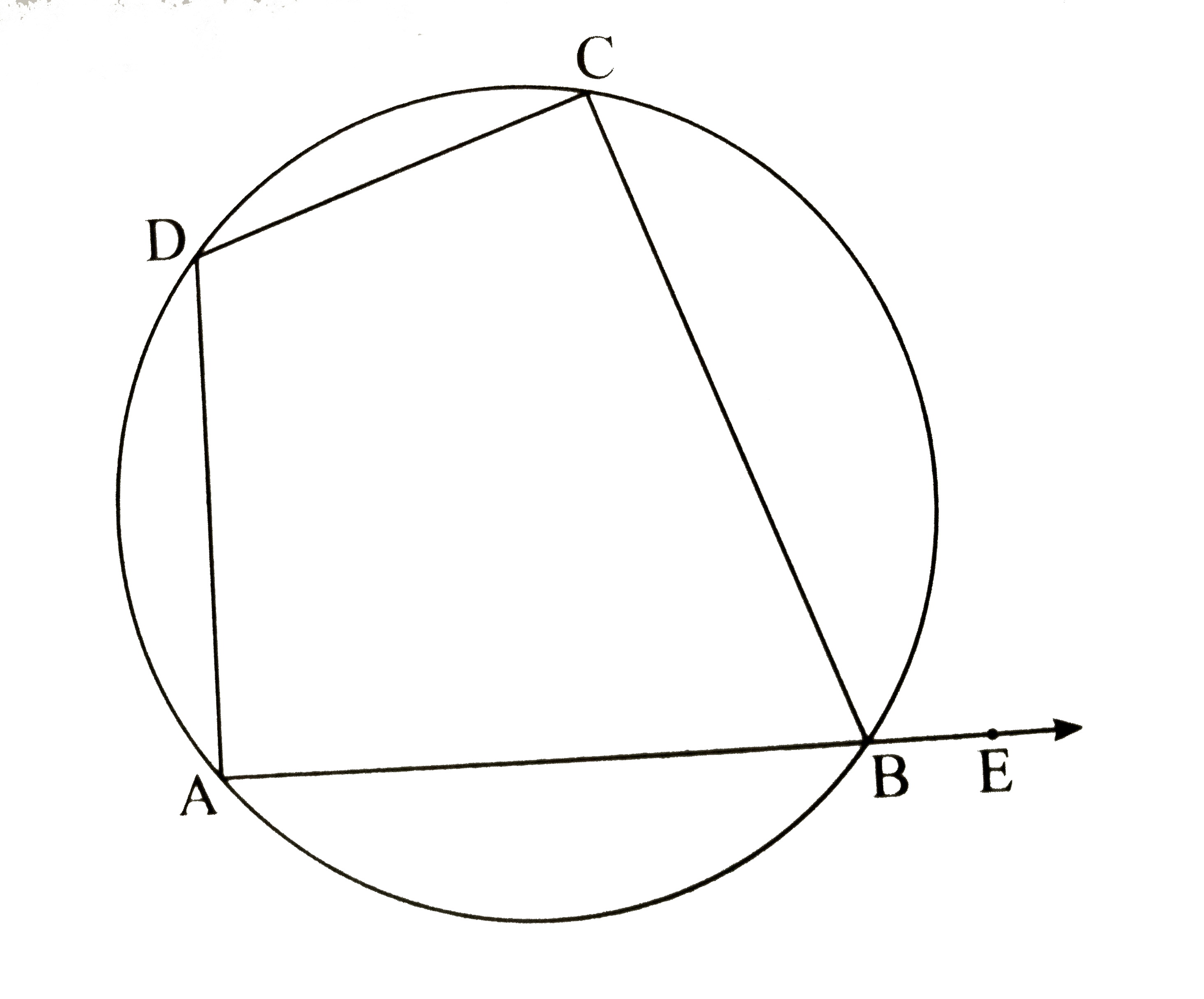
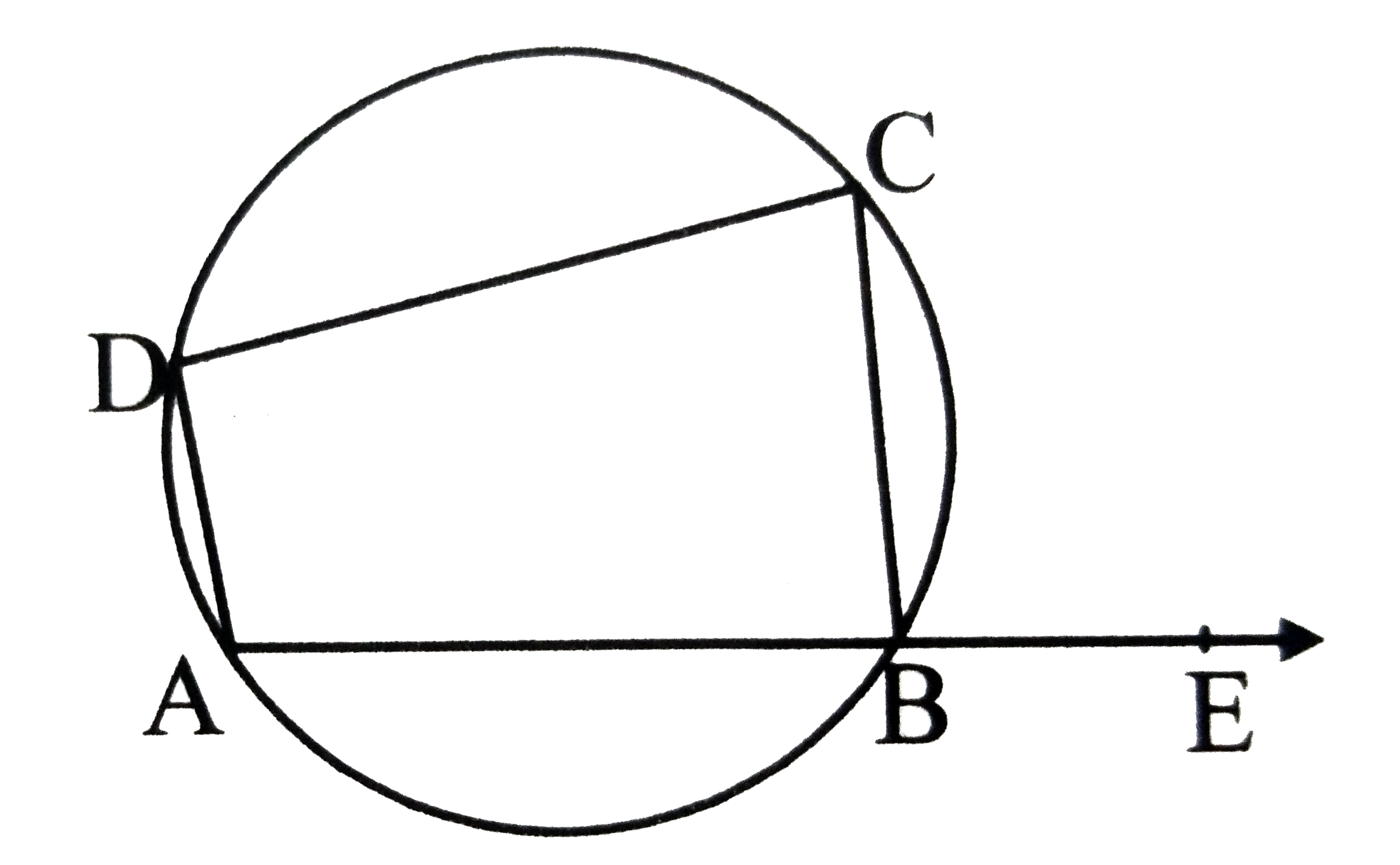
square ABCD is a cyclic quadrilateral in which AB = AD .∠BCD=70∘ . Find (i) m (arc BCD) (ii) m (arc BAD) (III) ∠ADB
To solve the problem step by step, we will find the measures of the arcs BCD and BAD, and the angle ADB in the cyclic quadrilateral ABCD.
Step 1: Understand the Given Information
We have a cyclic quadrilateral ABCD where:
- AB=AD
- ∠BCD=70∘
Step 2: Find the Measure of Arc BCD
Using the Inscribed Angle Theorem, we know that the measure of the arc subtended by an inscribed angle is twice the measure of the angle.
1. Since ∠BCD=70∘, the measure of arc BCD can be calculated as:
m(arc BCD)=2×∠BCD=2×70∘=140∘
Step 3: Find the Measure of Arc BAD
To find the measure of arc BAD, we can use the fact that the total measure of the arcs in a circle is 360∘.
1. Since the arcs BCD and BAD together make the complete circle, we have:
m(arc BAD)=360∘−m(arc BCD)=360∘−140∘=220∘
Step 4: Find the Measure of Angle ADB
To find angle ADB, we can use the properties of isosceles triangles and the fact that angles in a triangle sum up to 180∘.
1. In triangle ABD, since AB=AD, angles ADB and ABD are equal. Let ∠ADB=y.
2. We know that:
∠A+∠B+∠D=180∘
where ∠A=110∘ (since ∠BAD=110∘), and ∠B=∠D=y.
3. Thus, we can write:
110∘+y+y=180∘
110∘+2y=180∘
2y=180∘−110∘=70∘
y=70∘2=35∘
Therefore, ∠ADB=35∘.
Final Answers
- m(arc BCD)=140∘
- m(arc BAD)=220∘
- ∠ADB=35∘
We have a cyclic quadrilateral ABCD where:
- AB=AD
- ∠BCD=70∘
Using the Inscribed Angle Theorem, we know that the measure of the arc subtended by an inscribed angle is twice the measure of the angle.
m(arc BCD)=2×∠BCD=2×70∘=140∘
To find the measure of arc BAD, we can use the fact that the total measure of the arcs in a circle is 360∘.
m(arc BAD)=360∘−m(arc BCD)=360∘−140∘=220∘
To find angle ADB, we can use the properties of isosceles triangles and the fact that angles in a triangle sum up to 180∘.
2. We know that:
∠A+∠B+∠D=180∘
where ∠A=110∘ (since ∠BAD=110∘), and ∠B=∠D=y.
3. Thus, we can write:
110∘+y+y=180∘
110∘+2y=180∘
2y=180∘−110∘=70∘
y=70∘2=35∘
Therefore, ∠ADB=35∘.
- m(arc BCD)=140∘
- m(arc BAD)=220∘
- ∠ADB=35∘
Topper's Solved these Questions
- CIRCLEBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - ASSIGNMENT 4.5
8 Videos
- CIRCLEBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - EXAMPLES FOR PRACTICE
40 Videos
- CIRCLEBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - ASSIGNMENT 4.3
11 Videos
- CHALLENGING QUESTIONSBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CHALLENGING QUESTIONSEXERCISE - SECTION 3 (MODEL QUESTION PAPER FOR PRACTICE ) Solve any one of the following subquestions :
1 Videos
- COORDINATE GEOMETRY BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - COORDINATE GEOMETRY EXERCISE - Assignment 6.5
13 Videos
Similar Questions
Knowledge Check
ABCD is a cyclic quadrilateral in which AB = 15 cm, BC = 12 cm and CD = 10 cm. If AC bisects BD, then what is the measure of AD?
A15 cmB13.5 cmC18 cmD20 cm
□ABCD is a cyclic quadrilaterial. M (arc ABC) =230∘. Find ∠ABC,∠CDAand∠CBE.
View SolutionIn Figure ABCD is a cyclic quadrilateral. If ∠BCD=1000and ∠ABD=700, find ∠ADB
View SolutionABCD is a cyclic quadrilateral. If AD∣∣BCand∠B=70∘, find the other angles of ABCD.
View SolutionABCD is a quadrilateral in which ∠ABC=∠BCD and CD>AB Prove that ∠BAD>∠ADC
View SolutionABCD is a cyclic trapezium in which, AD| |BC and ∠B=70∘. Find its remaining angles,
View SolutionIn a cyclic quadrilateral ABCD , if ∠A=3∠C). Find ∠A
View SolutionABCD is a quadrilateral in which AD = BC and ∠DAB=∠CBA Prove that (i) ΔABD≅ΔBAC (ii) BD = AC (iii) ∠ABD=∠BAC
View Solution