Given that ΔTf is the depression in freezing point of the solvent in a solution of a non-volatile solute of molarity m,the quantity Ltm→0(ΔTf/m) is equal to ……………. .
To solve the problem, we need to analyze the relationship between the depression in freezing point (ΔTf), molarity (m), and the freezing point depression constant (Kf) for a non-volatile solute.
1. Understand the Formula for Depression in Freezing Point:
The depression in freezing point (ΔTf) is given by the formula:
ΔTf=Kf⋅m⋅i
where:
- Kf is the freezing point depression constant,
- m is the molarity of the solution,
- i is the van 't Hoff factor (which is 1 for non-volatile solutes).
2. Consider the Limit as Molarity Approaches Zero:
We are interested in the quantity:
limm→0(ΔTfm)
As the molarity m approaches zero, we need to analyze what happens to ΔTf.
3. Substituting the Formula into the Limit:
Substitute the expression for ΔTf from the first step into the limit:
ΔTfm=Kf⋅m⋅im
Here, since i=1 for a non-volatile solute, we can simplify this to:
ΔTfm=Kf⋅i=Kf⋅1=Kf
4. Taking the Limit:
Now, as m approaches 0, the expression simplifies to:
limm→0(ΔTfm)=Kf
5. Conclusion:
Therefore, the quantity limm→0(ΔTfm) is equal to Kf.
Final Answer:
limm→0(ΔTfm)=Kf
---
The depression in freezing point (ΔTf) is given by the formula:
ΔTf=Kf⋅m⋅i
where:
- Kf is the freezing point depression constant,
- m is the molarity of the solution,
- i is the van 't Hoff factor (which is 1 for non-volatile solutes).
We are interested in the quantity:
limm→0(ΔTfm)
As the molarity m approaches zero, we need to analyze what happens to ΔTf.
Substitute the expression for ΔTf from the first step into the limit:
ΔTfm=Kf⋅m⋅im
Here, since i=1 for a non-volatile solute, we can simplify this to:
ΔTfm=Kf⋅i=Kf⋅1=Kf
Now, as m approaches 0, the expression simplifies to:
limm→0(ΔTfm)=Kf
Therefore, the quantity limm→0(ΔTfm) is equal to Kf.
limm→0(ΔTfm)=Kf
Topper's Solved these Questions
- SOLUTIONSBOOK - CENGAGE CHEMISTRYCHAPTER - SOLUTIONSEXERCISE - Exercises Archives (Subjective)
18 Videos
- SOLUTIONSBOOK - CENGAGE CHEMISTRYCHAPTER - SOLUTIONSEXERCISE - Ex 2.1 (Objective)
10 Videos
- SOLUTIONSBOOK - CENGAGE CHEMISTRYCHAPTER - SOLUTIONSEXERCISE - Exercises Archives (Integer)
1 Videos
- SOLID STATEBOOK - CENGAGE CHEMISTRYCHAPTER - SOLID STATEEXERCISE - Ex 1.2 (Objective)
9 Videos
- SURFACE CHEMISTRYBOOK - CENGAGE CHEMISTRYCHAPTER - SURFACE CHEMISTRYEXERCISE - Archives Subjective
2 Videos
Similar Questions
Why is depression of freezing point of 0.1 M sodium chloride solution nearly twice that of 0.1 M glucose solution?
View SolutionState Raoult's law, If ΔT is the elevation in boiling point of a solvent and m is no. of moles of solute per kilogram of solvent. What is the relationship between ΔT and m?
View Solution
Knowledge Check
Given that ΔTf is the depression in freezing point of the solvent in a solution of a non-volatile solute of molarity m,the quantity Ltm→0(ΔTf/m) is equal to ……………. .
AZeroBOneCThreeDNone of the aboveConsider following figure and answer the questions at the end of it.
Figure explains elevation in boiling point when a non-volatile solute is added to a solvent.
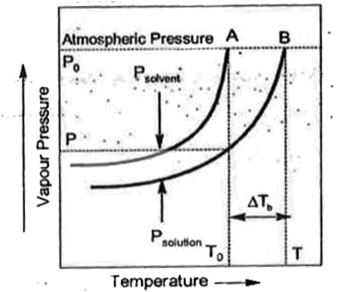
Variation of vapour pressure with temperature and showing elevation in boiling point.
Given that ΔTb is the elevation in boiling point of the solvent in a solution of molality 'm' then Ltm→0(DηlTbm) is equal toAKb (molal elevation constant)BLv (latent heat of vaporization)CΔS (entropy change)Dx (mole fraction of solute)Consider following figure and answer the questions at the end of it. Figure explains elevation in boiling point when a non-volatile solute is added to a solvent.
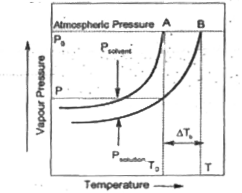
Variation of vapour pressure with temperature and showing elevation in boiling point.
Given that ΔTb is the elevation in boiling point of the solvent in a solution of molality m then Ltm→0(ΔTbm) is equal toAKb (molal elevation constant)BLy (latent heat of vaporization)CΔS (entropy change)Dx (mole fraction of solute)
Figure explains elevation in boiling point when a non-volatile solute is added to a solvent. Variation of vapour pressure with temperaure and elevation in boiling point is marked.
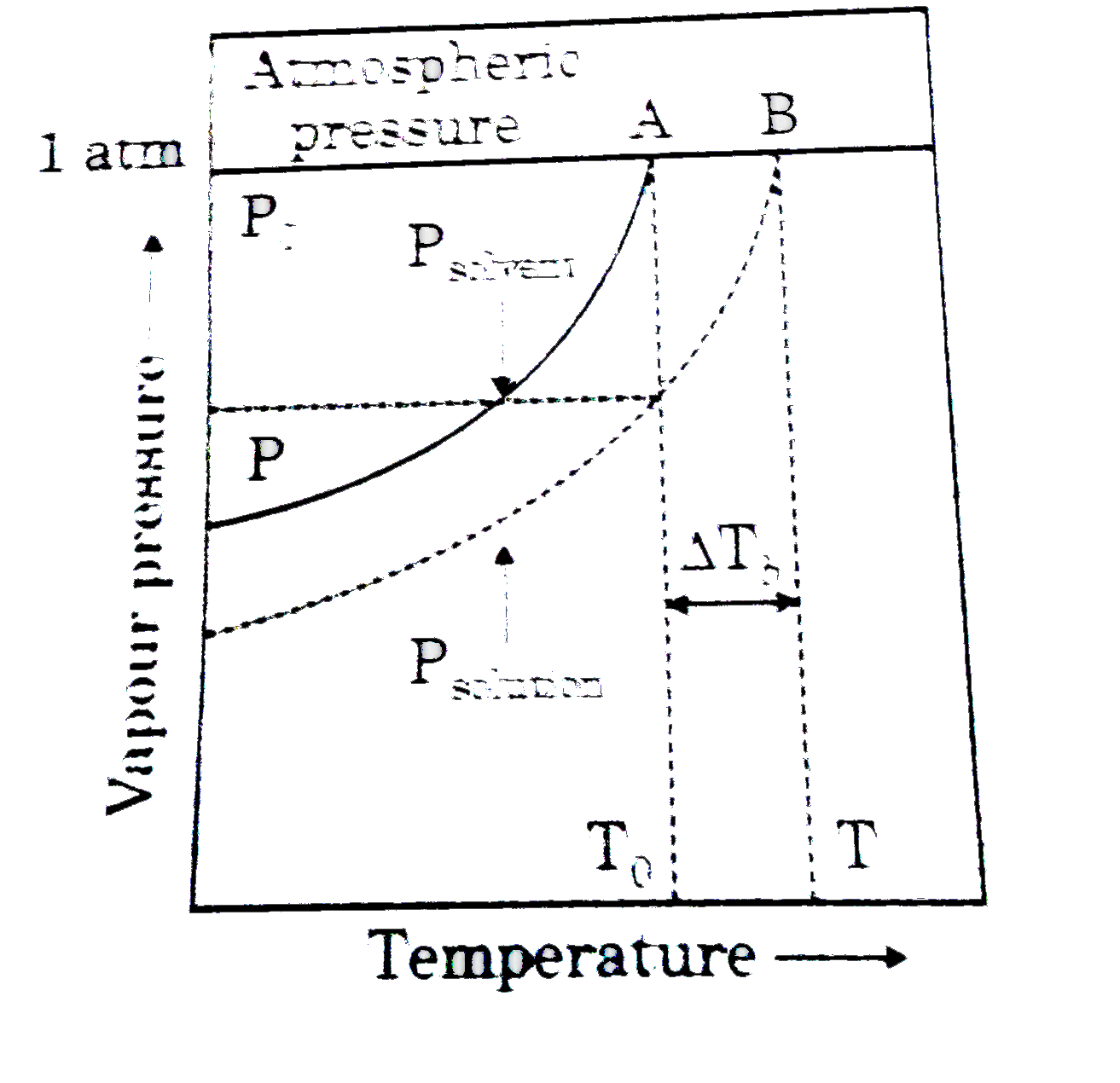
Given that ΔTb is the elevation in boiling point of the solvent in a solution of molarity m then limm→0(ΔTbm) is equal to:
View SolutionIf all symbols have their usual meaning, then for a non-volatile non-electrolyte solute Ltm→0(ΔTfm) is equal to
View SolutionThe freezing point of a 0.05 molal solution of a non-electrolyte in water is [Kf=1.86K/m]
View SolutionThe molal depression constant for a solvent is 4.9. The depression in freezing point for a millimolal solution of a non-electrolyte in the solvent is
View SolutionIn coparison to a 0.01 M solution of glucose, the depression in freezing point of a 0.01 M MgCl2 solution is……
View Solution
