Question

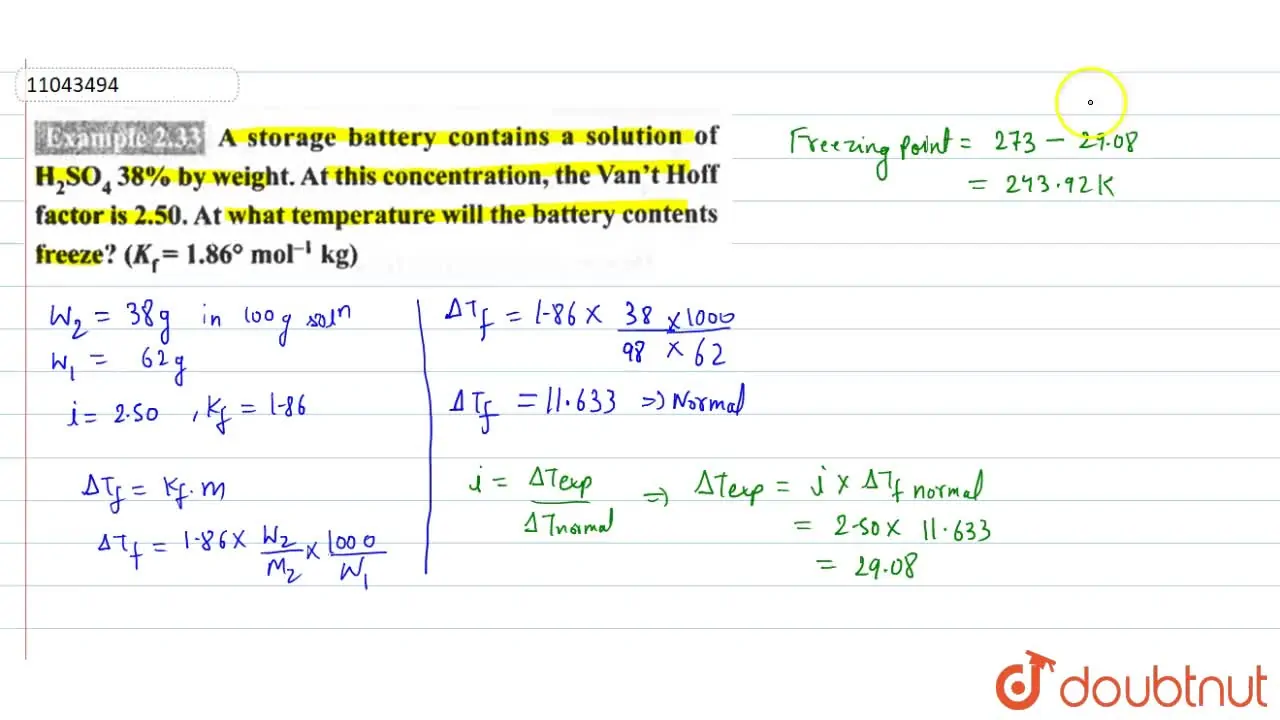
A storage battery contains a solution of $H_{2}S{O}_{4}38\%$ by weight. At this concentration, the Vant't Hoff factor is $2.50$. At what temperature will the battery contents freeze? $(K_f={1.86}^{\circ }mo{l}^{-1}kg)$

Explanation

To solve the problem of determining the freezing point of a storage battery solution containing 38% H₂SO₄ by weight, we will follow these steps:

Step 1: Determine the mass of the solute and solvent
Given that the solution is 38% H₂SO₄ by weight, we can assume:
- Mass of H₂SO₄ (solute), W₂ = 38 g
- Mass of the solution = 100 g
- Therefore, mass of the solvent (water), W₁ = 100 g - 38 g = 62 g

Step 2: Calculate the molality of the solution
The molality (m) of the solution is calculated using the formula:
\( m = \frac{W₂}{M₂} \times \frac{1000}{W₁} \)
Where:
- \(W₂\) = mass of the solute (H₂SO₄) = 38 g
- \(M₂\) = molar mass of H₂SO₄ = 98 g/mol
- \(W₁\) = mass of the solvent (water) = 62 g

Substituting the values:
\( m = \frac{38 \, \text{g}}{98 \, \text{g/mol}} \times \frac{1000}{62 \, \text{g}} \)
Calculating this gives:
\( m = \frac{38}{98} \times \frac{1000}{62} \approx 6.16 \, \text{mol/kg} \)

Step 3: Calculate the depression in freezing point (ΔTf)
The depression in freezing point can be calculated using the formula:
\( \Delta T_f = i \cdot K_f \cdot m \)
Where:
- \(i\) = Van't Hoff factor = 2.50
- \(K_f\) = freezing point depression constant = 1.86 °C kg/mol
- \(m\) = molality calculated in the previous step

Substituting the values:
\( \Delta T_f = 2.50 \cdot 1.86 \cdot 6.16 \)
Calculating this gives:
\( \Delta T_f \approx 28.69 \, °C \)

Step 4: Determine the freezing point of the solution
The normal freezing point of water is 0 °C (or 273 K). The freezing point of the solution can be found by subtracting the depression from the normal freezing point:
\( \text{Freezing Point} = 0 °C - \Delta T_f \)
Substituting the value of ΔTf:
\( \text{Freezing Point} = 0 °C - 28.69 °C \approx -28.69 °C \)

Final Answer
The freezing point of the battery contents is approximately -28.69 °C.

---