The first ionization constant of H2S is 9.1×10−8. Calculate the concentration of HSΘ ion in its 0.1M solution. How will this concentration be affected if the solution is 0.1M in HCl also? If the second dissociation constant if H2S is 1.2×10−13, calculate the concentration of S2− under both conditions.
To solve the problem, we will follow these steps:
Step 1: Calculate the concentration of HS⁻ in a 0.1M solution of H₂S.
The first dissociation of H₂S can be represented as:
\( H_2S \rightleftharpoons H^+ + HS^- \)
Let the initial concentration of H₂S be 0.1M. If we let x be the concentration of H+ and HS⁻ produced, we can set up the following equilibrium expression based on the first ionization constant \( K_{a1} \):
\( K_{a1} = \frac{(H^+)(HS^-)}{(H_2S)} \)
Given:
- \( K_{a1} = 9.1 \times 10^{-8} \)
- Initial concentration of \( H_2S = 0.1 \, M \)
At equilibrium:
- Concentration of \( H_2S = 0.1 - x \)
- Concentration of \( H^+ = x \)
- Concentration of \( HS^- = x \)
Substituting these into the equilibrium expression gives:
\( 9.1 \times 10^{-8} = \frac{x \cdot x}{0.1 - x} \)
Assuming \( x \) is small compared to 0.1, we can simplify to:
\( 9.1 \times 10^{-8} = \frac{x^2}{0.1} \)
Rearranging gives:
\( x^2 = 9.1 \times 10^{-8} \times 0.1 \)
\( x^2 = 9.1 \times 10^{-9} \)
\( x = \sqrt{9.1 \times 10^{-9}} \)
\( x \approx 9.54 \times 10^{-5} \, M \)
Thus, the concentration of HS⁻ in a 0.1M solution of H₂S is approximately:
\( (HS^-) \approx 9.54 \times 10^{-5} \, M \)
Step 2: Calculate the concentration of HS⁻ in a 0.1M solution of HCl.
When HCl is added, it provides a common ion \( H^+ \). The dissociation of HCl can be represented as:
\( HCl \rightarrow H^+ + Cl^- \)
The concentration of \( H^+ \) from HCl is 0.1M. Thus, the total concentration of \( H^+ \) becomes:
\( (H^+) = 0.1 + x \approx 0.1 \, M \) (since \( x \) is negligible)
Now, we set up the equilibrium expression again:
\( K_{a1} = \frac{(H^+)(HS^-)}{(H_2S)} \)
Substituting the known values:
\( 9.1 \times 10^{-8} = \frac{(0.1)(y)}{0.1} \)
Where \( y \) is the concentration of \( HS^- \).
This simplifies to:
\( 9.1 \times 10^{-8} = y \)
Thus, the concentration of HS⁻ in the presence of 0.1M HCl is:
\( (HS^-) \approx 9.1 \times 10^{-8} \, M \)
Step 3: Calculate the concentration of S²⁻ under both conditions.
The second dissociation of H₂S can be represented as:
\( HS^- \rightleftharpoons H^+ + S^{2-} \)
For the first case (without HCl):
Using the second dissociation constant \( K_{a2} \):
\( K_{a2} = 1.2 \times 10^{-13} \)
The equilibrium expression is:
\( K_{a2} = \frac{(H^+)(S^{2-})}{(HS^-)} \)
Substituting the values we have:
\( 1.2 \times 10^{-13} = \frac{(9.54 \times 10^{-5})(z)}{(9.54 \times 10^{-5})} \)
Where \( z \) is the concentration of \( S^{2-} \).
This simplifies to:
\( 1.2 \times 10^{-13} = z \)
Thus, the concentration of S²⁻ in the absence of HCl is:
\( (S^{2-}) \approx 1.2 \times 10^{-13} \, M \)
For the second case (with HCl):
Using the same approach:
\( K_{a2} = \frac{(0.1)(X)}{(9.1 \times 10^{-8})} \)
Where \( X \) is the concentration of \( S^{2-} \).
This gives:
\( 1.2 \times 10^{-13} = \frac{(0.1)(X)}{(9.1 \times 10^{-8})} \)
Rearranging gives:
\( X = \frac{1.2 \times 10^{-13} \times (9.1 \times 10^{-8})}{0.1} \)
\( X = 1.092 \times 10^{-19} \, M \)
Thus, the concentration of S²⁻ in the presence of 0.1M HCl is:
\( (S^{2-}) \approx 1.092 \times 10^{-19} \, M \)
Summary of Results:
- Concentration of HS⁻ in 0.1MH₂S: \( 9.54 \times 10^{-5} \, M \)
- Concentration of HS⁻ in 0.1MHCl: \( 9.1 \times 10^{-8} \, M \)
- Concentration of S²⁻ in 0.1MH₂S: \( 1.2 \times 10^{-13} \, M \)
- Concentration of S²⁻ in 0.1MHCl: \( 1.092 \times 10^{-19} \, M \)
\( H_2S \rightleftharpoons H^+ + HS^- \)
- \( K_{a1} = 9.1 \times 10^{-8} \)
- Initial concentration of \( H_2S = 0.1 \, M \)
- Concentration of \( H_2S = 0.1 - x \)
- Concentration of \( H^+ = x \)
- Concentration of \( HS^- = x \)
\( 9.1 \times 10^{-8} = \frac{x \cdot x}{0.1 - x} \)
\( 9.1 \times 10^{-8} = \frac{x^2}{0.1} \)
\( x^2 = 9.1 \times 10^{-8} \times 0.1 \)
\( x^2 = 9.1 \times 10^{-9} \)
\( x = \sqrt{9.1 \times 10^{-9}} \)
\( x \approx 9.54 \times 10^{-5} \, M \)
\( (HS^-) \approx 9.54 \times 10^{-5} \, M \)
\( HCl \rightarrow H^+ + Cl^- \)
\( (H^+) = 0.1 + x \approx 0.1 \, M \) (since \( x \) is negligible)
\( K_{a1} = \frac{(H^+)(HS^-)}{(H_2S)} \)
\( 9.1 \times 10^{-8} = \frac{(0.1)(y)}{0.1} \)
Where \( y \) is the concentration of \( HS^- \).
\( 9.1 \times 10^{-8} = y \)
\( (HS^-) \approx 9.1 \times 10^{-8} \, M \)
\( HS^- \rightleftharpoons H^+ + S^{2-} \)
Using the second dissociation constant \( K_{a2} \):
\( K_{a2} = 1.2 \times 10^{-13} \)
\( K_{a2} = \frac{(H^+)(S^{2-})}{(HS^-)} \)
\( 1.2 \times 10^{-13} = \frac{(9.54 \times 10^{-5})(z)}{(9.54 \times 10^{-5})} \)
Where \( z \) is the concentration of \( S^{2-} \).
\( 1.2 \times 10^{-13} = z \)
\( (S^{2-}) \approx 1.2 \times 10^{-13} \, M \)
Using the same approach:
\( K_{a2} = \frac{(0.1)(X)}{(9.1 \times 10^{-8})} \)
Where \( X \) is the concentration of \( S^{2-} \).
\( 1.2 \times 10^{-13} = \frac{(0.1)(X)}{(9.1 \times 10^{-8})} \)
\( X = \frac{1.2 \times 10^{-13} \times (9.1 \times 10^{-8})}{0.1} \)
\( X = 1.092 \times 10^{-19} \, M \)
\( (S^{2-}) \approx 1.092 \times 10^{-19} \, M \)
- Concentration of HS⁻ in 0.1MH₂S: \( 9.54 \times 10^{-5} \, M \)
- Concentration of HS⁻ in 0.1MHCl: \( 9.1 \times 10^{-8} \, M \)
- Concentration of S²⁻ in 0.1MH₂S: \( 1.2 \times 10^{-13} \, M \)
- Concentration of S²⁻ in 0.1MHCl: \( 1.092 \times 10^{-19} \, M \)
Topper's Solved these Questions
- NCERT BASED EXERCISEBOOK - CENGAGE CHEMISTRYCHAPTER - NCERT BASED EXERCISEEXERCISE - Thermodynamics
50 Videos
- ISOMERISMBOOK - CENGAGE CHEMISTRYCHAPTER - ISOMERISMEXERCISE - Assertion-Reasoning Type
1 Videos
- ORGANIC REACTION MECHANISMBOOK - CENGAGE CHEMISTRYCHAPTER - ORGANIC REACTION MECHANISMEXERCISE - Analytical and Descriptive
6 Videos
Similar Questions
The first ionization constant of H2S is 9.1×10−8. Calculate the concentration of HSΘ ion in its 0.1M solution. How will this concentration be affected if the solution is 0.1M in HCl also? If the second dissociation constant if H2S is 1.2×10−13, calculate the concentration of S2− under both conditions.
View SolutionCalculate the concentration of H+ ion in 5×10−1M solution of H2SO4
View Solution
Knowledge Check
The first ionization constant of H2S is 9.1×10−8. Calculate the concentration of HS− ion in its 0.1 M solution. How will this concentration be affected, if the solution is 0.1 M in HCl also? If the second dissociation constant of H2S is 1.2×10−13 , then calculate the concentration of S2− under both conditions. Select these four answers from the choices given below.
A B
B C
C DNone of these
DNone of theseThe first ionization constant of H2S is 9.1×10−8. Calculate the concentration of HS− ion in its 0.1 M solution. How will this concentration be affected, if the solution is 0.1 M in HCl also? If the second dissociation constant of H2S is 1.2×10−13 , then calculate the concentration of S2− under both conditions. Select these four answers from the choices given below.
A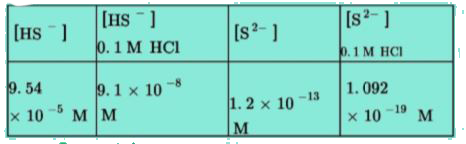 B
B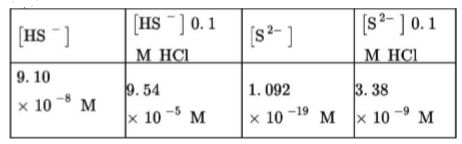 C
C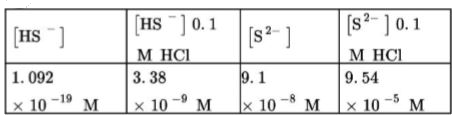 DNone of these
DNone of these
The ionization constant of HF is 3.2×10−4. Calculate the degree of ionization of HF in its 0.02M solution. Calculate the concentration of all species present in the solution and its pH.
View SolutionThe ionization constant of acetic acid 1.74×10−5. Calculate the degree of dissociation of acetic acid in its 0.05M solution. Calculate the concentration of acetate ion in the solution and its pH.
View SolutionThe ionization constant of acetic acid 1.74×10−5. Calculate the degree of dissociation of acetic acid in its 0.05M solution. Calculate the concentration of acetate ion in the solution and its pH.
View SolutionThe dissociation constatn of 0.01 M CH3COOH is 1.8×10−5 then calculate CH3COO− concentration of 0.1 M HCl solution.
View SolutionThe ionization constant of propanoic acid is 1.32×10−5. Calculate the degree of ionization of the acid in its 0.05M solution and also its pH. What will be its degree of ionization if the solution is 0.01M on HCl also?
View SolutionThe ionization constant of propanoic acid is 1.32×10−5. Calculate the degree of ionization of the acid in its 0.05M solution and also its pH. What will be its degree of ionization if the solution is 0.01M on HCl also?
View Solution
