Malonic acid is an organic dibasic acid such as $H_{2} S$ having first ionistion constant, $K_{1} = 1.42 \times 10^{- 3}$ and second ionisation constant, $K_{2} = 2.0 \times 10^{- 6}$ . Compute the divalent molanate ion concentration in:
a. $0.001 M$ malonic acid.
b. a solution that is $0.0001 M$ in malonc acid and $0.0004 M H C 1$ .
c. a solution that is $0.0001 M$ in malonic acid and $0.1 M H C 1$ .

Video Solution

Text Solution

Verified by Experts

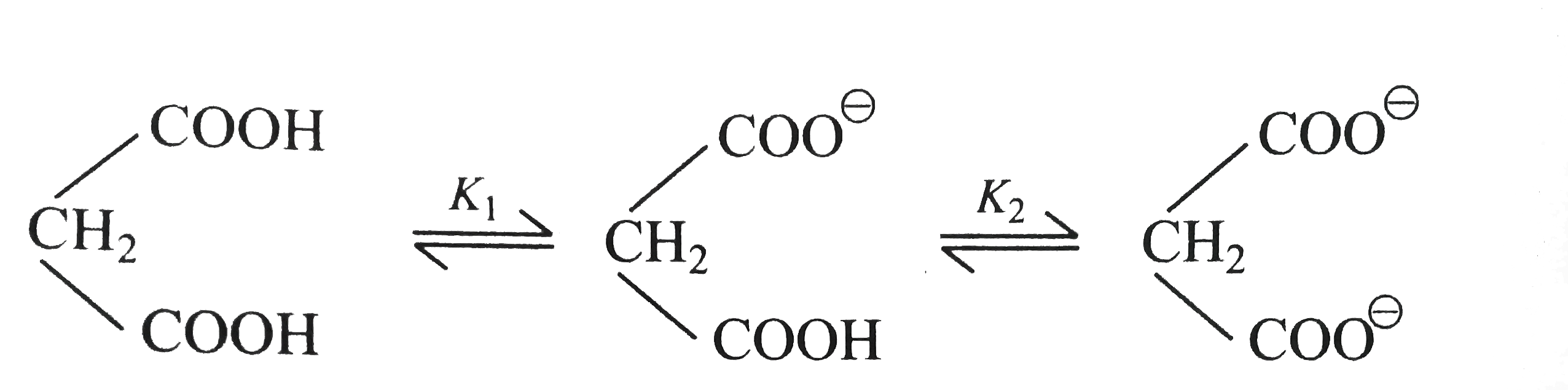
a. Consider malonic acid to be $H_{2} A$ , where malonate ions is $A^{2 -}$ . For dibasic acid, we consider ionisation in two stages as follows:
$(H_{2} A \Leftrightarrow, H^{\oplus} +, H A^{Θ},, first ionisation constant,), (C - x, x, x, = K_{1} = 1.42 \times 10^{- 3},), (,,, C = initial concentration,), (,,, = 0.001 M,)$
$\Rightarrow K_{1} = \frac{[H^{\oplus}] [H A^{Θ}]}{[H_{2} A]} = \frac{x^{2}}{C - x} = 1.42 \times 10^{- 3}$
Solving the quadratic,
$x^{2} + 1.42 \times 10^{- 3} x - 1.42 \times 10^{- 6} = 0$
$\Rightarrow x = 6.75 \times 10^{- 4} M$
Note that $K_{1} > > K_{2}$ so concentration of $H^{\oplus}$ in solution is considered only from first dissociation, i.e., $[H^{\oplus}] = x M$ neglect $[H^{\oplus}]$ concentration from second ionsiation.
Consider second ionisation:
$(H A^{Θ} \to, H^{\oplus} +, A^{2 -},, Second ionisation = K_{2},), (x, x, -, = 2.0 \times 10^{- 6},), (x - y, x + y, y,,)$
$\Rightarrow K_{2} = \frac{[H^{\oplus}] [A^{2 -}]}{[H A^{Θ}]} = \frac{(x + y) y}{x - y}$
Note: $[H^{\oplus}] = x + y \approx x [H A^{\oplus} = x - y = x (y < < x)$
$\Rightarrow K_{2} = y [A^{2 -}] = 2.0 \times 10^{- 6} M$
Note: Ustually, $[A^{2 -}]$ for $H_{2} A$ (dibasic acid) can be aproximately taken to be $K_{2}$ .
b. Hence $C = 10^{- 4} M$ ,
$[H^{\oplus}] = 4 \times 10^{- 4} M$ (from $H C 1)$
$\Rightarrow K_{1} = \frac{[H^{\oplus}] [H A^{Θ}]}{[H_{2} A]} = \frac{(4 \times 10^{- 4} + x) (x)}{10^{- 4} - x}$
$= 1.42 \times 10^{- 3}$
Importane: Note that, we can not go for approximation in this case, since the concentration of $H C 1$ is so low that $H^{\oplus}$ is considered both from $H C 1$ and malonic acid. Solving the quadratic.
$x^{2} + 1.82 \times 10^{- 3} x - 1.42 x 10^{- 7} = 0$
$\Rightarrow x = 7.5 \times 10^{- 5} M$
Now consider, second ionisation and substitute for value of $x$ .
$K_{2} = \frac{[H^{\oplus}] [A^{2 -}]}{[H A^{Θ}]} = \frac{(4 \times 10^{- 4} + x) (y)}{x} = 2.0 \times 10^{- 6}$
$\Rightarrow \frac{(4 \times 10^{- 4} + 7.5 \times 10^{- 5}) y}{7.5 \times 10^{- 5}} = 2.0 \times 10^{- 6}$
$\Rightarrow y = [A^{2 -}] = 3.2 \times 10^{- 7} M$
c. Here $C = 10^{- 4} M, [H^{\oplus}] = 0.1 M$
$\Rightarrow K_{a} = K_{1} K_{2} = \frac{{[H^{\oplus}]}^{2} [A^{2 -}]}{[H_{2} A]} = \frac{{(0.1)}^{2} [A^{2 -}]}{10^{- 4}}$
$= 2.84 \times 10^{- 9}$
Important: Note thate, we will do the approximation in this case, since the concentration of $H C 1$ is so high that $H^{\oplus}$ considered from malonic acid is negligible. Recall the ionisation of $H_{2} S (g)$ in an acidic solution. Solving the equation, we get: $[A^{2 -}] = 2.84 \times 10^{- 11} M$

Updated on:21/07/2023