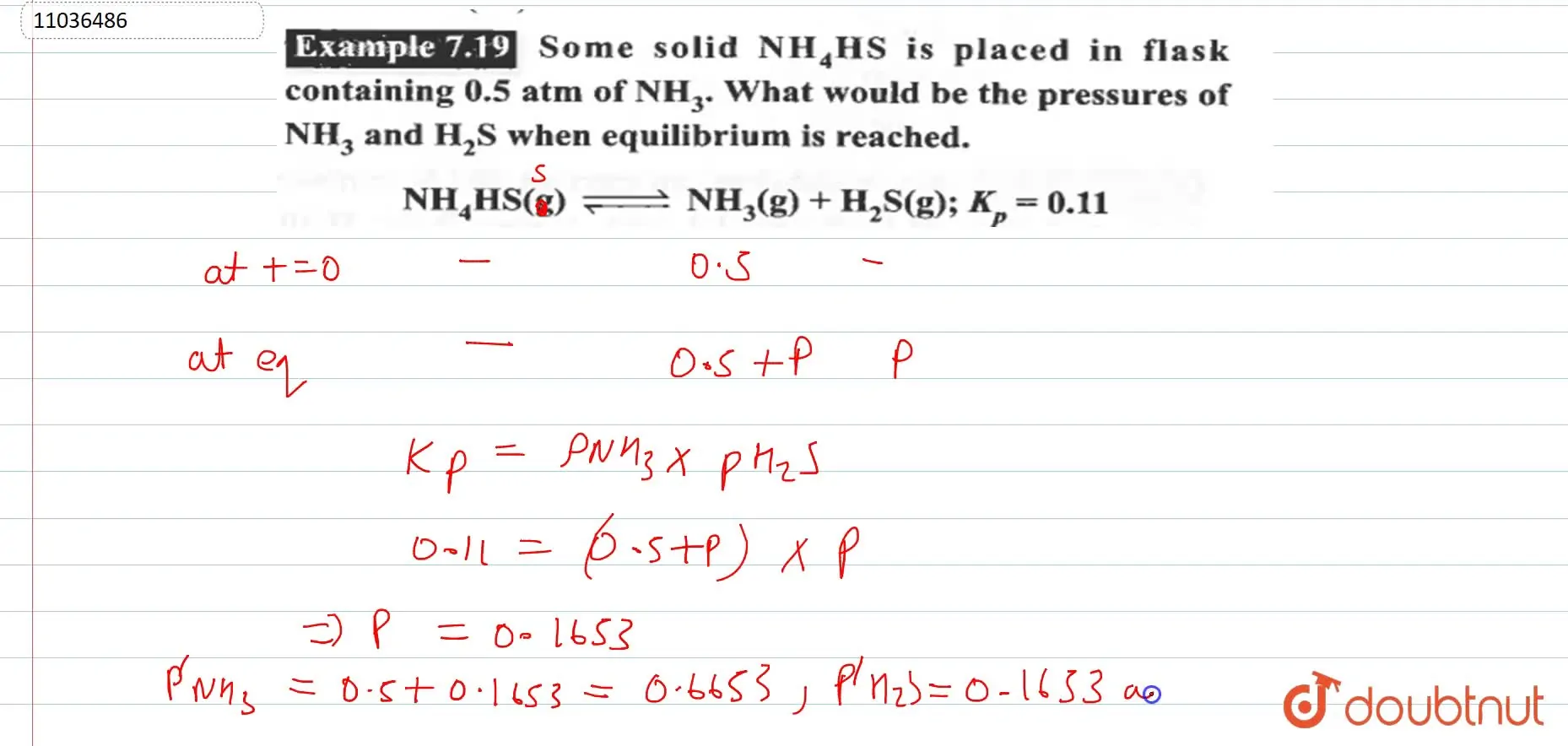
Some solid NH4HS is placed in flask containing 0.5 atm of NH3. What would be the pressure of NH3 and H2S when equilibrium is reached.
NH4HS(g)⇔NH3(g)+H2S(g),Kp=0.11
NH4HS(g)⇐⇒NH3(g)+H2S(g)Pressure before dissociation0.50Pressure after dissociation(0.5+P)P
Let pressure equivalent to P atm is developed by NH3 and H2S on dissociation of NH4HS, when 0.5atm of NH3 is already present.
∴Kp=P'NH3×P'H2S=(0.5+P)(P)
or 0.11=(0.5+P)(P)
∴P=0.1653
∴P'NH3=0.5+0.1653=0.6653atm
∴P'H2S=0.1653atm
Related Playlists
- BOOK - CENGAGE CHEMISTRYCHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - Concept Applicationexercise 7.153videos
CHEMICAL EQUILIBRIUM
- BOOK - CENGAGE CHEMISTRYCHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - Ex 7.240videos
CHEMICAL EQUILIBRIUM
- BOOK - CENGAGE CHEMISTRYCHAPTER - CHEMICAL BONDING AND MOLECULAR STRUCTUREEXERCISE - Archives Subjective15videos
CHEMICAL BONDING AND MOLECULAR STRUCTURE
- BOOK - CENGAGE CHEMISTRYCHAPTER - CLASSIFICATION AND NOMENCLATURE OF ORGANIC COMPOUNDSEXERCISE - Analytical and Descriptive Type3videos
CLASSIFICATION AND NOMENCLATURE OF ORGANIC COMPOUNDS
Similar Questions
Some acid NH4HS is placed in flask containing 0.5atm of NH3. What would be pressures of NH3 and H2S when equilibrium is reached?
NH4HS(s)⇔NH3(g)+H2S(g), Kp=0.11View SolutionSome acid NH4HS is placed in flask containing 0.5atm of NH3. What would be pressures of NH3 and H2S when equilibrium is reached?
NH4HS(s)⇔NH3(g)+H2S(g), Kp=0.11View SolutionSome solid NH4HS is placed in flask containing 0.5 atm of NH3. What would be the pressure of NH3 and H2S when equilibrium is reached.
NH4HS(g)⇔NH3(g)+H2S(g),Kp=0.11View SolutionOn adding NH4HS in following equilibrium NH4HS(s) ↔ NH3(g)+H2S(g)
View SolutionConsider the decomposition of solid NH4HS in a flask containing NH3(g) at a pressure of 2 atm. What will be the partial pressure of NH3(g)andH2S(g) after the equilibrium has been attained?
Kp for the reaction is 3.View Solution" The equilibrium constant "Kp" for the reaction NH4HS(s)→NH3(g)+H2S(g) is
View SolutionThe equilibrium constant Kp for the reaction NH4HS(s)⇔NH3(g)+H2S(g) is
View SolutionWhich of the following statement(s) is/are correct for the following equilibrium?
NH4HS(s)⇔NH3(g)+H2(g)View SolutionA definite amount of solid NH4HS is placed in a flask already containing ammonia gas at a certain temperature and 0.1 atm pressure. NH4HS decompses to give NH3 and H2S and at equilibrium total pressure in flask is 1.1 atm. If the equilibrium constant KP for the reaction NH4HS(s)⇔NH3(g)+H2S(g) is represented as z×10−1 then find the value of z.
View SolutionOne "mole" of NH4HS(s) was allowed to decompose in a 1−L container at 200∘C. It decomposes reversibly to NH3(g) and H2S(g).NH3(g) further undergoes decomposition to form N2(g) and H2(g). Finally, when equilibrium was set up, the ratio between the number of moles of NH3(g) and H2(g) was found to be 3.
NH4HS(s)⇔NH3(g)+H2S(g),Kc=8.91×10−2M2
2NH3(g)⇔N2(g)+3H2(g),Kc=3×10−4M2
Answer the following:
Assuming the volume due to solid NH4HS is negligible what will be the density of the gaseous mixture in the above equilibrium system?View Solution