If sum of two unit vectors is a unit vector; prove that the magnitude of their difference is $\sqrt{3}$

Video Solution

Text Solution

Verified by Experts

Let $ˆ a$ and $ˆ b$ be two unit vectors represented by sides OA and AB of a $Δ O A B$ .

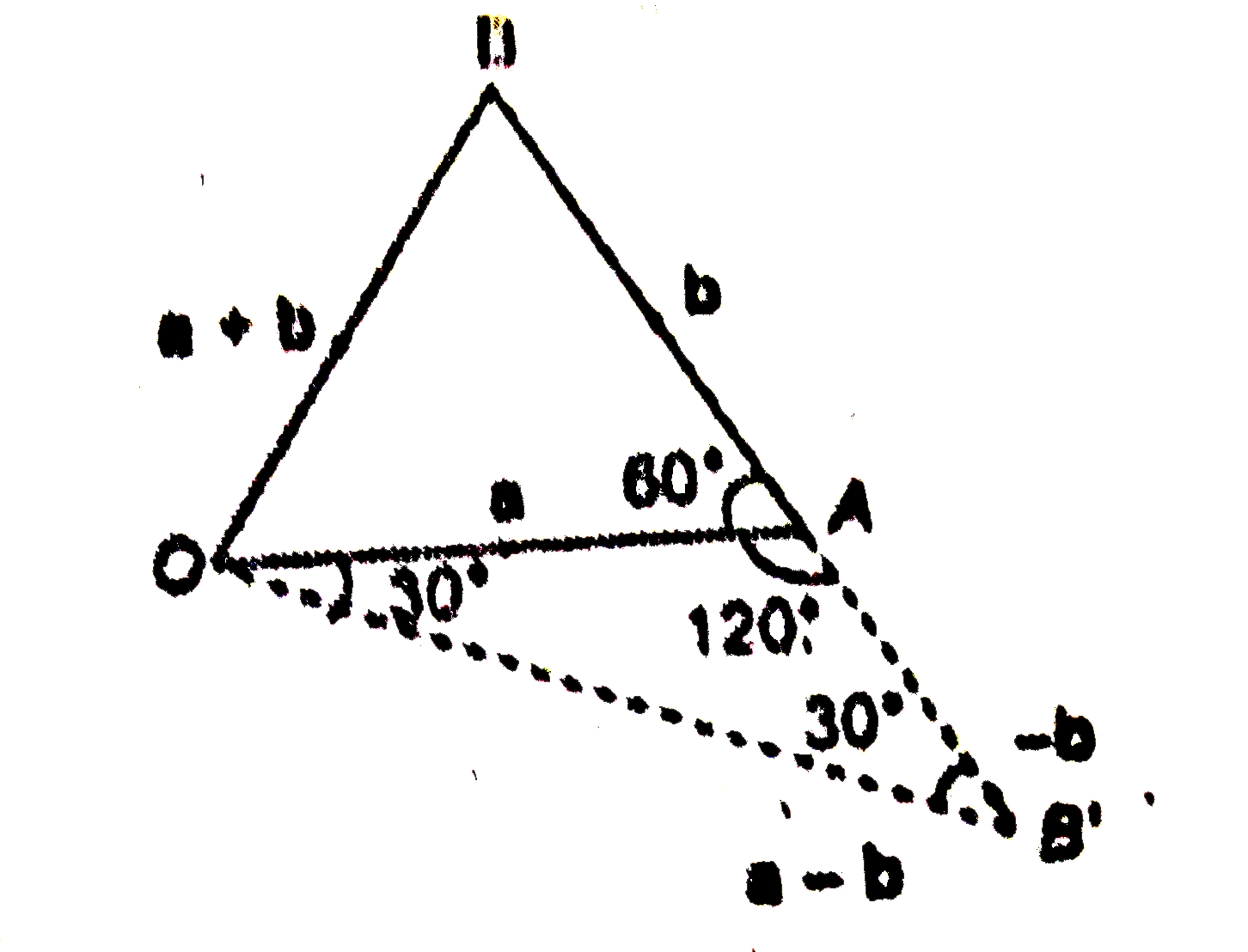
Then, $O A = ˆ a, A B = ˆ b$
$O B = O A + A B = ˆ a + ˆ b$ (using triangle law of vector addition)
It is given that, $| ˆ a | = ∣ ∣ ˆ b ∣ ∣ = ∣ ∣ ˆ a + ˆ b ∣ ∣ = 1$
$\Rightarrow | O A | + | A B | = | O B | = 1$
$Δ O A B$ is equilateral triangle.
Since, $| O A | = | ˆ a | = 1 = | = | - ˆ b | = | A B' ∣ ∣$
therefore, $Δ O A B'$ is a isosceles triangle.
$\Rightarrow ∠ A B' O = ∠ A O B' = 30^{\circ}$
$\Rightarrow ∠ B O B' = ∠ B O A + ∠ A O B' = 60^{\circ} + 30^{\circ} = 90^{\circ}$ (since, $Δ B O B'$ is right angled)
$∴$ In $Δ B O B'$ , we have
$| B B' | = {| O B |}^{2} + {| O B' |}^{2}$
$= {∣ ∣ ˆ a + ˆ b ∣ ∣}^{2} + {∣ ∣ ˆ a - ˆ b ∣ ∣}^{2}$
$2^{2} = 1^{2} + {∣ ∣ ˆ a - ˆ b ∣ ∣}^{2}$
$ˆ a - ˆ b ∣ = \sqrt{3}$ . . . hence proved.

Updated on:21/07/2023

If the sum of two unit vectors is a unit vector,then find the magnitude of their differences.
A $\sqrt{2}$
B $\sqrt{3}$
C $\sqrt{5}$
D $\sqrt{7}$
If the sum of two unit vectors is a unit vector, then the magnitude of their difference is
A $\sqrt{2} u n i t s$
B2 units
C $\sqrt{3} u n i t s$
D $\sqrt{5} u n i t s$
If the sum of two unit vectors is a unit vector, then the magnitude of their diffierence is
A $\sqrt{2}$
B $\sqrt{3}$
C $\sqrt{5}$
D $\sqrt{7}$