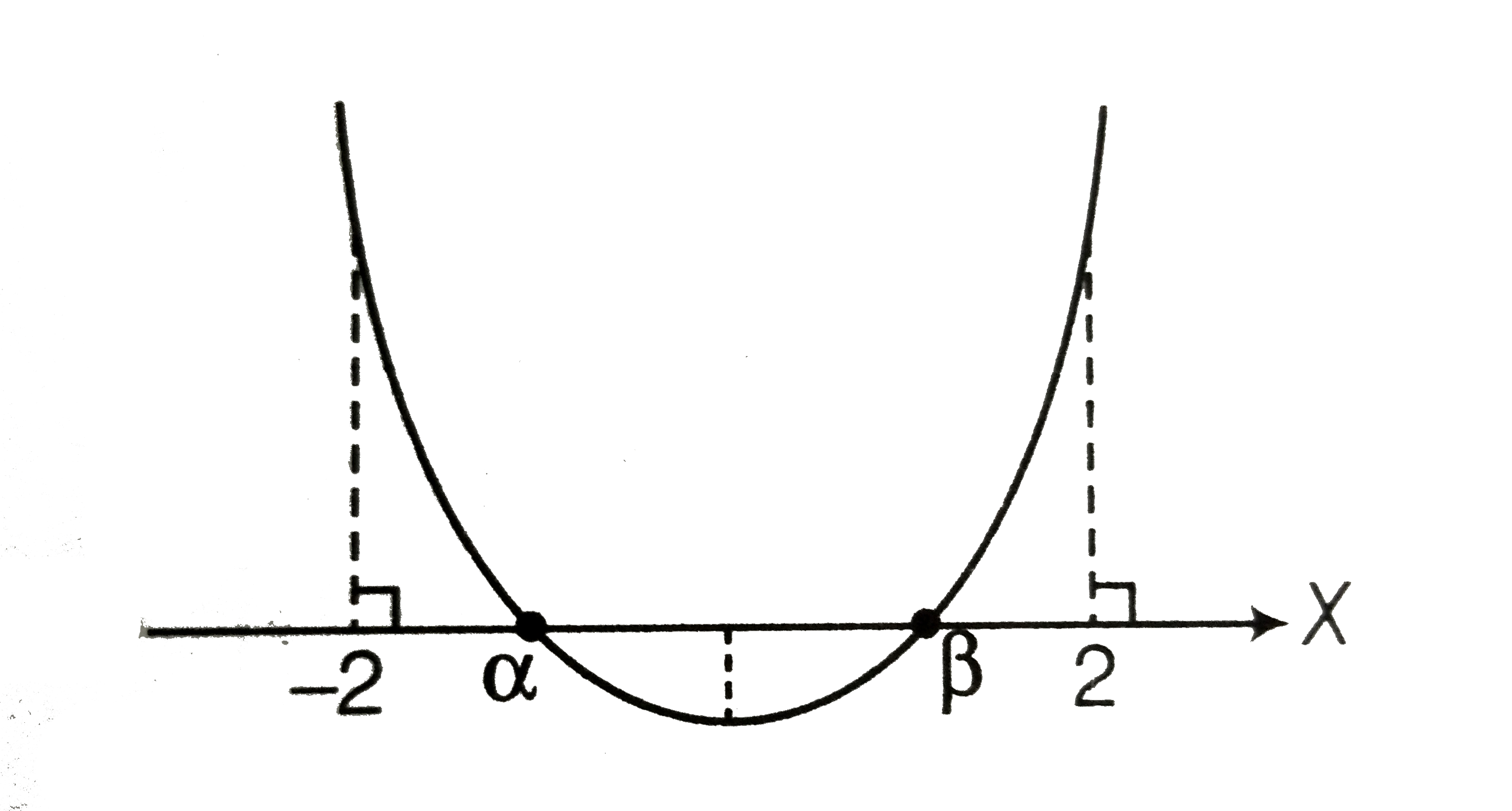
If both roots of the equation $x^{2} - 2 a x + a^{2} - 1 = 0$ lie between $(- 2, 2)$ then a lies in the interval

A

$R$

B

$(- 1, 1)$

C

$(- 2, 2)$

D

$(- 3, - 1) \cup (1, 3)$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Let $f (x) = x^{2} - 2 a x + a^{2} - 1$
Now four cases arise:
Case I $D \geq 0$

$\Rightarrow {(- 2 a)}^{2} - 4.1 (a^{2} - 1) \geq 0$
$\Rightarrow 4 \geq 0$ ltbr>gt $∴ a ε R$
Case II $f (- 2) > 0$
$\Rightarrow 4 + 4 a + a^{2} - 1 > 0$
$\Rightarrow a^{2} + 4 a + 3 > 0$
$\Rightarrow (a + 1) (a + 3) > 0$
$∴ a ε (- \infty, - 3) \cup (- 1, \infty)$
Case III $f (2) > 0$
$\Rightarrow 4 - 4 a + a^{2} - 1 > 0$
$\Rightarrow a^{2} - 4 a + 3 > 0$
$\Rightarrow (a - 1) (a - 3) > 0$
$∴ a ε (- \infty, 1) \cup (3, \infty)$
Case IV $- 2 < x$ coordinate of vertex $< 2$
$\Rightarrow - 2 < 2 a < 2$
$∴ a ε (- 1, 1)$
Hence $[a] = - 1, 0$

|

Updated on:21/07/2023

Class 12 MATHS QUADRATIC EQUATIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One or More
If both roots of the equation $x^{2} - 2 a x + a^{2} - 1 = 0$ lie between -3 and 4 ,then [a] is/are , where [ ] represents the greatest ineger function
A1
B $- 1$
C2
D0
Question 2 - Select One
Find the valuesof $m$ for which exactly one root of the equation $x^{2} - 2 m x + m^{2} - 1 = 0$ lies in the interval $(- 2, 4)$
A $m > 3$
B $- 1 < m < 3$
C $1 < m < 4$
D $- 2 < m < 4$
Question 3 - Select One
If both the roots of the quadratic equation $x^{- 2} k x + k^{2} - 5 = 0$ are less than 5, then k lies in the
A $(- \infty, 4)$
B $[4, 5]$
C $(5, 6)$
D $(6, \infty)$

ARIHANT MATHS-THEORY OF EQUATIONS-Exercise (Single Option Correct Type Questions)

Home
Ask Doubt
Profile