निम्नलिखित सर्वसमिकाओं को सिद्ध कीजिए
(i)1+cot2θ1+cosecθ=cosecθ (ii)sec4θ−sec2θ=tan4θ+tan2θ
(iii)2sec2θ−sec4θ−2cosec2θ+cosec4θ =cot4θ−tan4θ
(i) L.H.S. =1+cot2θ1+cosecθ=1+cosec2θ−11+cosecθ
=1+(cosecθ+1)(cosecθ−1)1+cosecθ=1+cosecθ−1
=cosecθ=R.H.S.
(ii) L.H.S. =sec4θ−sec2θ=sec2θ(sec2θ−1)
=(1+tan2θ)tan2θ=tan2θ+tan4θ
=tan4θ+tan2θ=R.H.S.
(iii) L.H.S. =2sec2θ−sec4θ−2cosec2θ+cosec4θ
=2sec2θ−2cosec2θ−sec4θ+cosec4θ
=2(sec2θ−cosec2θ)−(sec4θ−cosec4θ)
=2(sec2θ−cosec2θ)−(sec2θ−cosec2θ)×(sec2θ+cosec2θ)
=(sec2θ−cosec2θ)(2−sec2θ−cosec2θ)
=(1+tan2θ−1−cot2θ)(2−1−tan2θ−1−cot2θ)
=(tan2θ−cot2θ)(−tan2θ−cot2θ)
=−(tan2θ−cot2θ)(tan2θ+cot2θ)
=−(tan4θ−cot4θ) ltBrgt =cot4θ−tan4θ=R.H.S.
Topper's Solved these Questions
- त्रिकोणमितीय अनुपात और सर्वसमिकाएँ BOOK - KC SINHACHAPTER - त्रिकोणमितीय अनुपात और सर्वसमिकाएँ EXERCISE - EXERCISE 8.1
65 Videos
- त्रिकोणमितीय अनुपात और सर्वसमिकाएँ BOOK - KC SINHACHAPTER - त्रिकोणमितीय अनुपात और सर्वसमिकाएँ EXERCISE - लघु उत्तरीय प्रश्न
87 Videos
- त्रिकोणमिति के कुछ अनुप्रयोग BOOK - KC SINHACHAPTER - त्रिकोणमिति के कुछ अनुप्रयोग EXERCISE - वस्तुनिष्ठ प्रश्न
14 Videos
- त्रिभुज BOOK - KC SINHACHAPTER - त्रिभुज EXERCISE - वस्तुनिष्ठ प्रश्न
28 Videos
Similar Questions
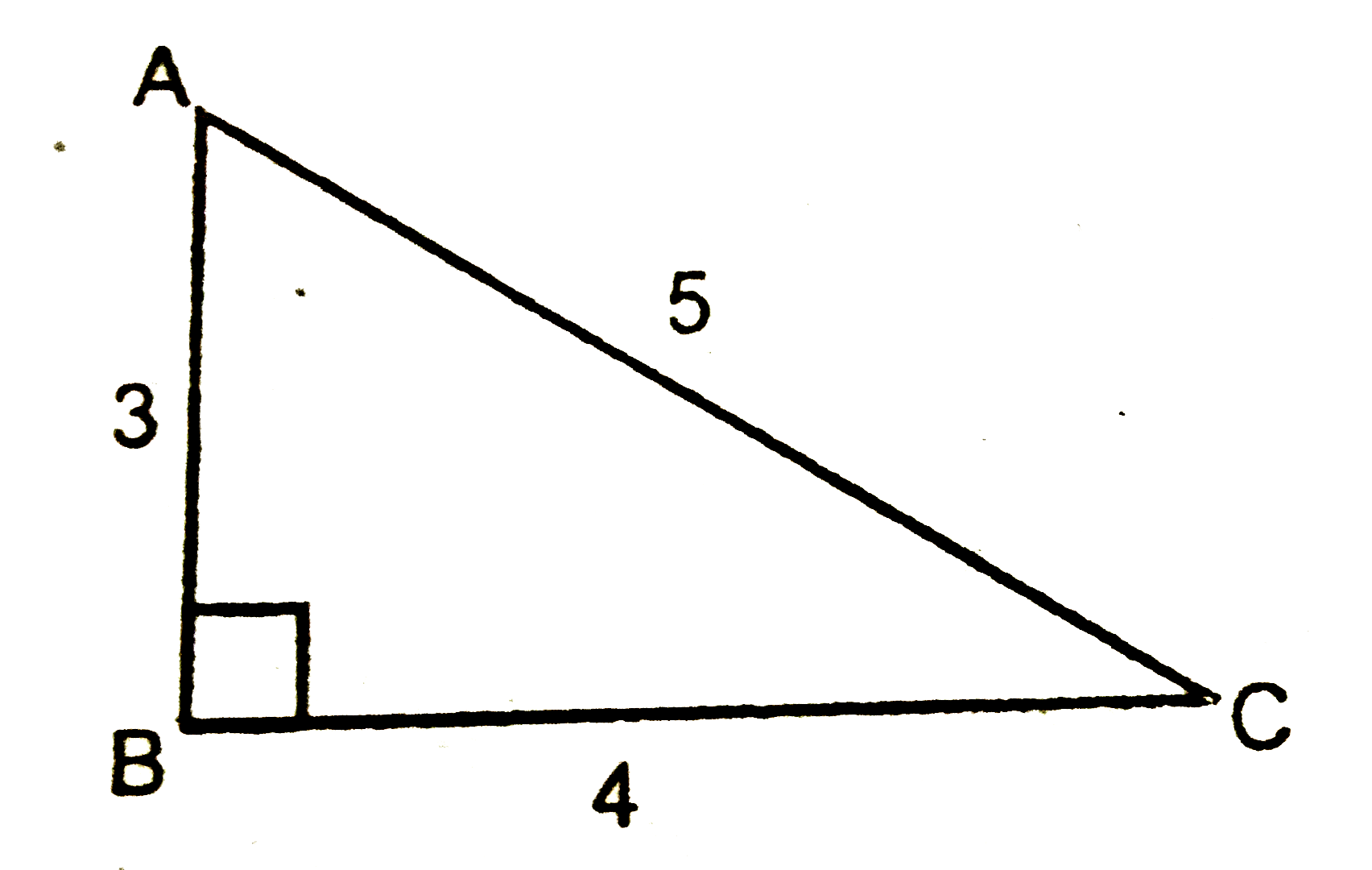
दिए गये चित्र से निम्नलिखित के मान ज्ञात कीजिए :
(i) sinC (ii)sinA
(iii) cosC (iv)cosA
(v) tanC (vi)tanA
View Solutionयदि tanA=ba,जहाँ a और b वास्तविक संख्याएँ है , तो sin2A का मान ज्ञात करें ।
View Solutionयदि एक मीनार के पाद से 100 m दूर स्थित बिंदु पर मीनार का उन्नयन कोण60∘ है , तो मीनार कि ऊँचाई है :
View SolutionΔABC में DE∣∣BC और ADDB=53 तब AEAC=
View Solution(sec theta+csc theta)^(2)-(tan theta+cot theta)^(2)=
View SolutionProve that 2sec^(2)theta-sec^(4)theta-2csc^(2)theta+csc^(4)theta=(1-tan^(8)theta)/(tan^(4)theta)
View Solution(sec theta+tan theta)/(csc theta+cot theta)=(csc theta-cot theta)/(sec theta-tan theta)
View SolutionProve each of the following identities :
(i)cosecθ+cotθcosecθ−cotθ=(cosecθ+cotθ)2=1+2cot2θ+2cosecθcotθ
(ii)secθ+tanθsecθ−tanθ=(secθ+tanθ)2=1+2tan2θ+2secθtanθ
View Solutionसिद्ध कीजिए 2sec2θ−sec4θ−2cosec2θ+cosec4θ=cot4θ−tan4θ.
View Solutionयदि tanθ=1√7, तब cosec2θ−sec2θcosec2θ+sec2θ
View Solutionयदि cotθ=1√7, तब सिद्ध कीजिए कि
cosec2θ−sec2θcosec2θ+sec2θ=−34.
View Solution(cosecθ−secθ)(cotθ−tanθ)=(cosecθ+secθ)(secθcosecθ−2)
View Solutionसिद्ध कीजिए कि : (secθ+cosec θ)(sinθ+cosθ)=tanθ+cotθ+2=2+secθcosecθ
View SolutionThe value of cosec2θcot2θ−sec2θtan2θ−(cot2θ−tan2θ)(sec2θcosec2θ−1) is-
View Solutioncosec2θ⋅cot2θ−sec2θ⋅tan2θ−(cot2θ−tan2θ)(sec2θ⋅cosec2θ−1)=
View Solution
