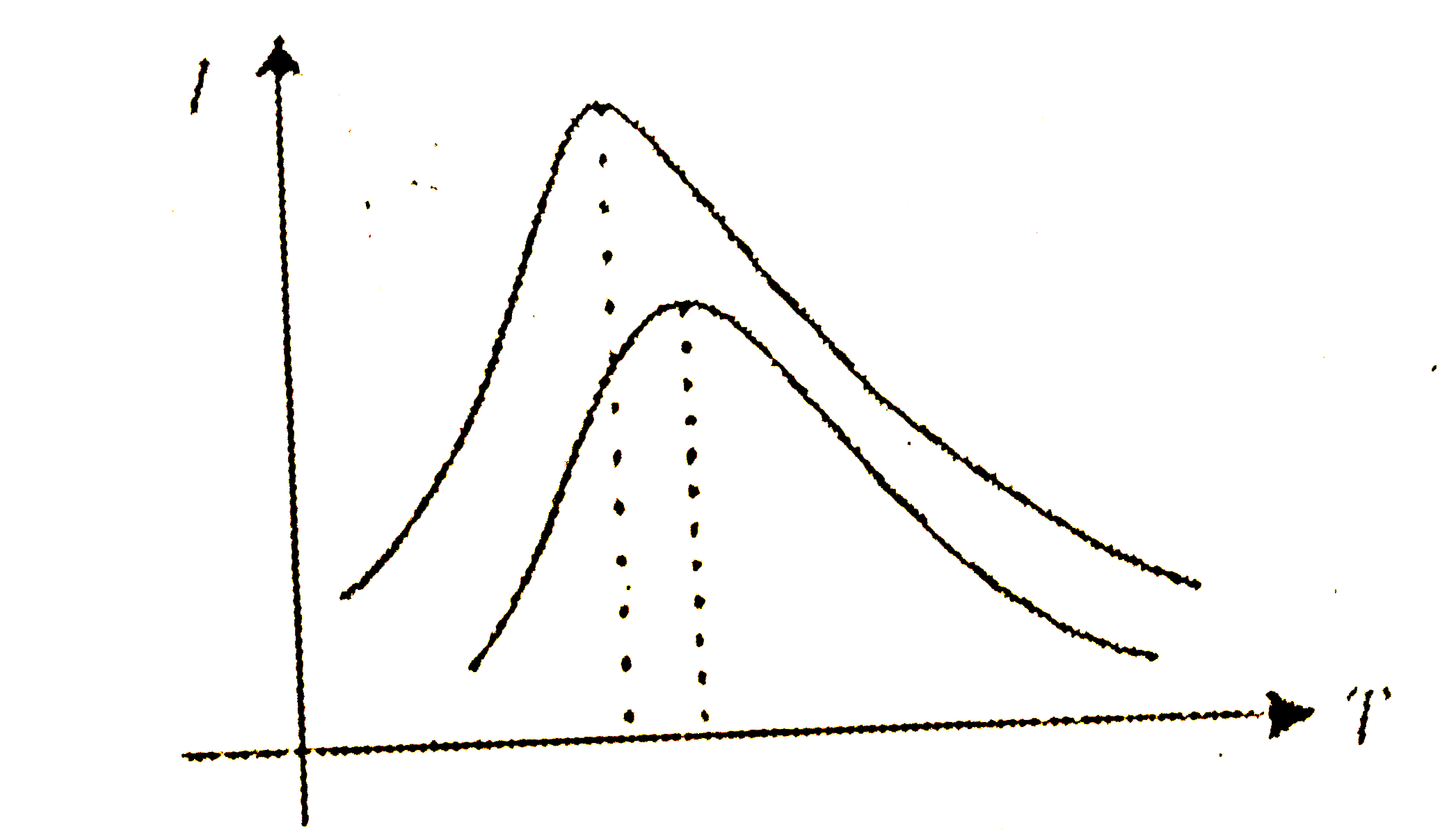
Assertion : For higher temperature, the peak emission wavelength of a black body shifts to lower wavelengths.
Reason : Peak emission wavelength of a black body is proportional to the fourth power of temperature.
If both assertion and reason are true and reason is the correct explanation of assertion
If both assertion and reason are true but reason is not the correct explanation of assertion
If assertion is true but reason is false
If both assertion and reason are false
The correct Answer is:B
Wien's relation given λ at maximum intensity, λm⋅T= constant.
Stefan's law,
σT4= intensity of emission of a particular wavelength does not explains the shift of λat with absolute temperature.
Topper's Solved these Questions
Similar Questions
Knowledge Check
This section contains Assertion and Reason type questions. Each question has 4 choices (1), (2), (3) and (4), out of which only one is correct.
Assertion (A) For higher temperatures, the peak emission wavelength of a black body shifts to lower wavelengths.
Reason (R) Peak emission wavelengths of a black body is proportional to the fourth-power of temperatureABoth Assertion and Reason are correct and Reason Is the correct explanation of the AssertionBBoth Assertion and Reason are correct but Reason Is not the correct explanation of AssertionCAssertion Is correct but Reason Is IncorrectDAssertion Is incorrect but Reason Is correctEmissivity of perectly black body is
A1B2C5D0The wavelength of maximum emission shifts towards smaller wavelengths as the temperature of black body
AincreasesBdecreasesCincreases or decreasesDremains constant
Statement-1: When the temperature of a black body is doubled fromt∘C to 2t∘C, the radiant power becomes 16 times.
Statement-2: The radiant power of a body is proportional to fourth power of absolute temperature.
View SolutionStatement I: As the temperature of the black body increases, the wavelength at which the spectral intensity (Eλ) is maximum decreases.
Statement II: The wavelength at which the spectral intensity will be maximum for a black body is proportional to the fourth power of its absolute temperature.
View SolutionIf the temperature of any ideal black body is halved, then the wavelength of maximum emission will be
View SolutionIf the temperature of a black body is increased then the wavelength corresponding to the maximum emission will
View SolutionAssertion : Coefficient of absorption of radiation of an ideal black body is 1.
Reason : An ideal black body emits radiation of all wavelengths.
View Solution
