There are four concentric shells A,B, C and D of radii $a, 2 a, 3 a$ and $4 a$ respectively. Shells B and D are given charges $+ q$ and $- q$ respectively. Shell C is now earthed. The potential difference $V_{A} - V_{C}$ is $k = (\frac{1}{4 π ε_{0}})$

A

$\frac{k q}{2 a}$

B

$\frac{k q}{4 a}$

C

$\frac{k q}{3 a}$

D

$\frac{k q}{6 a}$

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:D

To solve the problem, we need to find the potential difference $V_{A} - V_{C}$ given the conditions of the concentric shells. Let's break down the solution step by step.
Step 1: Understand the configuration
We have four concentric shells A, B, C, and D with radii $a, 2 a, 3 a,$ and $4 a$ respectively. Shell B has a charge of $+ q$ and shell D has a charge of $- q$ . Shell C is earthed, meaning its potential $V_{C} = 0$ .
Step 2: Determine the potential at shell C
Since shell C is earthed, we set its potential $V_{C} = 0$ . The potential at any point due to a charged shell can be calculated using the formula:
$V = \frac{k Q}{r}$
where $k = \frac{1}{4 π ϵ_{0}}$ , $Q$ is the charge, and $r$ is the distance from the center.
Step 3: Calculate the potential at shell A
The potential at shell A ( $V_{A}$ ) is influenced by the charges on shells B and D. The contributions to the potential at shell A from these shells can be calculated as follows:
1. Contribution from shell B (at $2 a$ ):
- Since shell A is inside shell B, the potential due to shell B at shell A is:
$V_{B} = \frac{k (+ q)}{2 a}$
2. Contribution from shell D (at $4 a$ ):
- Shell D is outside shell A, so the potential due to shell D at shell A is:
$V_{D} = \frac{k (- q)}{4 a}$
Step 4: Combine the potentials
Now we can combine the contributions to find the total potential at shell A:
$V_{A} = V_{B} + V_{D} = \frac{k q}{2 a} - \frac{k q}{4 a}$
Step 5: Simplify the expression for $V_{A}$
To combine the terms, we need a common denominator:
$V_{A} = \frac{2 k q}{4 a} - \frac{k q}{4 a} = \frac{(2 k q - k q)}{4 a} = \frac{k q}{4 a}$
Step 6: Calculate the potential difference $V_{A} - V_{C}$
Since $V_{C} = 0$ :
$V_{A} - V_{C} = V_{A} - 0 = V_{A} = \frac{k q}{4 a}$
Step 7: Final result
Thus, the potential difference $V_{A} - V_{C}$ is:
$V_{A} - V_{C} = \frac{k q}{4 a}$
Summary
The potential difference between shell A and shell C is given by:
$V_{A} - V_{C} = \frac{k q}{4 a}$

Updated on:7/8/2024

|

Class 12 PHYSICS ELECTRIC CHARGES AND FIELDS

Topper's Solved these Questions

Similar Questions

Knowledge Check

There are four concentric shells A,B, C and D of radii $a, 2 a, 3 a$ and $4 a$ respectively. Shells B and D are given charges $+ q$ and $- q$ respectively. Shell C is now earthed. The potential difference $V_{A} - V_{C}$ is $k = (\frac{1}{4 π ε_{0}})$
A $\frac{K q}{2 a}$
B $\frac{k K q}{3 a}$
C $\frac{K q}{4 a}$
D $\frac{k q}{6 a}$
There are four concentric spherical shells A, B, C and D of radii R, 2R, 3R and 4R respectively. Net charge on all the spherical shells are same and equal to Q. If $V_{A}, V_{B}, V_{C}) and V_{D}$ represent electric potentials of A, B, C and D respectively, the magnitude of $V_{D} = V$ .

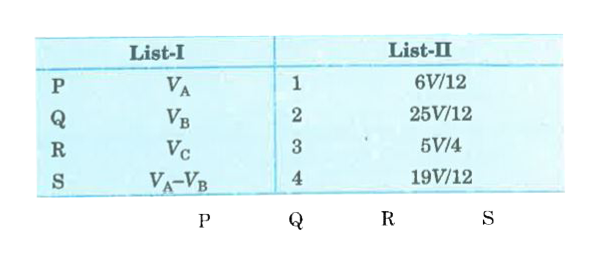A $\begin{matrix} P & Q & R & S 1 & 3 & 2 & 4 \end{matrix}$
B $\begin{matrix} P & Q & R & S 1 & 2 & 4 & 3 \end{matrix}$
C $\begin{matrix} P & Q & R & S 2 & 4 & 3 & 1 \end{matrix}$
D $\begin{matrix} P & Q & R & S 4 & 2 & 3 & 1 \end{matrix}$
Two concentric spherical conducting shells of radii 'a' and 2a are initially given charges +2Q and +Q respectively as shown. The total heat dissipated after switch 'S' has been closed is (where $k = \frac{1}{4 π ε_{0}}$ )

A $\frac{5 k Q^{2}}{4 a}$
B $\frac{k Q^{2}}{a}$
C $\frac{k Q^{2}}{2 a}$
D $\frac{k Q^{2}}{4 a}$

DC PANDEY-ELECTROSTATICS-Level 1 Subjective

Home
Ask Doubt
Profile