A battery has an open circuit potential difference of 6V between its terminals. When a load resistance of 60Ω is connected across the battery,the total power supplied by the bttery is 0.4W. What should be the load resistance R, so that maximum power will be dissipated in R. Calculated this power. What is the total power supplied by the battery when such a load is connected?
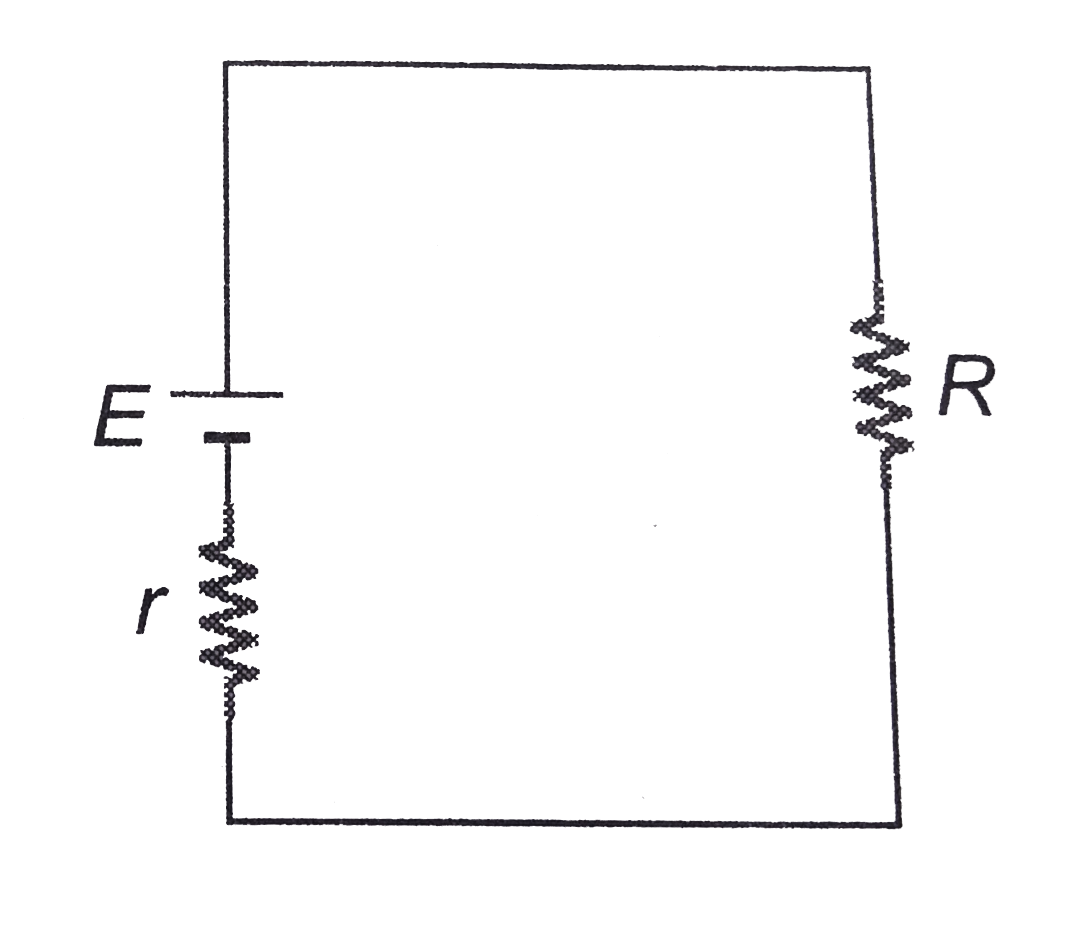
Whe the circuit is open V=E
∴E=6V
Let r be the internal resistance of the battery
Power supplied by the battery in this case is
P=E2R+r
Subsituting the value, we have 0.4=(6)260+r
Solving this we get r=30Ω
Maximum power is dissipated in the circuit when net external resistance is equal to net internal resistance or
R=r
∴R=30Ω
further, total power supplied by the battery under this condition is
Ptotal=E2R+r=(6)230+30
=0.6W
of this 0.6 W half of the power is dissipated in R and half in r. Therefore, maximum power dissipated in R would be
0.62=0.3W
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - DC PANDEYCHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise 23.1
6 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEYCHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise 23.2
1 Video
- CURRENT ELECTRICITYBOOK - DC PANDEYCHAPTER - CURRENT ELECTRICITYEXERCISE - Example Type 6
1 Video
- COMMUNICATION SYSTEMBOOK - DC PANDEYCHAPTER - COMMUNICATION SYSTEMEXERCISE - Subjective
11 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrances gallery
25 Videos
Similar Questions
The potential differnce between the terminals of a battery of emf 6.0 V and internal resistance 1Ω drops to 5.8 V when connected across an external resistor . Find the resistance of the external res
View SolutionA battery of emf 10 V is connected with a load resistance of 50 Omega the total power delivered by the battery is 2 W. If load resistance is replaced by R then power delivered is 4 W, the value of R is
View Solution
Knowledge Check
A battery has an open circuit potential difference 10 V between the terminals. When loads 9Ωand4Ω are connected one by one across the battery , the power in the load resistance is the same. The amount of heat approximately generated in one second in the load when a load of 5Ω Is connected across the battery will be
A10.4JB9 JC6.8JD4.13JIn the circuit shown, total power supplied by an ideal battery is 80 W. if R=10Ω. Then
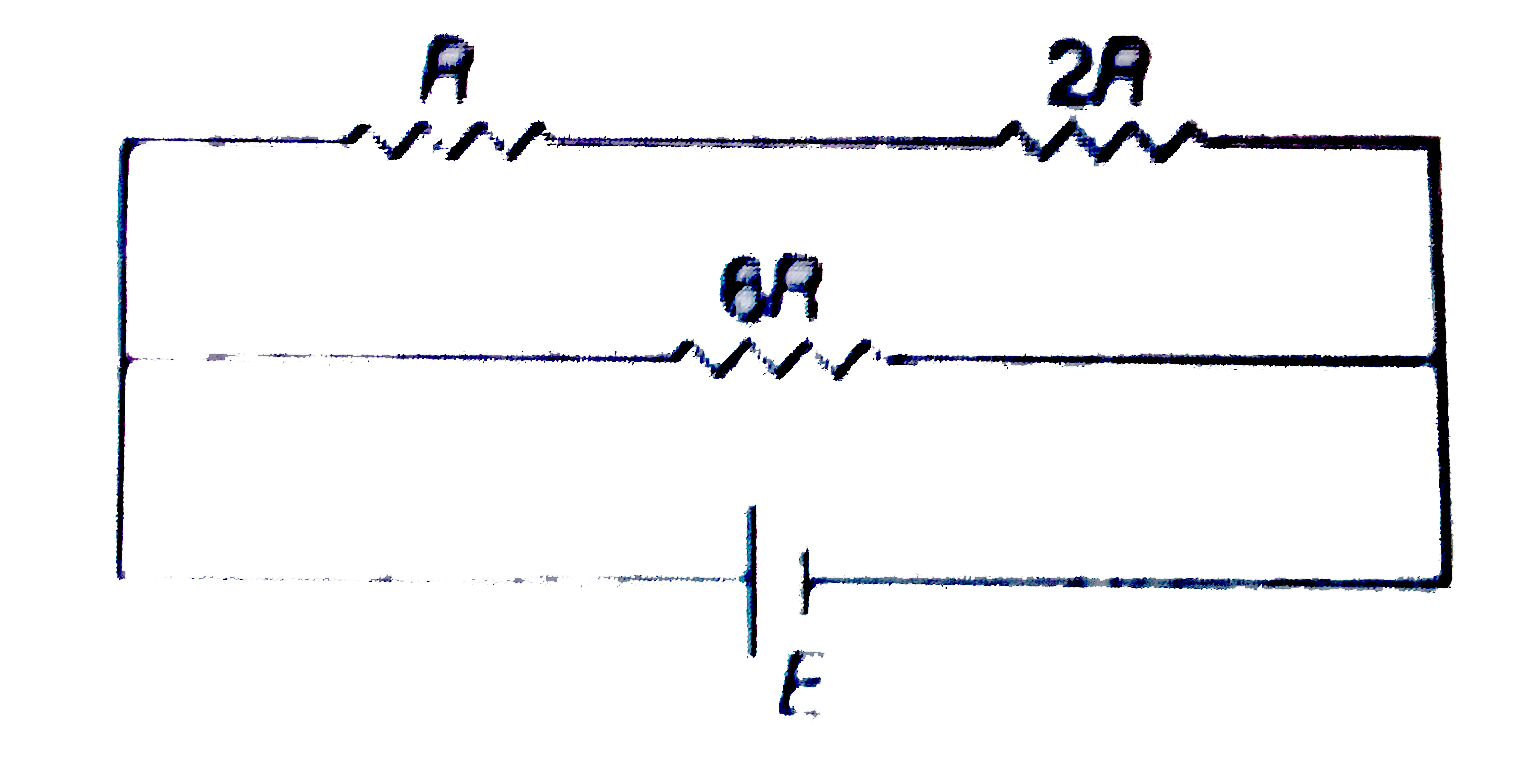
If the resistance R is removed from the circuit. ThenApower consumed by 2R will increaseBpower consumed by 6R will increaseCboth (a) and (b) are correctDboth (a) and (b) wrongIn the circuit shown, total power supplied by an ideal battery is 80 W. if R=10Ω. Then
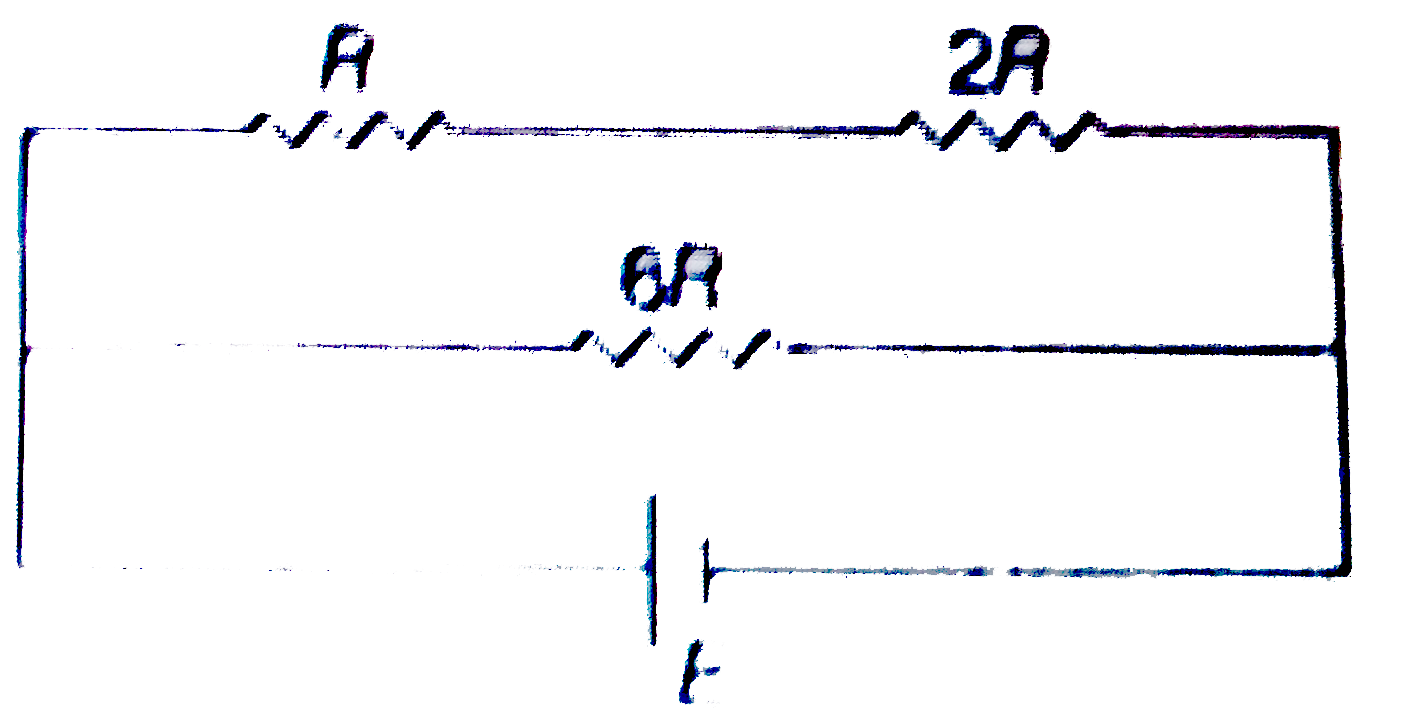
EMF of the battery isA20 VB10 VC40 VD60 V
When a resistance of 2Ω is placed across the terminals of a battery, the current is 0.5 A. When the resistance across the terminals of the battery is 5Ω, the current is 0.25 A. Calculate the emf of the battery and also its internal resistance.
View SolutionIn the circuit shown power dissipated in 12Ωresistance is 6 watt. What is the power dissipated in the 8Ω resistance.
View SolutionA battery has an emf E and internal resistance r. A variable resistance R is connected across the terminals of the battery. Find the value of R such that (a) the current in the circuit id maximum (b) the potential difference across the terminals is maximum.
View SolutionIn the circuit shown, total power supplied by an ideal battery is 80 W. if R=10Ω. Then
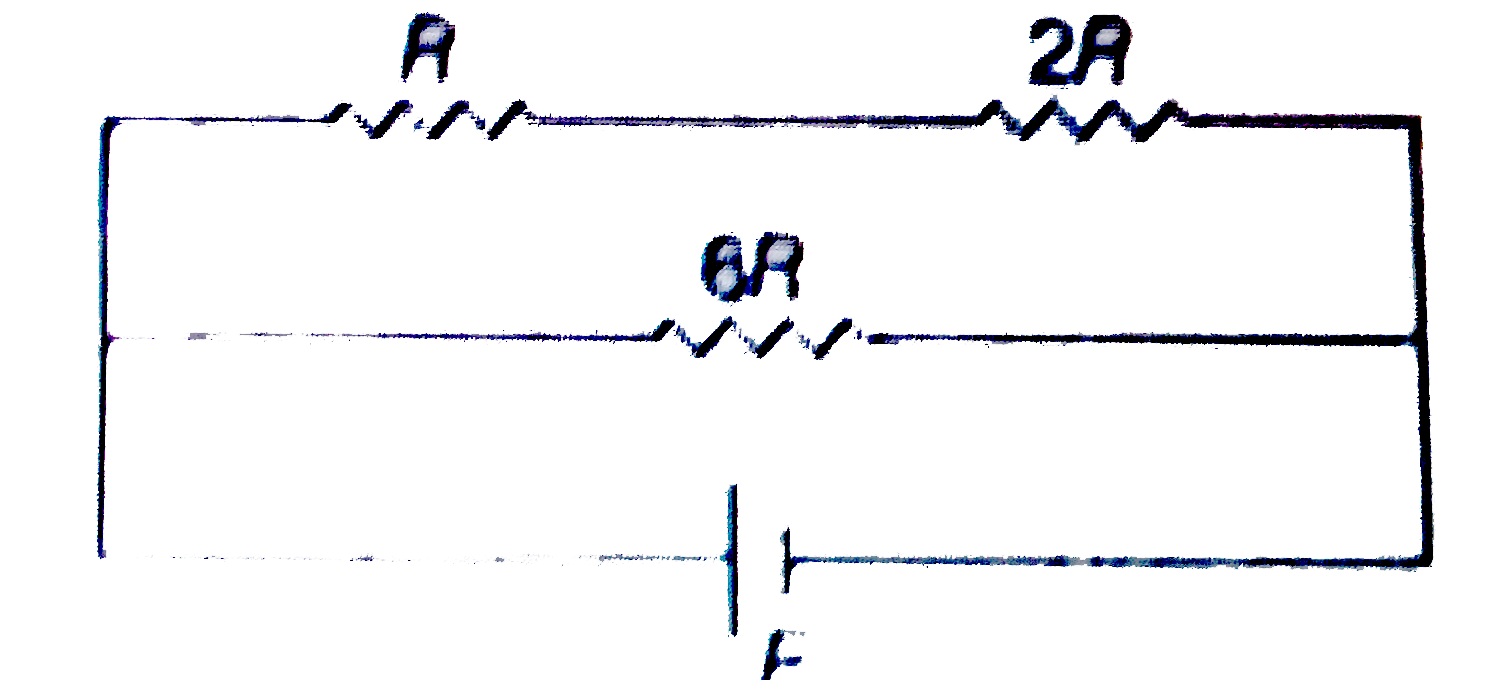
Ratio of power developed across R, 2R and 6R will be
View SolutionThe power dissipated across resistance R which is connected across a battery of potential V is P. If resistance is doubled, then the power becomes
View Solution