Three discs, A, B and C having radii 2m, 4m and6m respectively are coated with carbon black on their outer surfaces. The wavelengths corresponding to maximum intensity are and , respectively. The power radiated by them are respectively
(a) is maximum (b) is maximum (c) is maximum (d)
The correct Answer is:A
To solve the problem, we need to determine the power radiated by the three discs A, B, and C, which are treated as black bodies. The power radiated by a black body is given by the Stefan-Boltzmann law, which states that the power P radiated by a black body is proportional to the fourth power of its temperature T and its surface area A. The relevant formula for power can be expressed as:
P∝AT4
Since the discs are coated with carbon black, we can assume they behave like ideal black bodies. The area A of a disc is given by:
A=πr2
Where r is the radius of the disc. The wavelength corresponding to maximum intensity λm is given for each disc. According to Wien's displacement law, the temperature T of a black body is inversely proportional to the wavelength of maximum intensity:
T∝1λm
Therefore, we can express the power radiated by each disc in terms of its radius and the wavelength corresponding to maximum intensity.
Step 1: Calculate the area of each disc
- For disc A (radius = 2m):
AA=π(22)=4πm2
- For disc B (radius = 4m):
AB=π(42)=16πm2
- For disc C (radius = 6m):
AC=π(62)=36πm2
Step 2: Calculate the temperature for each disc using Wien's law
- For disc A (λm=300nm=300×10−9m):
TA∝1300×10−9⟹TA=k⋅1300×10−9
- For disc B (λm=400nm=400×10−9m):
TB∝1400×10−9⟹TB=k⋅1400×10−9
- For disc C (λm=500nm=500×10−9m):
TC∝1500×10−9⟹TC=k⋅1500×10−9
Step 3: Express the power for each disc
Using the formula P∝AT4, we can express the power for each disc:
- For disc A:
PA∝AAT4A=(4π)(k⋅1300×10−9)4
- For disc B:
PB∝ABT4B=(16π)(k⋅1400×10−9)4
- For disc C:
PC∝ACT4C=(36π)(k⋅1500×10−9)4
Step 4: Compare the powers
Now we can compare the ratios of the powers:
PAPB=4π(1300×10−9)416π(1400×10−9)4
PBPC=16π(1400×10−9)436π(1500×10−9)4
Calculating these ratios will show which disc radiates the most power.
Conclusion
After evaluating the ratios, we find that disc A radiates the maximum power due to its smaller wavelength corresponding to maximum intensity, which results in a higher temperature and thus more power radiated.
Final Answer
The correct option is (a) QA is maximum.
- For disc A (radius = 2m):
AA=π(22)=4πm2
- For disc B (radius = 4m):
AB=π(42)=16πm2
AC=π(62)=36πm2
- For disc A (λm=300nm=300×10−9m):
TA∝1300×10−9⟹TA=k⋅1300×10−9
- For disc B (λm=400nm=400×10−9m):
TB∝1400×10−9⟹TB=k⋅1400×10−9
TC∝1500×10−9⟹TC=k⋅1500×10−9
Using the formula P∝AT4, we can express the power for each disc:
- For disc A:
PA∝AAT4A=(4π)(k⋅1300×10−9)4
PB∝ABT4B=(16π)(k⋅1400×10−9)4
PC∝ACT4C=(36π)(k⋅1500×10−9)4
Now we can compare the ratios of the powers:
PAPB=4π(1300×10−9)416π(1400×10−9)4
PBPC=16π(1400×10−9)436π(1500×10−9)4
After evaluating the ratios, we find that disc A radiates the maximum power due to its smaller wavelength corresponding to maximum intensity, which results in a higher temperature and thus more power radiated.
The correct option is (a) QA is maximum.
Topper's Solved these Questions
- CALORIMETRY & HEAT TRANSFERBook:DC PANDEYChapter:CALORIMETRY & HEAT TRANSFERExercise:Example Type 4
Explore 4 Videos
- CALORIMETRY & HEAT TRANSFERBook:DC PANDEYChapter:CALORIMETRY & HEAT TRANSFERExercise:Miscellaneous Examples
Explore 13 Videos
- CALORIMETRY & HEAT TRANSFERBook:DC PANDEYChapter:CALORIMETRY & HEAT TRANSFERExercise:Example Type 2
Explore 2 Videos
- BASIC MATHEMATICSBook:DC PANDEYChapter:BASIC MATHEMATICSExercise:Exercise
Explore 13 Videos
- CALORIMETRY AND HEAT TRANSFERBook:DC PANDEYChapter:CALORIMETRY AND HEAT TRANSFERExercise:Medical entrance s gallery
Explore 38 Videos
Similar Questions
Three discs A, B and C having radii 2 m, 4m, and 6 m, respectively are coated with carbon black on their outer surfaces. The wavelengths corresponding to maximum intensity are 3 m, 4 m and 5 m respectively. The power radiated by them are PA,PBandPC respectively. Find the ratio of powers emitted by the three discs.
View SolutionTwo spheres A and B having radii 3cm and 5cm respectively are coated with carbon black on their outer surfaces.The wavelengths of maximum Intensity of emission of radiation are 300nm and 500nmrespectively.Find the ratio of powers ((P_(A))/(P_(B))) radiated by them.
View Solution
Knowledge Check
Two spheres A and B having radii 3 cm and 5 cm respectively are coated with carbon black on their outer surfaces. The wavelengths of maximum intensity of emitted radiation are 300 nm and 500 nm respectively. If the powers radiated are QA and QB respectively, then QAQB is
A√53B53C(53)2D(53)4Three thin disc A, B, C of the same material are coated with carbon black radii of discs are 2cm, 4cm, & 6cm respectively. Wavelength corresponding to maximum intensity are 300nm, 500nm, & 600 nm respectively. Power radiated by the discs are QA,QB & QC
AQA is maximumBQB is maximumCQC is maximumDQA=QB=QCThree discs A,B and C having radii 2,4 and 6 cm respectively are coated with carbon black. Wavelength for maximum intensity for the three discs are 300,400 and 500 nm respectively. If QA,QBandQCare power emitted by A,B and D respectively, then
AQA will be maximumBQB will be maximumCQC will be maximumDQA=QB=QC

Q. Identify the A,B,C and D.
View SolutionIn, the charges on C1,C2, and C3, are Q1,Q2, and Q3, respectively.
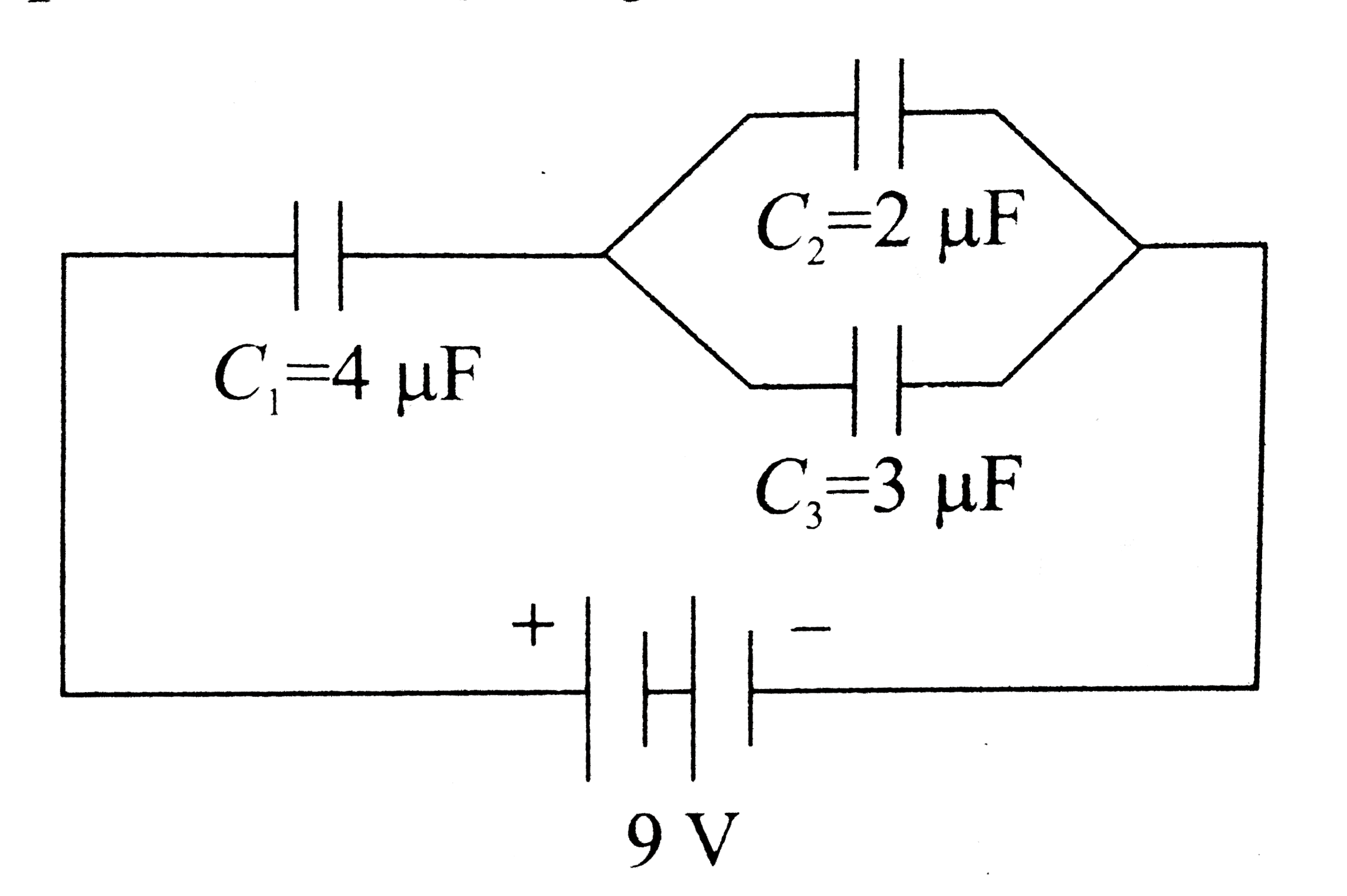 .
.
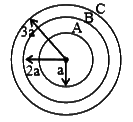
View SolutionThree concentric spherical shells A, B and C having uniformly distributed total charges +Q, -2Q and +Q respectively are placed as shown. The potential at the centre will be -
View SolutionA, B and C are the points (a, p), (b,q) and (c,r) respectively such that a, b and c are in AP and p,q and r in GP. If the points are collinear, then
View SolutionTwo small conductors A and B are given charges q1andq2 respectively. Now they are placed inside a hollow metallic conductor C carrying a charge Q. If all the three conductors A, B and C are connected by a conducting wire as shown, the charges on A,B and C will be respectively :

View Solution
