Asserton: In a standing wave x=0 is a node. Then, total mechanical energy lying between x=0andx=(lambda/8)is¬equal→thee≠rgyly∈gbetweenx=lambda/8andx=lambda/4.
Reason: In standing waves different particles oscillate with different amplitudes.
If both Assertion and Reason are true and the Reason is correct explanation of the Assertion.
If both Assertion and Reason are true but Reason is not the correct explanation of Assertion.
If Assertion is true, but the Reason is false.
If Assertion is false but the Reason is true.
The correct Answer is:A
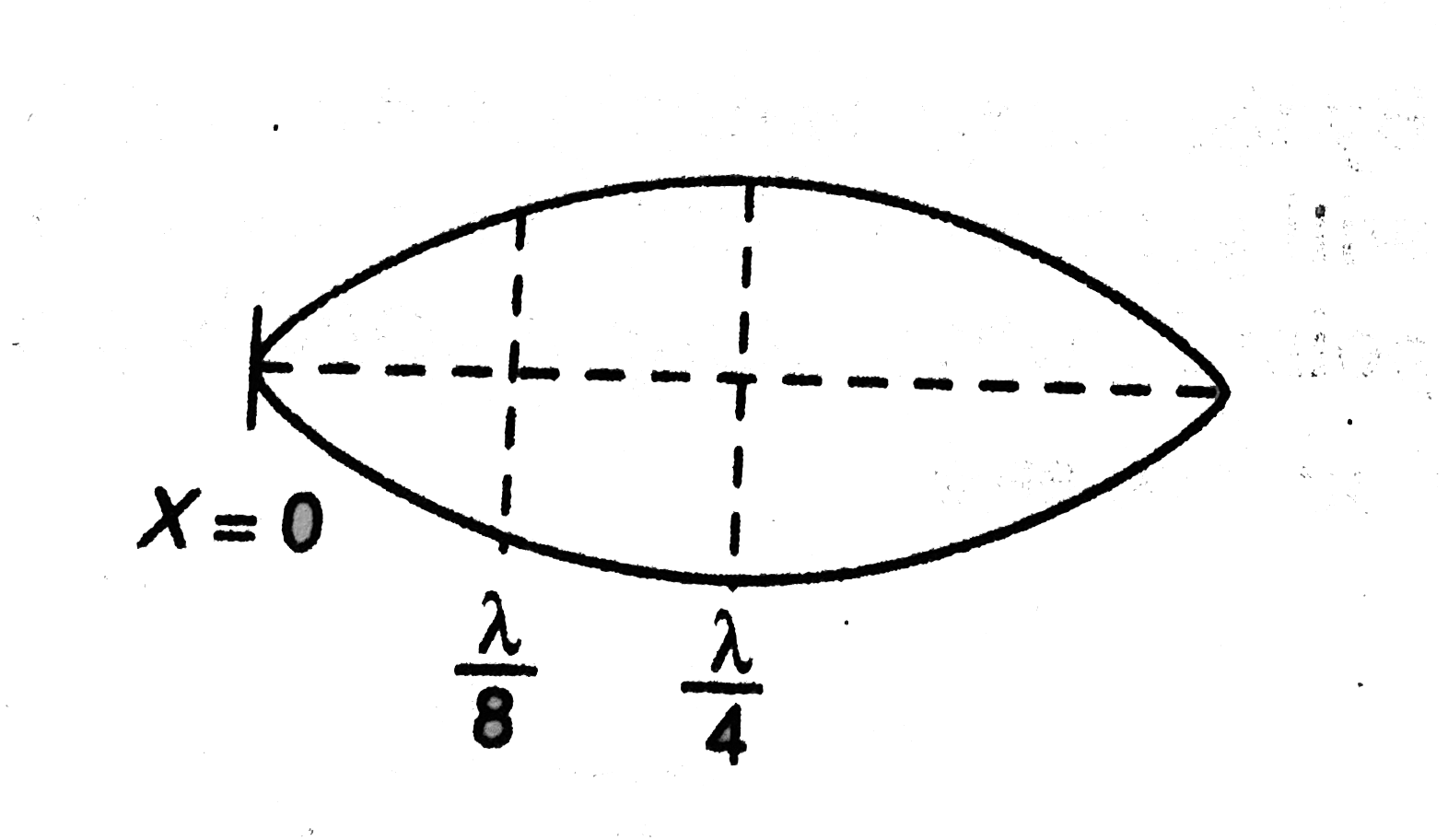
Energy lying between λ8 and λ4 will be more.
Topper's Solved these Questions
- SUPERPOSITION OF WAVESBOOK - DC PANDEYCHAPTER - SUPERPOSITION OF WAVESEXERCISE - Level 1 Objective
11 Videos
- SUPERPOSITION OF WAVESBOOK - DC PANDEYCHAPTER - SUPERPOSITION OF WAVESEXERCISE - Objective Questions
1 Videos
- SUPERPOSITION OF WAVESBOOK - DC PANDEYCHAPTER - SUPERPOSITION OF WAVESEXERCISE - Exercise 18.4
3 Videos
- SOUND WAVESBOOK - DC PANDEYCHAPTER - SOUND WAVESEXERCISE - Exercise 19.7
4 Videos
- THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESBOOK - DC PANDEYCHAPTER - THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESEXERCISE - Medical entrance gallary
30 Videos
Similar Questions
Knowledge Check
If path difference between is 11λ4 then the phase difference between two waves will be
A11π/2B5π/2C13π/2D7π/2The area between the curves x=4y−y2 and 0 is λ square units, then the value of 3λ is equal to
A28B30C32D36The angle between x2+y2−2x−2y+1=0 and line y=λx+1−λ, is
A0∘B45∘C30∘D90∘
If λ is a root of x2+3x+1=0 then tan−1λ+tan−1(1λ) is equal to
View SolutionIf the roots of the given equation 2x2+3(λ−2)x+λ+4=0 be equal in a magnitude but opposite in sign then λ
View SolutionA circle passes through a point (2,8) and touches the lines 4x−3y−24=0and4x+3y+42=0 and centre lies on the line x=λ then (−4λ) is
View SolutionThe circle x2+y2+2λx=0,λ∈R, touches the parabola y2=4x externally. Then, λ>0 (b) λ<0 λ>1 (d) none of these
View SolutionStatement I : In a standing wave on a string , the spacing between nodes is Δx. If the tension in string is increased wave same as before , then the separation between nearest node and antinode will be Δx.
Statement II : Spacing between nodes ( consecutive) in the standing wave is equal to half of the wavelength of component waves.
View Solution
