The equation of a wave travelling on a string stretched along the X-axis is given by
y=Ae(−xa+tT)2
(a) Write the dimensions of A, a and T.
(b) Find the wave speed.
(c) In which direction is the wave travelling?
(d) Where is the maximum of the pulse located at t=T and at t=2T?
(a) A has the dimensions of y.
xa and tT are dimensionless.
(b) v=Coefficient of tCoefficient of x
(c) xa and tT are of same sign
(d) ymax=A
Topper's Solved these Questions
- WAVE MOTIONBOOK - DC PANDEYCHAPTER - WAVE MOTIONEXERCISE - Exercise 17.2
2 Videos
- WAVE MOTIONBOOK - DC PANDEYCHAPTER - WAVE MOTIONEXERCISE - Exercise 17.3
4 Videos
- WAVE MOTIONBOOK - DC PANDEYCHAPTER - WAVE MOTIONEXERCISE - Miscellaneous Examples
9 Videos
- VECTORSBOOK - DC PANDEYCHAPTER - VECTORSEXERCISE - Medical enrances gallery
9 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEYCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
Similar Questions
The equation of a wve travelling on a string stretched along the X-axis is given by
y=Ad−(xatT)2
a. Write the dimensions of A, a and T. b. Find the wave speed. c.In which direction is the wave travelling? d.Where is the maximum of the pulse located at t=T?Att=2T?
View SolutionThe equation of a wave travelling along the positive x-axis, as shown in figure at t=0 is given by
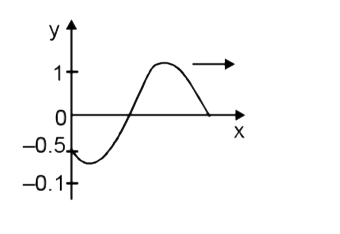
View Solution
Knowledge Check
The equation of a wave travelling on a stretched string along the x-axis is y=ae−(bx+ct). The direction of propagation of wave is
Aalong negative y-axisBalong positive y-axisCalong negative x-axisDalong positive x-axisThe equation of a wave travelling along the positive x-axis, as shown in figure at t=0 is given by :-
_E01_059_Q01.png) .Asin(kx−ωt+π6)Bsin(kx−ωt−π6)Csin(ωt−kx+π6)Dsin(ωt−kx−π6)
.Asin(kx−ωt+π6)Bsin(kx−ωt−π6)Csin(ωt−kx+π6)Dsin(ωt−kx−π6)The equation of a wave travelling in a string can be written as y=3cosπ(100t−x). Its wavelength is
A10 cmB2 cmC5 cmD10 cm
The equation of a wave travelling in a string can be written as y=3cosπ(100t−x). Ita wavelength is
View Solutionthe equation of a wave travelling along the positive x - axis ,as shown in figure at t = 0 is given by
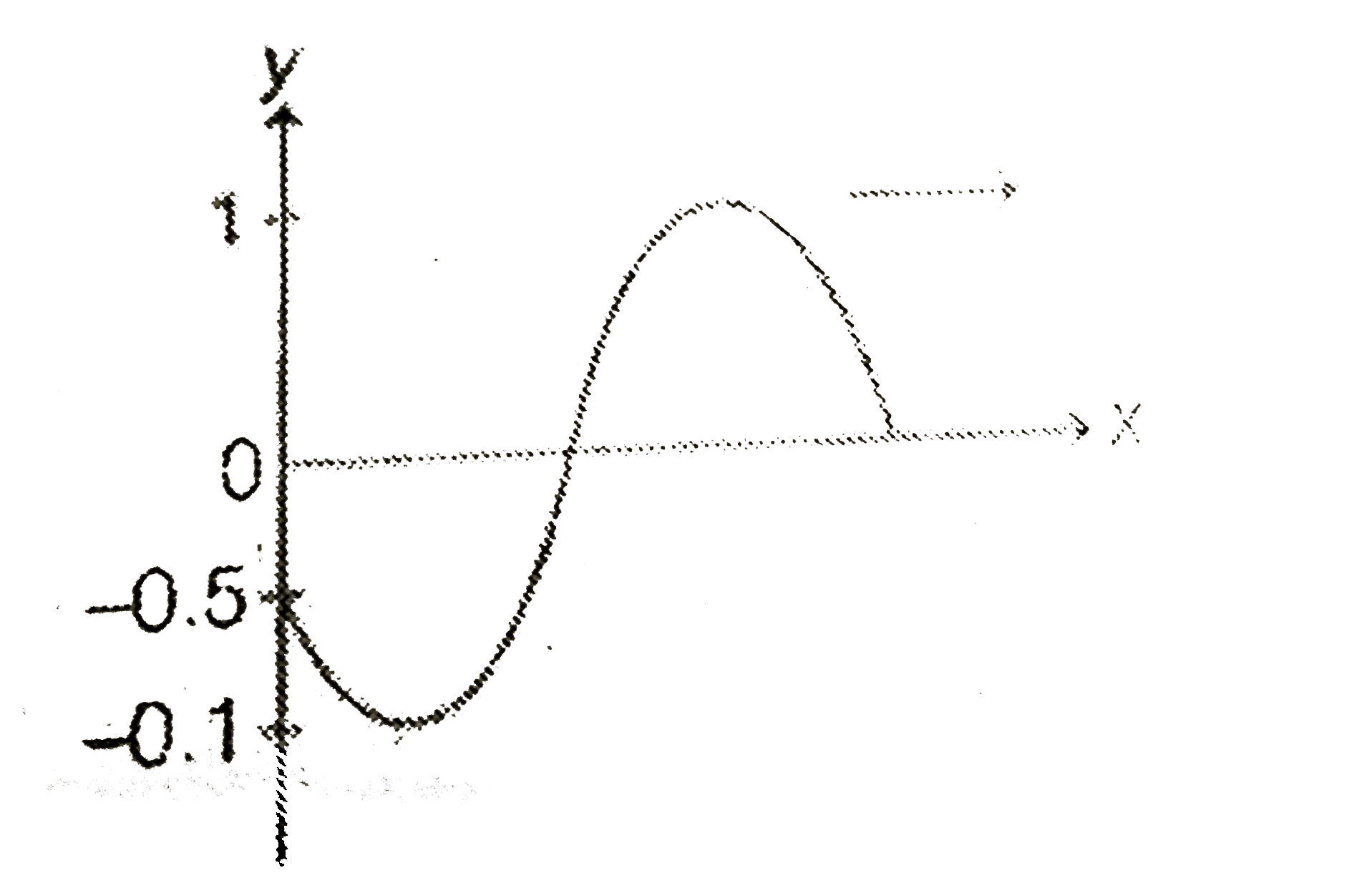
View SolutionThe equation of a wave travelling on a stretched string is y = 4 sin 2π(t0.02−x100)
Here, x and y are in cm and t is in second. The speed of wave is
View SolutionThe equation of a transverse wave along a stretched string is given by
y=15sin2π(t0.04−x40)cm.
The velocity of the wave is
View SolutionThe equation of a wave travelling on a string is y=4sin[π2(8t−x8)], where x,y are in cm and t in second. They velocity of the wave is
View Solution