A hydraulic automobile lift is designed to lift cars with a maximum mass of . The area of cross section of the piston carrying the load is . What maximum pressures would the smaller piston have to bear?
The correct Answer is:A, B
To solve the problem of determining the maximum pressure that the smaller piston in a hydraulic lift would have to bear, we can follow these steps:
Step 1: Identify the mass of the car.
- Given mass (m) = 3000 kg.
Step 2: Calculate the weight of the car.
- The weight (W) can be calculated using the formula:
W=m⋅g
where g is the acceleration due to gravity (approximately 9.8m/s2).
- Thus,
W=3000kg⋅9.8m/s2=29400N
Step 3: Convert the area of the piston from cm² to m².
- Given area (A) = 425 cm².
- To convert to m², use the conversion factor 1cm2=10−4m2:
A=425cm2⋅10−4m2/cm2=0.0425m2
Step 4: Calculate the pressure exerted on the piston.
- Pressure (P) is defined as force (F) divided by area (A):
P=FA
- Here, F is the weight of the car (29400 N) and A is the area of the piston (0.0425 m²):
P=29400N0.0425m2≈691764.71N/m2
Step 5: Convert the pressure to standard scientific notation.
- The pressure can be expressed in scientific notation:
P≈6.92×105N/m2
Final Answer:
The maximum pressure that the smaller piston would have to bear is approximately 6.92×105N/m2.
---
- Given mass (m) = 3000 kg.
- The weight (W) can be calculated using the formula:
W=m⋅g
where g is the acceleration due to gravity (approximately 9.8m/s2).
- Thus,
W=3000kg⋅9.8m/s2=29400N
- Given area (A) = 425 cm².
- To convert to m², use the conversion factor 1cm2=10−4m2:
A=425cm2⋅10−4m2/cm2=0.0425m2
- Pressure (P) is defined as force (F) divided by area (A):
P=FA
- Here, F is the weight of the car (29400 N) and A is the area of the piston (0.0425 m²):
P=29400N0.0425m2≈691764.71N/m2
- The pressure can be expressed in scientific notation:
P≈6.92×105N/m2
The maximum pressure that the smaller piston would have to bear is approximately 6.92×105N/m2.
Topper's Solved these Questions
- FLUID MECHANICSBook:DC PANDEYChapter:FLUID MECHANICSExercise:Subjective Question
Explore 2 Videos
- FLUID MECHANICSBook:DC PANDEYChapter:FLUID MECHANICSExercise:Level 2 Single Correct
Explore 36 Videos
- FLUID MECHANICSBook:DC PANDEYChapter:FLUID MECHANICSExercise:Level 1 Single Correct
Explore 49 Videos
- EXPERIMENTSBook:DC PANDEYChapter:EXPERIMENTSExercise:Subjective
Explore 15 Videos
- GENERAL PHYSICSBook:DC PANDEYChapter:GENERAL PHYSICSExercise:INTEGER_TYPE
Explore 2 Videos
Similar Questions
A hydraulic automobile lift is designed to lift cars with a maximum mass of 300 kg. the area of cross-section of the piston carrying the load is 425cm3. What maximum pressure would smaller piston have to bear?
View SolutionWhen a pressure measuring device is immersed in a liquid, a restoring force of 2 N is exerted by the spring. If the area of cross-section of the pistion is 10 cm2, what pressure does the fluid exert on the piston?
View Solution
Knowledge Check
A lift is tied with thick iron wire and its mass is 1000kg. If the maximum acceleration of the lift is 1.2ms2 and the maximum stress of the wire is 1.4×108Nm2 what should be the minimum diameter of the wire?
A10−2mB10−4mC10−6mD0.5×10−2mAn object of mass 100 kg is to be lifted by a 10 kg effort. The possible values of the area of cross sections of the pump piston and press piston are ____ and ____, repectively, both in the same unit.
A100, 1B10,1C1100D2, 20
The figure shows a schematic layout of hydraulic jack. The load to be raised weighs 20,000N. The area of cross section of the two pistons are A1=50cm2 and A2=10cm2. The force (F) is applied at theend of a ligh lever bar as shown in the figure. Lenghts a and b are 4cm and 36cm respectively. Find the force (F) required to raise the load slowly.
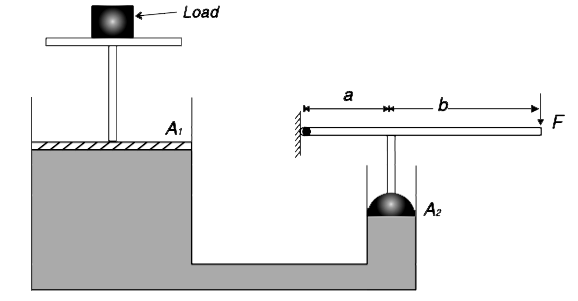
View Solution2 moles of a diatomic gas are enclosed in a cylinder piston arrangment. The area of cross section and mass of the piston are 1cm2 and 1 kg respectively. A heater is supplying heat to the gas very slowly. Find heat supplied (in joule) by the heater is the piston moves through a distance of 10 cm.
 .
.
View SolutionConsider a vertical tube open at both ends. The tube consistss of two parts, each of different cross sections and each part having a piston which can move smoothly in respective tubes. The two piston which can move smoothly in respective tube wire. The piston are joined together by an inextensible wire. The combined mass of the two piston is 5kgand area of cross section of the upper piston is 10cm2 greater than that of the lower piston. Amount of gas enclosed by the pistons is 1mol. When the gas is heated slowly, pistons move by 50cm. Find the rise in the temperature of the gas in the form X/RK, where R is universal gas constant. Use g=10m/s2 and outside pressure =105N/m2).

View SolutionAn object of weight 500kgwt is lifted by using a hydraulic press. The radii of the cross sections of the pump piston and press piston are 10 cm and 20 cm, respectively. Calculate the effort required to lift the object. Calculate the displacement of the load if the effort is moved through a distance of 20 cm.
View SolutionAn object of weight 500kgwt is lifted by using a hydraulic press. The radii of the cross sections of the pump piston and press piston are 10 cm and 20 cm, respectively. Calculate the effort required to lift the object. Calculate the displacement of the load if the effort is moved through a distance of 20 cm.
View SolutionFigure shows a liquid being pushed out of a tube by pressing a piston. The area of cross section of the piston is 1.0 cm^2 and that of the tube at the outlet is 20mm2. If the piston is pushed at a speed of 2cms−1. What is the speed of the outgoing liquid?

View Solution