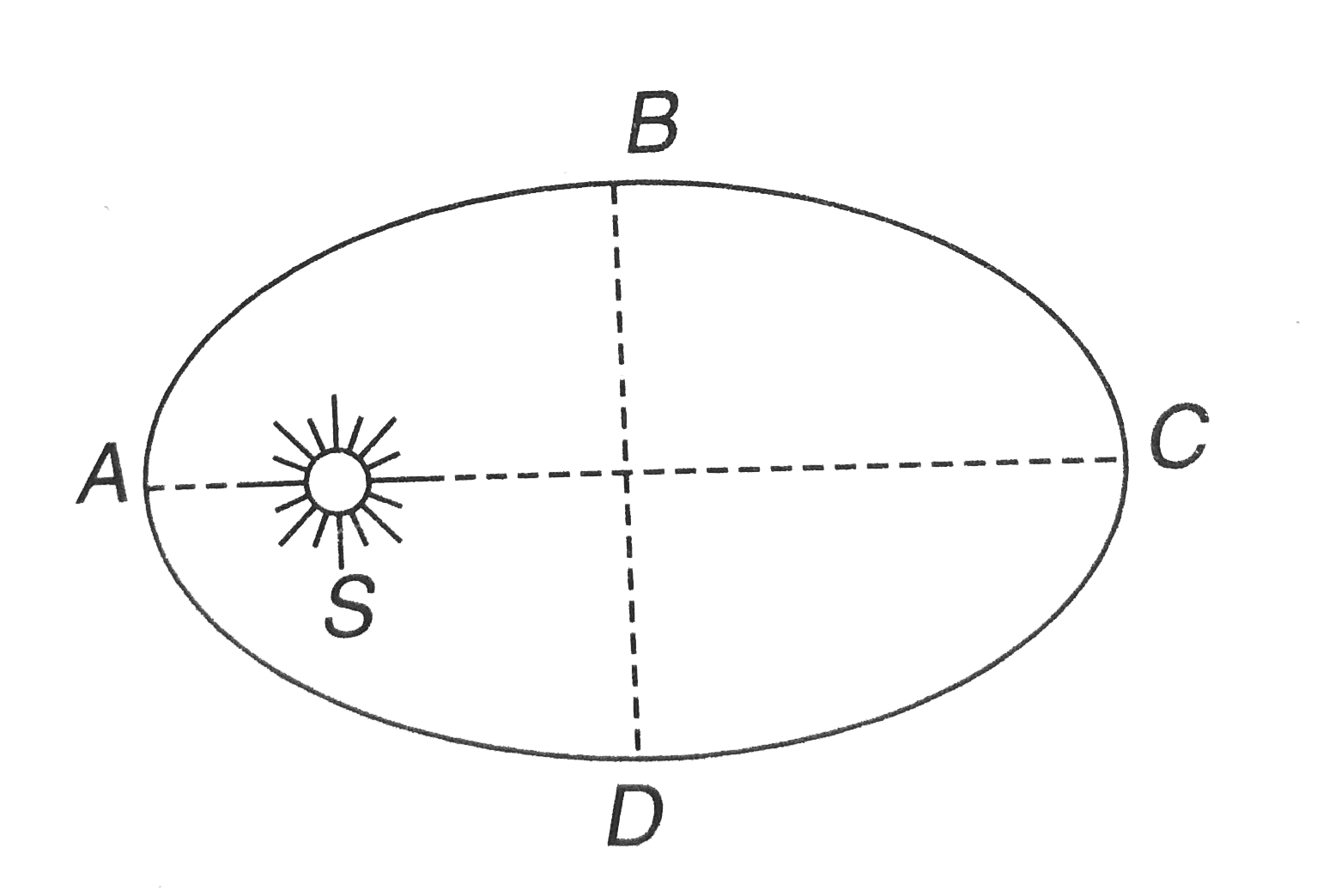
For a planet revolving around sun, if a and b are the respective semi-major and semi-minor axes, then the square of its time period is proportional to
(a+b2)3
(a−b2)3
b3
a3
The correct Answer is:D
Step by step video, text & image solution for For a planet revolving around sun, if a and b are the respective semi-major and semi-minor axes, then the square of its time period is proportional to by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - Level 1 Subjective
19 Videos
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - Level 2 Single Correct
22 Videos
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - Level 1 Assertion And Reason
11 Videos
- GENERAL PHYSICSBOOK - DC PANDEYCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
- KINEMATICSBOOK - DC PANDEYCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
11 Videos
Similar Questions
Knowledge Check
A planet revolves in an elliptical orbit around the sun. The semi-major and minor axes are a and b , then the time period is given by :
AT2∝b3BT2∝(a+b2)3CT2∝a3DT2∝(a−b2)3A planet revolves round the sun in an elliptical orbit of semi minor and semi major axes x and y respectively. Then the time period of revolution is proportional to
A(x+y)32B(y−x)32Cx32Dy32A planet is revolving around the sun in a circular orbit with a radius r. The time period is T .If the force between the planet and star is proportional to r−3/2 then the quare of time period is proportional to
Ar3/2Br2CrDr5/2
A planet revolves in an ellipticle orbit around the sun. the semimjor and semiminor axis are a and b. How time-period is related with them?
View SolutionImagine a light planet revolving around a very massive star in a circular orbit of radius r with a period of revolution T.If the gravitational force of attraction between the planet and the star is proportional to r−3 ,then the square of the time period will be proportional to
View SolutionFor a planet revolving around Sun in an elliptical orbit, to obtain its velocity at any point we have to apply
View SolutionIf a planet of mass m is revolving around the sun in a circular orbit of radius r with time period, T then mass of the sun is
View SolutionA planet is revolving around the sun as shown in elliptical path. The correct option is
View Solution
