A 4.00g bullet travelling horizontally with a velocity of magnitude 500m/s is fired into a wooden block with a mass of 1.00kg, initially at rest on a level surface. The bullet passes through the block and emerges with speed 100m/s. The block slides a distance of 0.30m along the surface from its initial position.
(a) What is the coefficient of kinetic friction between block and surface?
(b) What is the decrease in kinetic energy of the bullet?
(c) What is the kinetic energy of the block at the instant after the bullet has passed through it? Neglect friction during collision of bullet with the block.
The correct Answer is:A, B, C, D

(a) pi=pf
∴mv0=mv1+mv2
∴v2=mv0−mv1M
=(0.004)(500−100)1.0
=1.6m/s
Retardation due to friction,
a=μmgm=μg=(10μ)
s=v222a=v2220μ
∴μ=v2220s
=(1.6)220×0.3=0.43
(b) Decrease in kinetic energy of bullet
=12m(v20−v21)
=12×0.004[(500)2−(100)2]
=480J
(c) Kinetic energy of block,
=12Mv22
=12×1.0×(1.6)2
=1.28J
Topper's Solved these Questions
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEYCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 1 Subjective Questions
1 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEYCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Single Correct
23 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEYCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 1 Objective
42 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEYCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
- CIRCULAR MOTIONBOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Medical entrances s gallery
19 Videos
Similar Questions
A bullet of mass 20 g travelling horizontally with a speed of 500 m/s passes through a wooden block of mass 10.0 kg initially at rest on a level surface. The bulet emerges with speed of 100 m/s and the block slides 20 cm on the surface before coming to rest. Find the friction cioefficient between the block and the surface figure.

View SolutionA block begins to move on a rough horizontal surface with an initial velocity u. If it loses of its initial kinetic energy in time t, the coefficient of kinetic friction between the block and the surface is
View Solution
Knowledge Check
A block of mass M is using on a rough horizontal surface. μR is the coefficient of kinetic friction between the block and the surface. What is the net force exerted by the surface on the block?
AMgBμMgCMg√1+μ2KDMg√1−μ2KA block B is pushed momentarily along a horizontal surface with an initial velocity v. If μ is the coefficient of sliding friction between B and the surface, block B will come to rest after a time
 AvgμBgμvCgvDvg
AvgμBgμvCgvDvgA block B is pushed momentarily along a horizontal surface with an initial velocity v. If μ is the coefficient of sliding friction between B and the surface, block B will come to rest after a time
 AvgμBgμvCgvDvg
AvgμBgμvCgvDvg
A bullet fired horizontally with a speed of 400m/sec. It strikes a wooden block of mass 5kg initially at rest placed on a horizontal floor as shown in the figure.It emerges with a speed of 200m/sec and the block slides a distance 20cm before coming to rest.Find the coefficient of friction between block and the surface.Mass of bullet is 20g . (take g=10ms2)
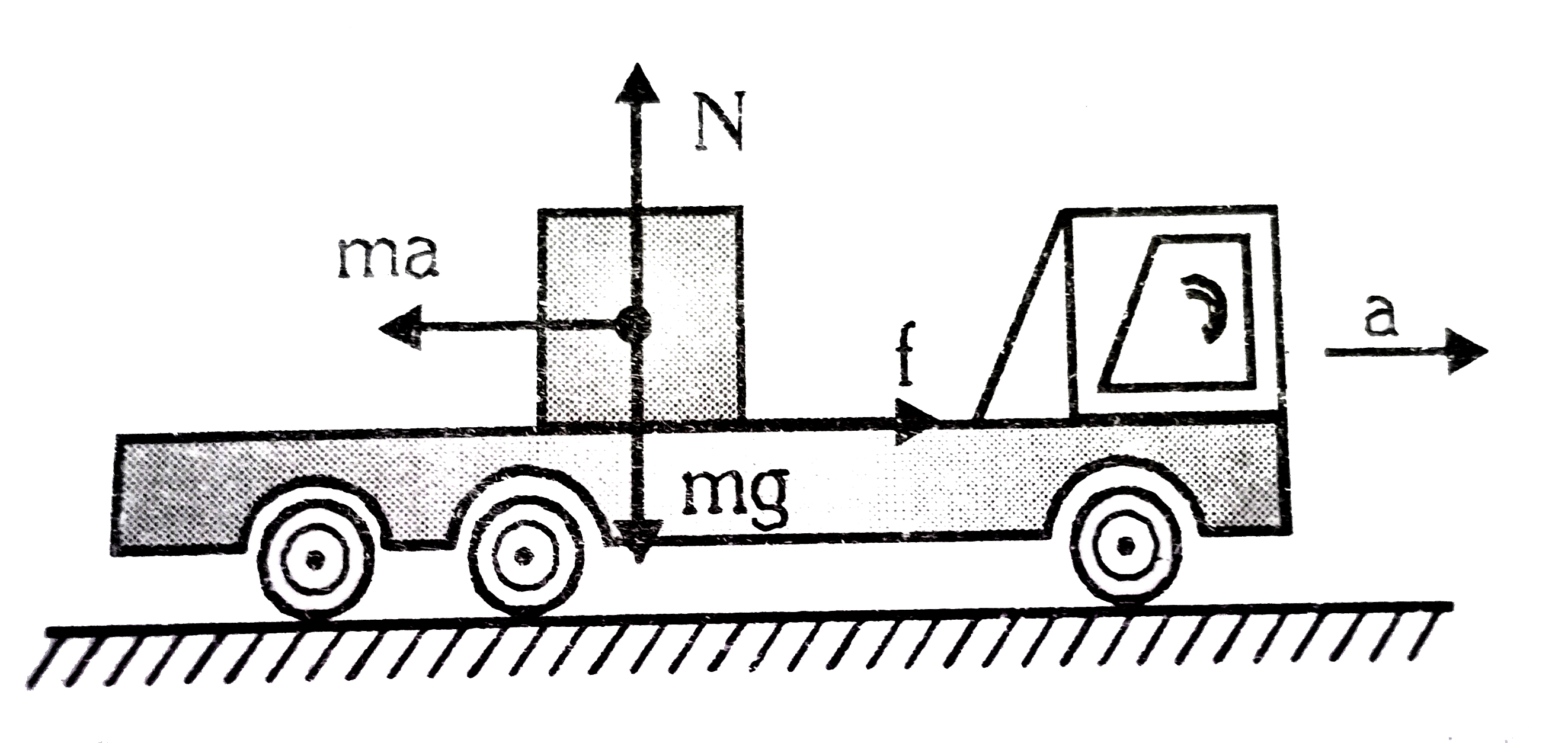
View SolutionA block of mass 1kg lies on a horizontal surface in a turck, the coefficient of static fricition between the block and the surface is 0.6, what is the force of fricition on the block. If the acceleration of the truck is 5m/s2.
View SolutionA block B is pushed momentarily along a horizontal surface with an initial velocity v . If mu is the coefficient of sliding friction between B and the surface, block B will come to rest after a time:

View SolutionIn the previous problem , the bullet penetrates the block and emerges with speed v0.If the block travels 2.5m on the rough surface , the value of v0 is
View SolutionA wooden block of mass M resting on a rough horizontal surface is pulled with a force F at an angle with the horizontal. If is the coefficient of kinetic friction between the block and the surface, then acceleration of the block is -
View Solution
