The momentum of a system with respect to centre of mass
(a) is zero only if the system is moving uniformly
(b) is zero only if no external force acts on the system
(c) is always zero
(d) can be zero in certain conditions
The correct Answer is:C
Step by step video, text & image solution for The momentum of a system with respect to centre of mass by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEYCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 1 Subjective
32 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEYCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 1 Subjective Questions
1 Video
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEYCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 1 Assertion and Reason
15 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEYCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
- CIRCULAR MOTIONBOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Medical entrances s gallery
19 Videos
Similar Questions
A rigid system consists of two point masses,A and B of masses 1kg and 2kg respectively.At an instant the kinetic energy of A with respect to the centre of mass is 2 Joules and the velocity of centre of mass is 2m/s.The kinetic energy of the system at this instant is?
View SolutionA satellite of mass m is in a circular orbit of radius r round the Earth. Calculate its angular momentum with respect to the centre of the orbit in terms of the mass M of the Earth and G.
View Solution
Knowledge Check
A comet of mass m moves in a highly elliptical orbit around the sun of mass M the maximum and minium distacne of the comet from the centre of the sun are r1 and r2 respectively the magnitude of angular momentum of the comet with respect to the centre of sun is
A[GM1r1+r2]1/2B[GMmr1r1+r2]1/2C[rGM2r1r2r1+r2]1/2D[2GMm2r1r2r1+r2]1/2Statement-1: The kinetic energy of a system of particles is minimum from centre of mass frame of reference.
Statement-2 : The total momentum of a system as seen from centre of mass frame is minimum.AStatement-1 is true, statement-2 is true and statement-2 is correct explanation for statement-1.BStatement -1 is true, statement-2 is true and statement-2 is NOT the correct explanation for statement-1.CStatement-1 is true, statement-2 is false.DStatement-1 is false, statement-2 is true.The rate of change of angular momentum of a system of particles about the centre of mass is equal to the sum of external torque about the centre of mass when the centre of mass is :
AFixed with respect to an inertial frame.Bin linear acceleration.Cin rotational motion.Dis in a translational motion.
If the external forces acting on a system have zero resultant, the centre of mass :
View SolutionIf the external forces acting on a system have zero resultant, the centre of mass :
View SolutionStatement-1 : Total linear momentum of system in centre of mass frame is zero only when there is no net external force.
Statement-2 : Total linear momentum of system is conserved in absence of net external force.
View SolutionConsider the following two statements:
A. Kinear momentum of the system remains constant.
B. Centre of mass of the system remains at rest
View Solution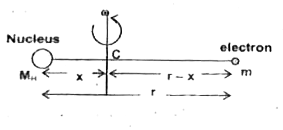
The angular momentum of the atom about the centre of mass will be
View Solution