The sphere at A is given a downward velocity v0 of magnitude 5m/s and swings in a vertical plane at the end of a rope of length l=2m attached to a support at O . Determine the angle θ at which the rope will breack, knowing that it can withstant a maximum tension equal to twich the weight of the sphere.
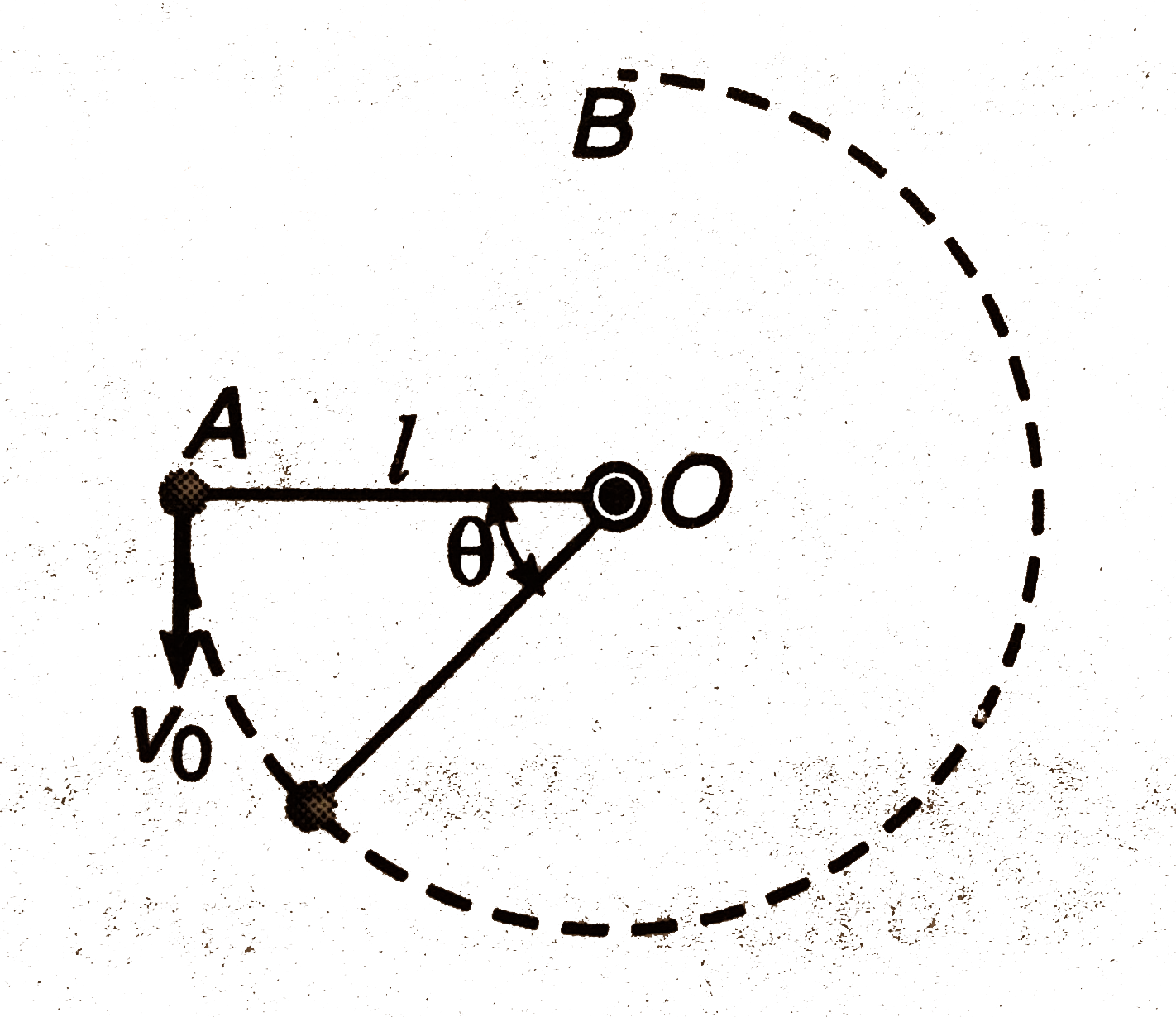
The correct Answer is:A, D
v2=v20+2gh=v20+2gRsinθ
=(5)2+2×10×2sinθ
=(25+40sinθ)
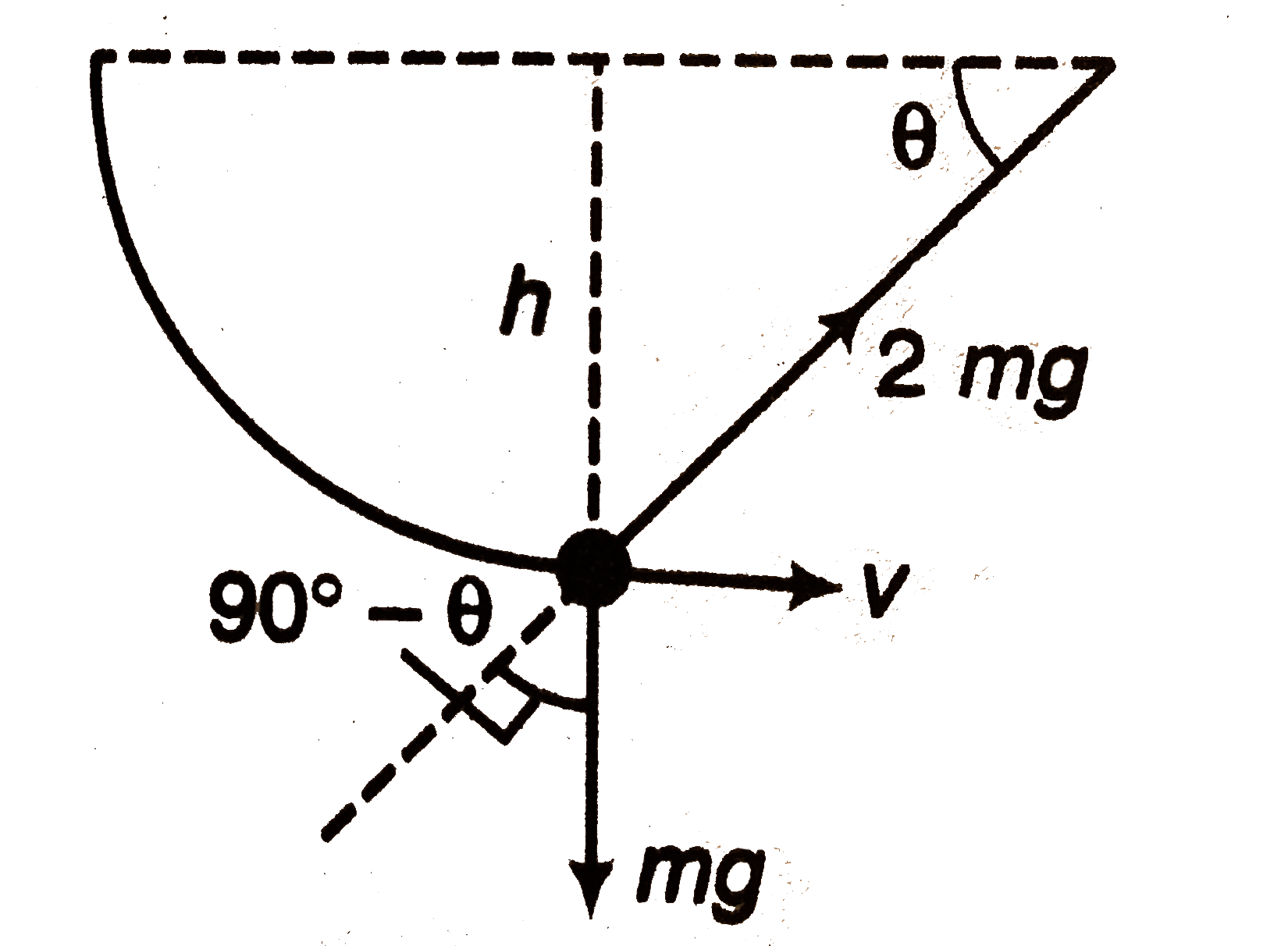
Now, 2mg−mgsinθ=mv2R
or 2g−gsinθ=v2R
or 2×10−10sinθ=25+40sinθ=25+40sinθ2
Solving this equation we get,
θ=sin−1(14)
Topper's Solved these Questions
- CIRCULAR MOTIONBook:DC PANDEYChapter:CIRCULAR MOTIONExercise:Level 2 Single Correct
Explore 18 Videos
- CIRCULAR MOTIONBook:DC PANDEYChapter:CIRCULAR MOTIONExercise:Level 2 More Than One Correct
Explore 5 Videos
- CIRCULAR MOTIONBook:DC PANDEYChapter:CIRCULAR MOTIONExercise:Level 1 Objective
Explore 14 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBook:DC PANDEYChapter:CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONExercise:Level 2 Subjective
Explore 21 Videos
- COMMUNICATION SYSTEMBook:DC PANDEYChapter:COMMUNICATION SYSTEMExercise:Only One Option is Correct
Explore 27 Videos
Similar Questions
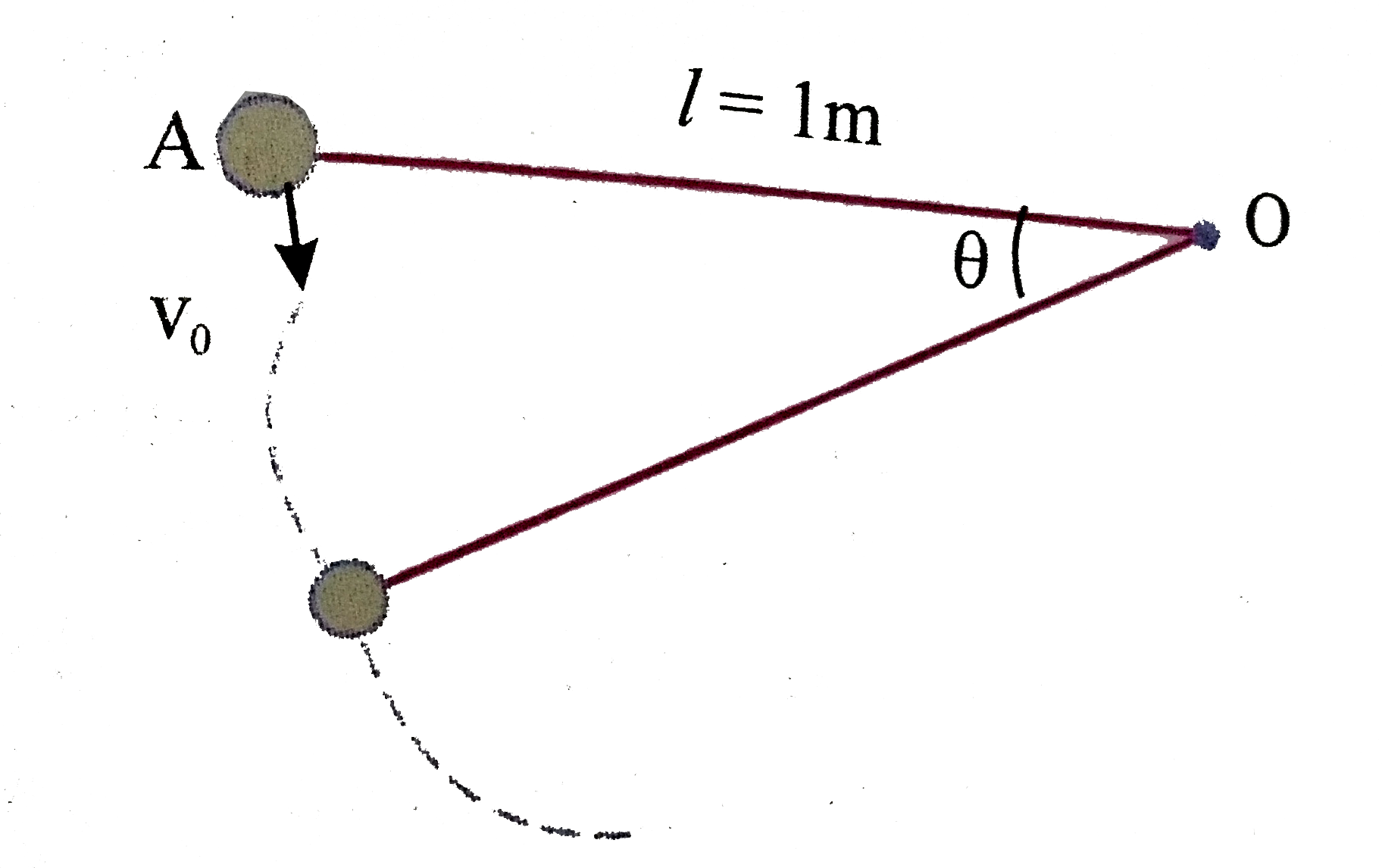
The sphere at P is given a down ward velocity v0 and swings in a vertical plane at the end of a rope of l=1m attached to a support at O. The rope breaks at angle 30∘ from horizontal, knowing that it can withstand a maximum tension equal to four times the weight of the sphere. then the value of v0 will be (g=10m/s2).
 .
.
View SolutionA small body of mass m = 0.1 kg swings in a vertical circle at the end of a chord of length 1 m. Its speed is 2 m/s when the chord makes an angle θ=30∘ with the vertical. Find the tension in the chord.
View Solution
Knowledge Check
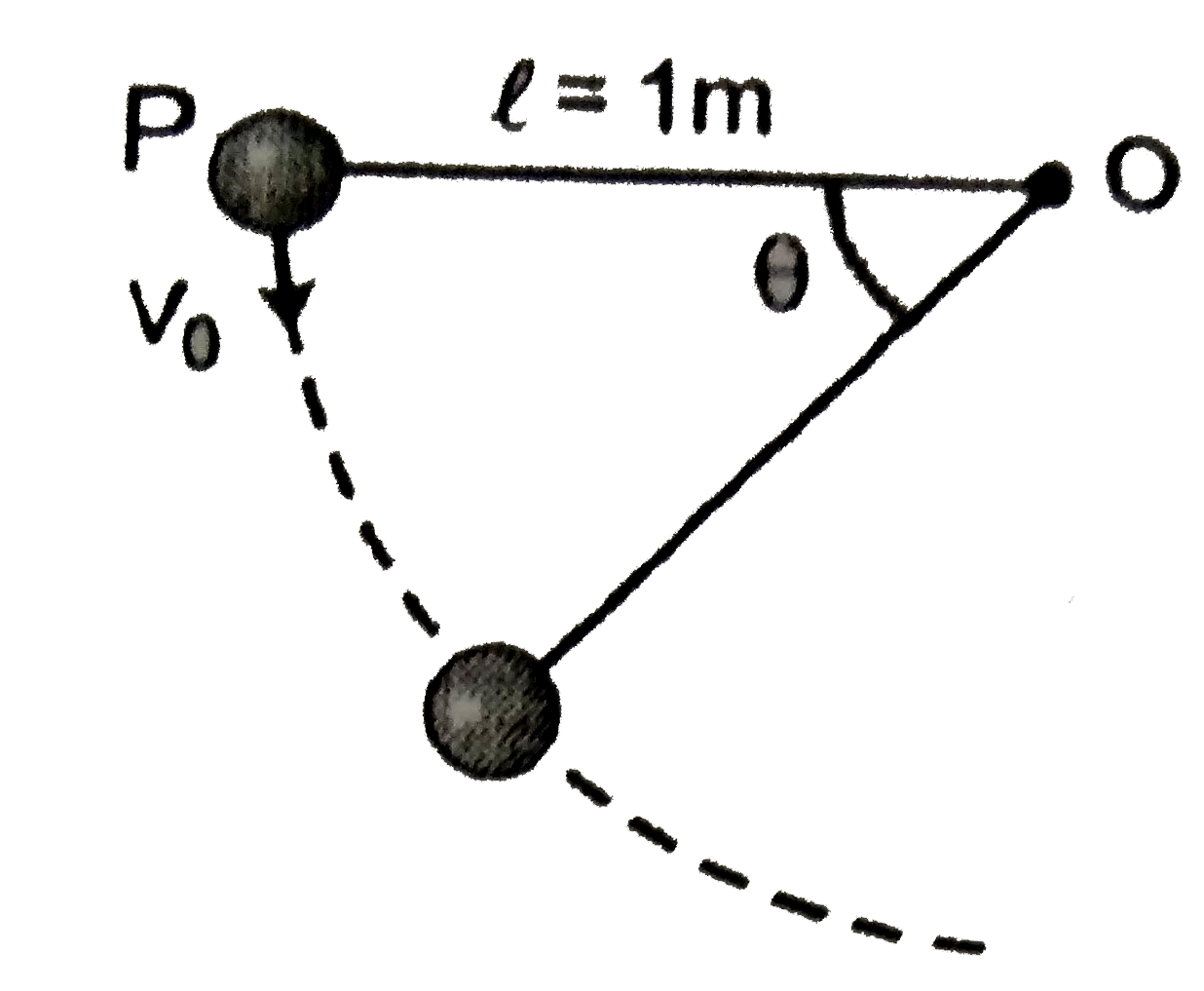
The sphere at P is given a downward velocity v0 and swings in a vertical plane at the end of a rope of l=1m attached to a support at O. The rope breaks at angle 30∘ from horizontal , knowing that it can withstand a maximum tension equal to three times the weight of the sphere. Then the value of v0 will be :
(g=π2m/s2)
 Ag2m/sB2g3m/sC√3g2m/sDwill have minimum value 38m
Ag2m/sB2g3m/sC√3g2m/sDwill have minimum value 38mA small sphere is given vertical velocity of magnitude v0=5ms−1 and it swings in a vertical plane about the end of a massless string. The angle θ with the vertical at which string will break, knowing that it can withstand a maximum tension equal to twice the weight of the sphere, is
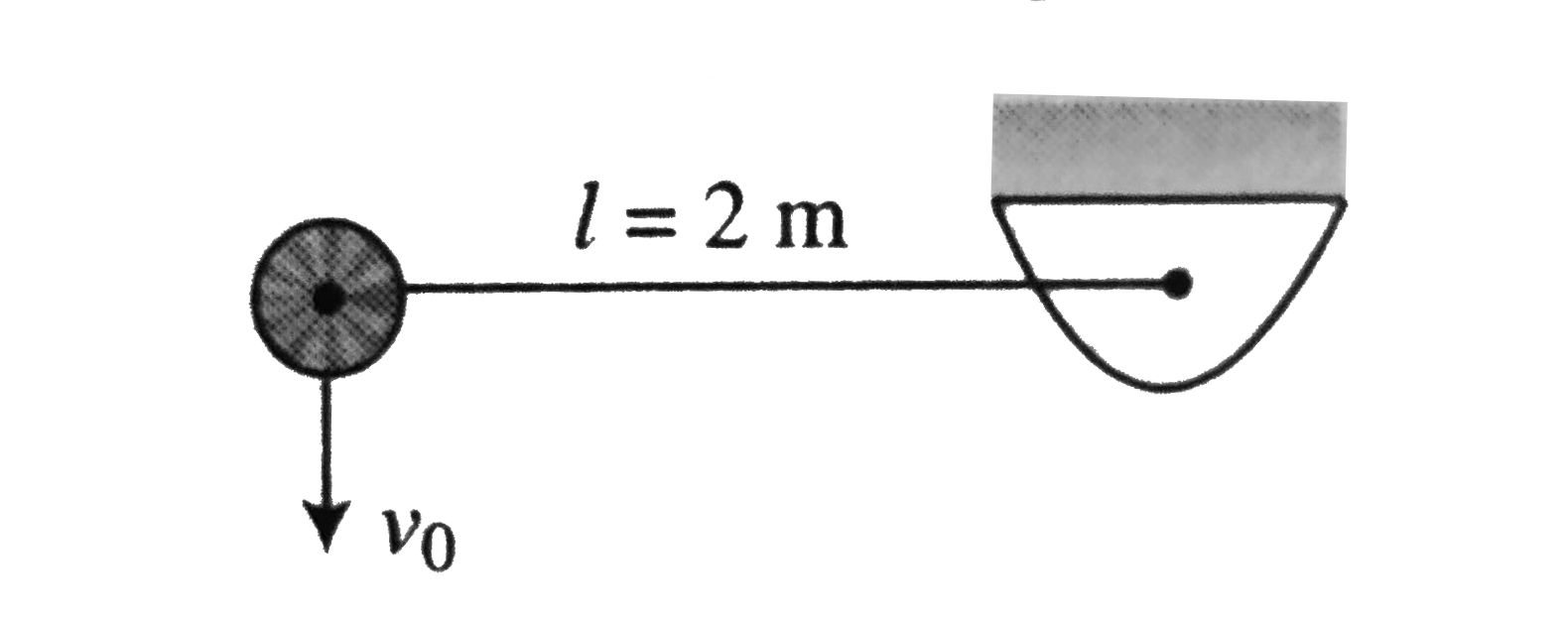 A(a) cos−123B(b) cos−1(14)C(c) 60∘D(d) 30∘
A(a) cos−123B(b) cos−1(14)C(c) 60∘D(d) 30∘A stone is whirled in a vertical circle at the end of a rope of length 0.5m . The velocities of the stone at lowest and highest points to just complete the circle are [Take,g=9.8ms−2]
A4.949and2.213ms−1B5.921and3.312ms−1C1.235and2.312ms−1DNone of these
A bob tied to the end of a string of length 2m, other end of which is fixed at a point in a vertical wall at a point O. The bob is imparted a vertical downward velocity of 5m/s when the string is horizontal and swings in a vertical plane. Find the angular displacement of the bob from its initial position, when the string breaks. Given that the tensile strength of the string is twice the weight of the bob. Take g=10m/s2
View SolutionA 40-kg mass hanging at the end of a rope of length l. Oscillates in a vertical plane with an angular of θ0. What is the tension in the rope when it makes an angle θ with the vertial ? If the breaking strength of the rope is 80 kg, what is the maximum angular amplitude with which the mass can oscillate without the rope breaking ?
View SolutionA stone of mass 0.5kg is attached to a string of length 2m and is whirled in a horizontal circle. If the string can withstand a tension of 9N the maximum velcoity with which the stone can be whirled is .
View SolutionA stone of mass 0.5 kg is attached to a string of length 2m and is whirled in a horizontal circle. If the string can withstand a tension of 9N, the maximum velocity with which the stone can be whirled is
View SolutionA simple pendulum of length L swings in a vertical plane. The tension of the string when it makes an angle θ with the vertical and the bob of mass m moves with a speed v is (g is the gravitational acceleration)
View Solution