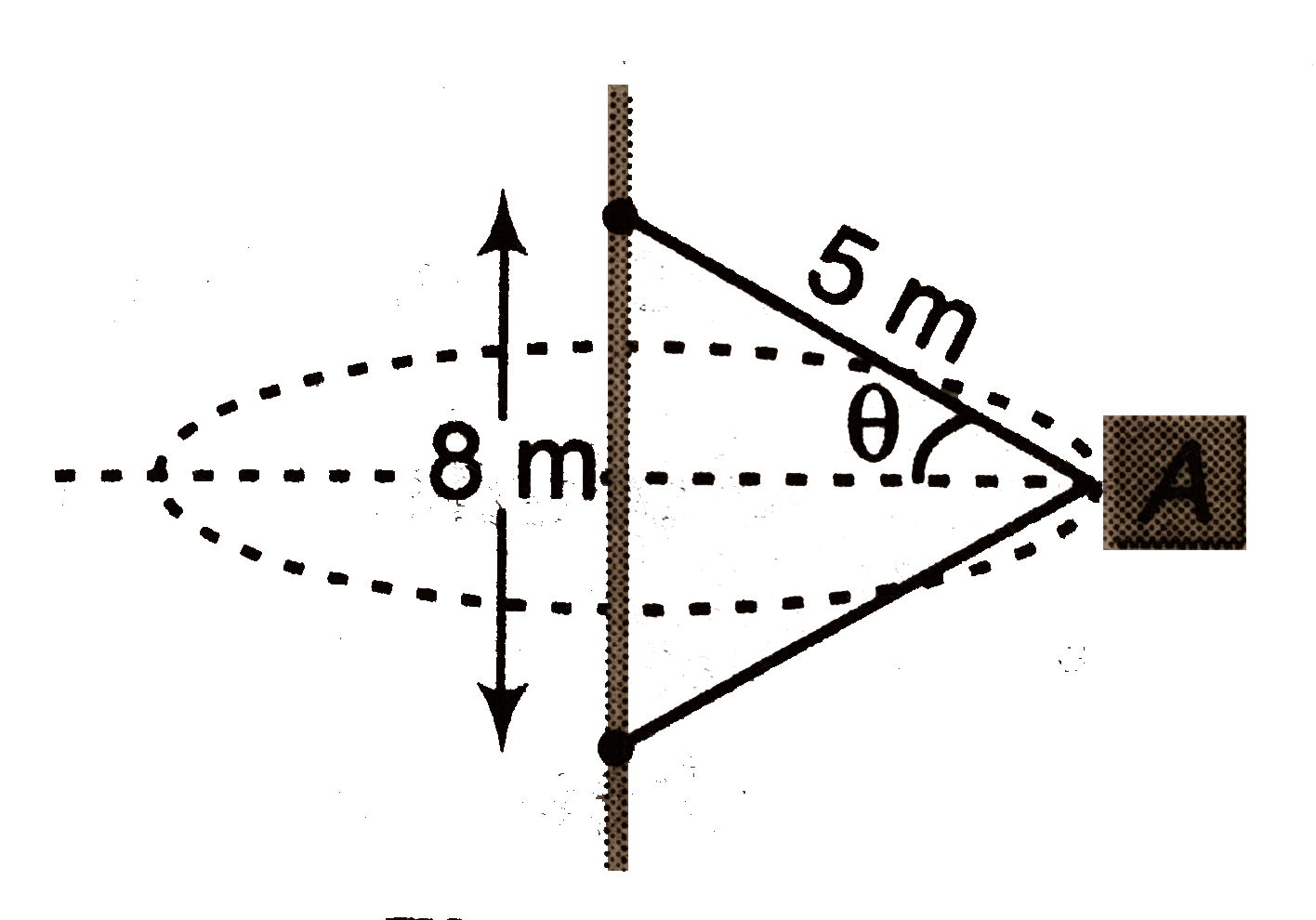
A 4kg block is attached to a vertical rod by means of two strings of equal length. When the system rotates about the axis of the rod, the strings are extended as shown in figure.
(a) How many revolutions per minute must the system make in order for the tension in the upper string to be 200N ?
(b) What is the tension in the lower string then ?
The correct Answer is:A, B, C
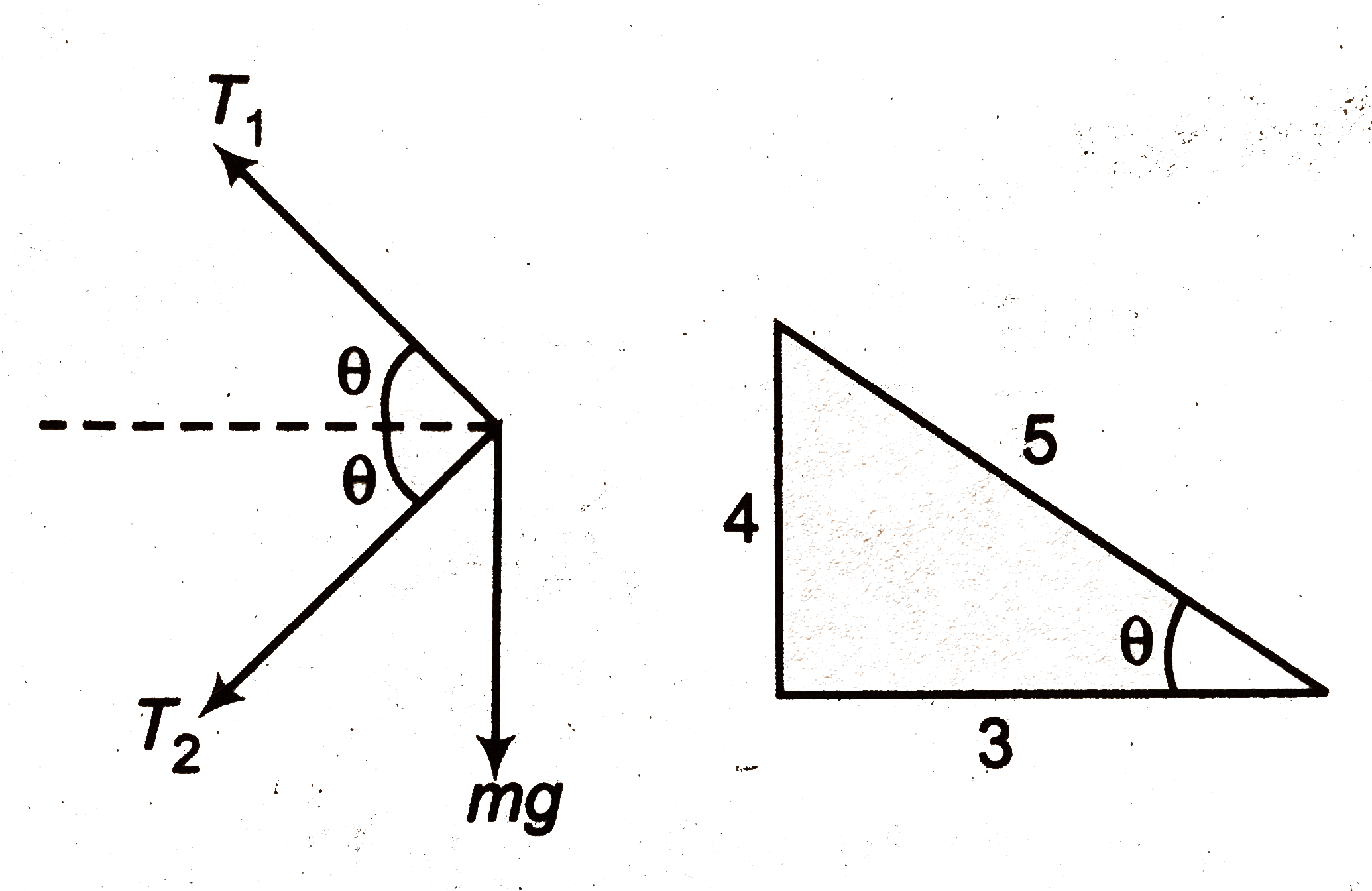
T1cosθ+T2cosθ=mrω2
ω=(2nπ)
Here, n=number of revolutions per second. Substituting the proper values in Eq. (i) ,
200(35)+T2×(35)=(4)(3)(2nπ)2
or 600+3T2=240n2π2
Further, T1sinθ=T2sinθ+mg
or 200×45=T2×45+4×10
or 800=4T2+200
Solving Eqs. (ii) and (iii) we get,
T2=150N and n=0.66rps
=39.6rpm
Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Exercise 10.3
4 Videos
- CIRCULAR MOTIONBOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Assertion And Reason
28 Videos
- CIRCULAR MOTIONBOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Exercise 10.1
7 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEYCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective
21 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEYCHAPTER - COMMUNICATION SYSTEMEXERCISE - Only One Option is Correct
27 Videos
Similar Questions
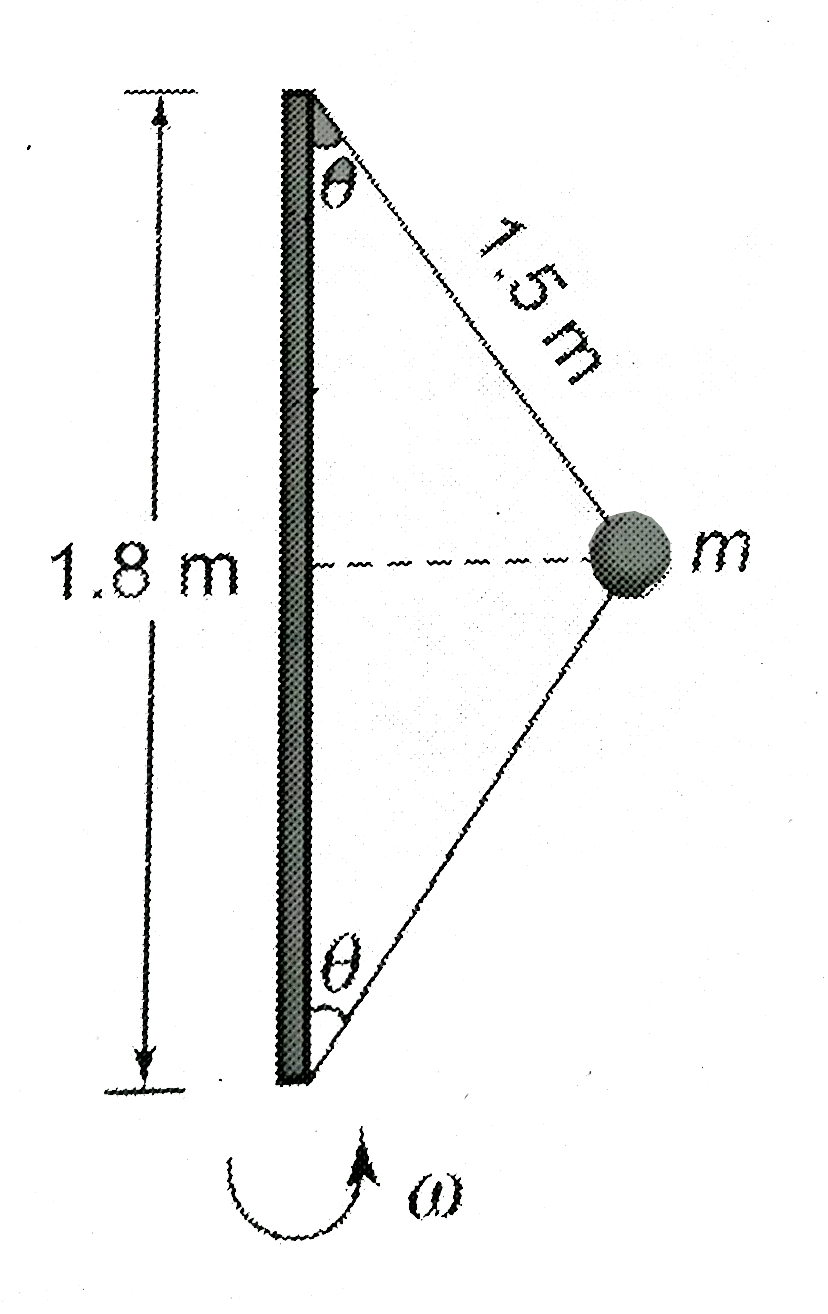
a ball of mass 240g is attached to the verticle rod by means of two strings. When the ball rotates about the axis of rod, the strings are extended as shown in the figure.
(a) find the angular speed of the ball if tension in lower string is 16N.
(b) What is tension in the upper string?
View SolutionFor the system shown in figure, the tensions in the string
View Solution
Knowledge Check
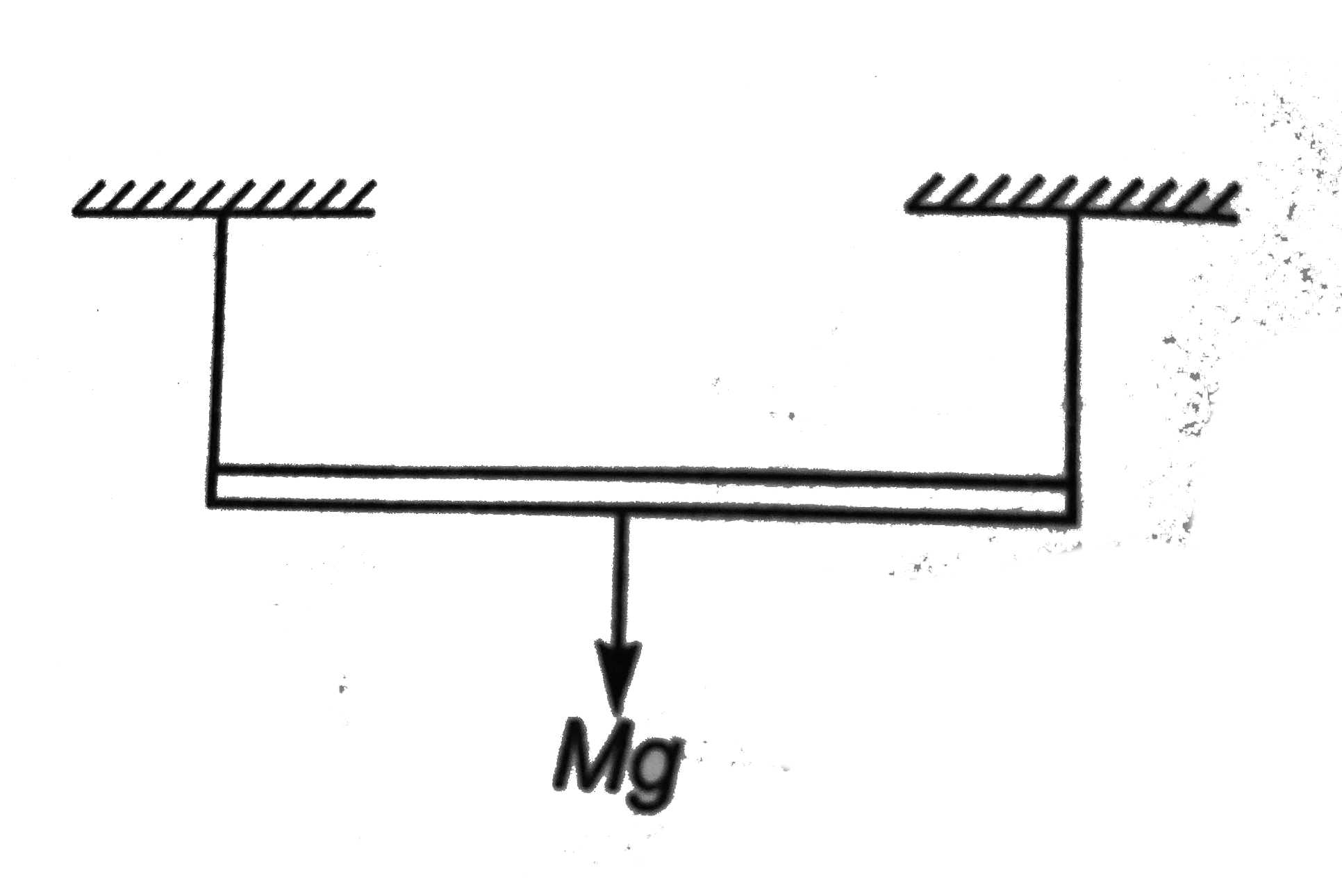
A rod is supported horizontally by means of two strings of equal length as shown in figure. If one of the string is cut. Then tension in other string at the same instant will.Aremains unaffectedBincreaseCdecreaseDbecome equal to weight of the rod.A block of weight W is supported by three strings as shown in figure. Which of the following relations is true for tension in the string ? (Here, T1,T2 and T3 are the tension in the strings A,B and C respectively)
 AT1=T2BT1=T3CT2=T3DT1=T2=T3
AT1=T2BT1=T3CT2=T3DT1=T2=T3The tension in the string in the pulley system shown in
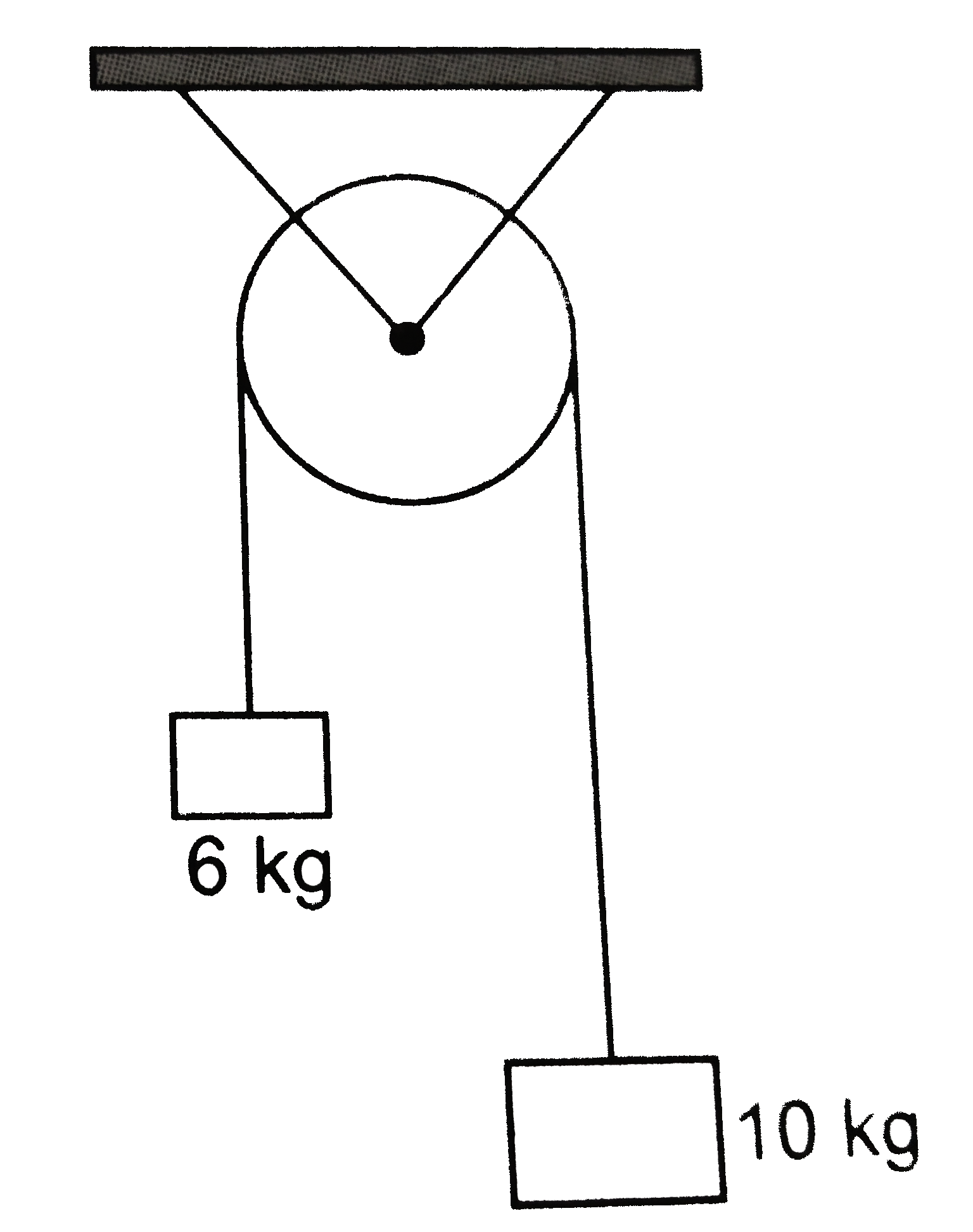 .A5.7NB7NC7.5ND73.5N
.A5.7NB7NC7.5ND73.5N
The tension T in the string shown in figure is

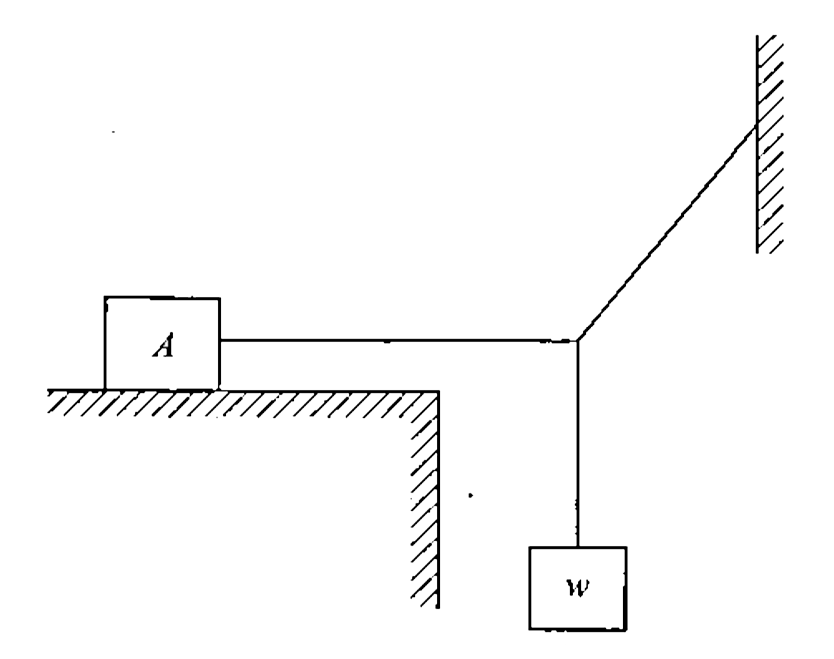
View SolutionConsider the situation shown in figure. Find the acceleration of the system and the tension in the strings.
View SolutionIn the situation shown, the tension in the string is 22N. What is m (in kg) ?
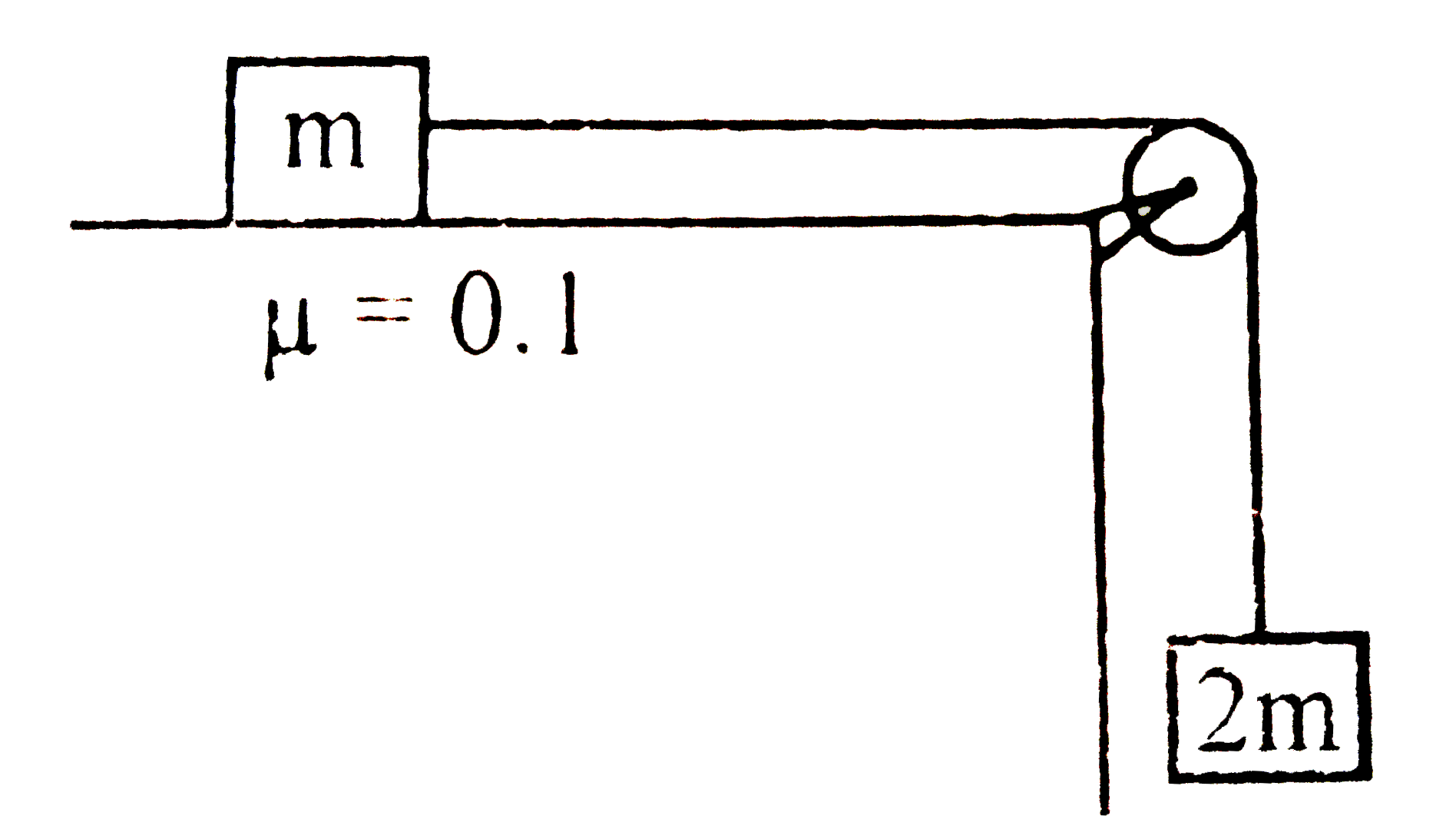
View SolutionFor the system shown in the figure,the pulleys are light and frictionless.The tension in the string will be
View SolutionIf vibrations of a string are to be increased by a factor of two, then tension in the string must be made
View Solution
