In the system shown in figure pully is smooth. Strings is massless and inextensible.Find acceleration of the system a, tension T1 andT2 (g=10m/s2)
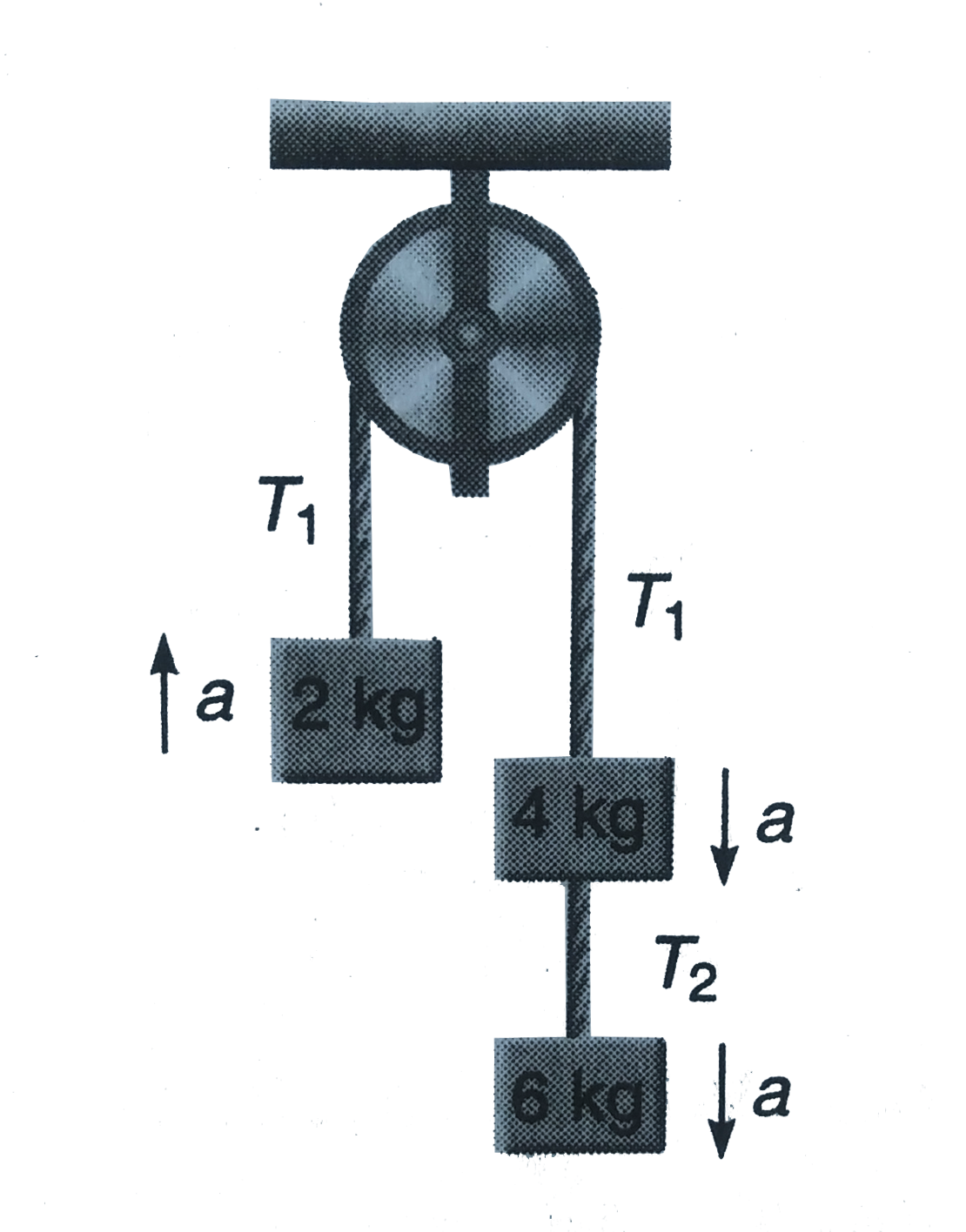
Here net pulling force will be
Weight of 4kg and 6kg blocks on one side -weight of 2kg blocks on one the other side - therefore.
a=Net pulling forceTotal mass
=(6×10)+(4×10)−(2)(10)6+4+2
=203m/s2
for T1, Let us consider FBD of 2kg block. Writing equation of motion, we get.
T1−20=2a or T1+20×2203=1003N
For T2,We may consider FBD of6kg block. Writing equation of motion, we get.
60−T2=6a
T2=60−6a=60−6[203]
=603N
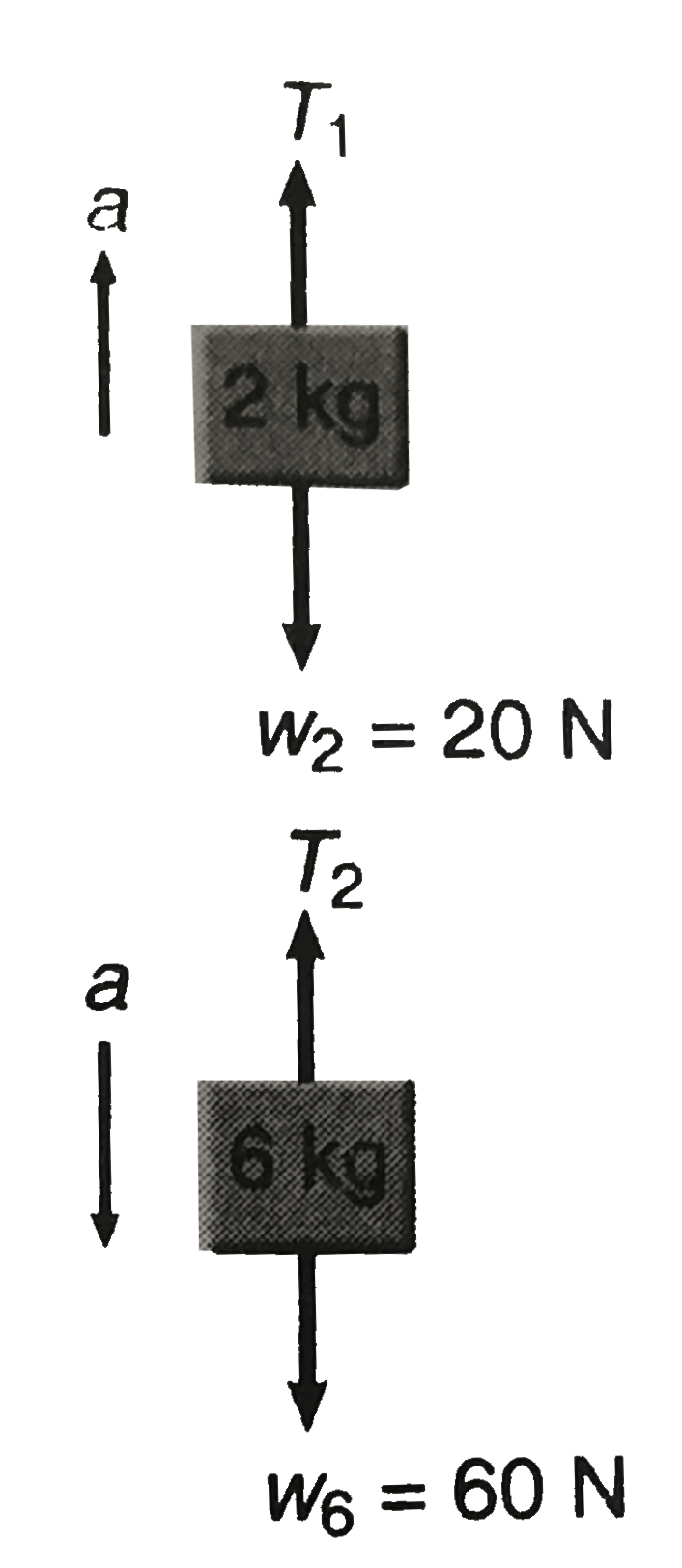
Similar Questions
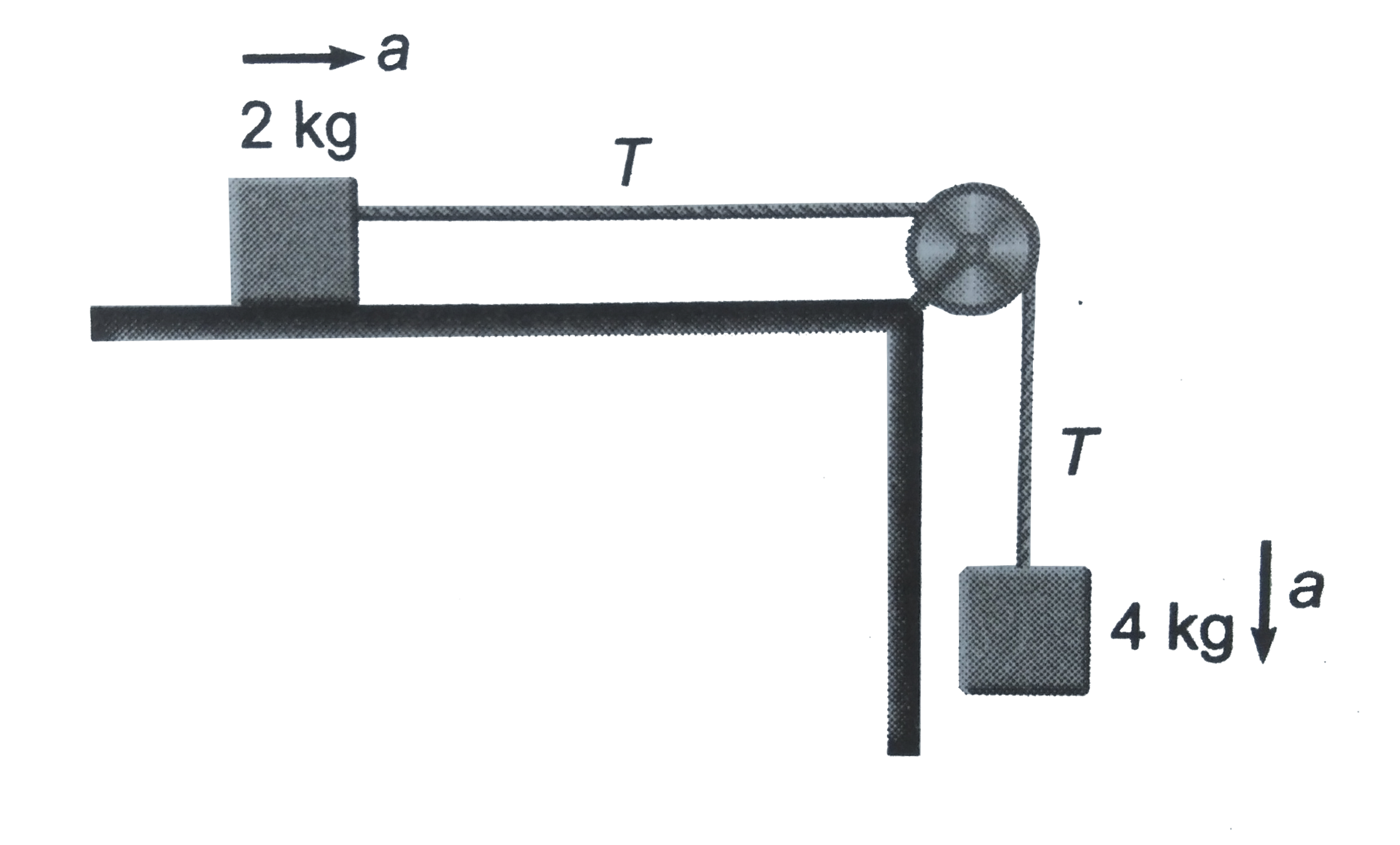
In the system shown in figure all surfaces are smooth. Strings is massless and inextensible.Find acceleration of the system and tension T in the string (g=10m/s2)
View SolutionIn the system shown in figure all surface are smooth, string in massless and inextensible. Find:
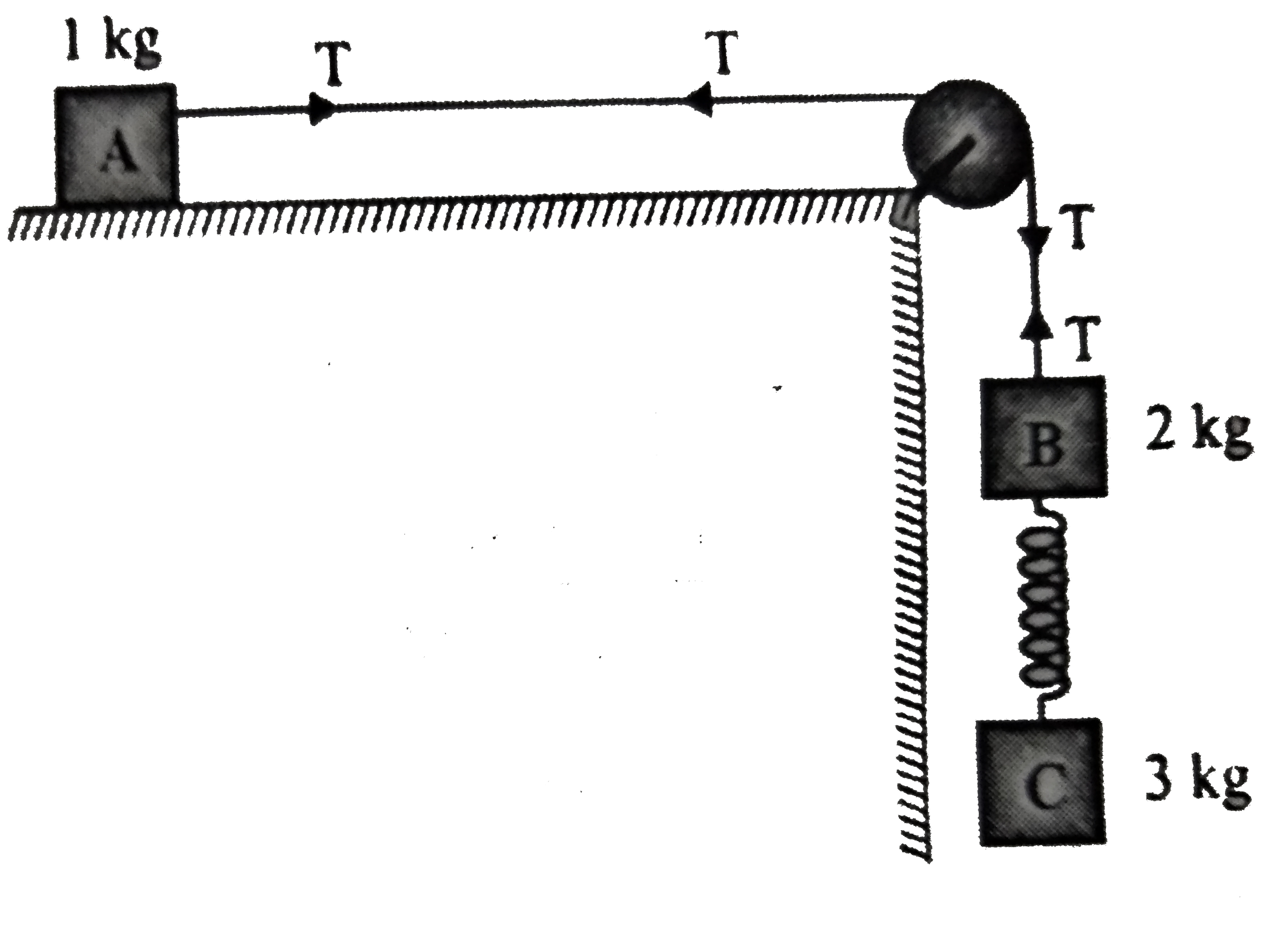
(a) acceleration of the system
(b) tensionin the string and
(c ) ectension in the spring if force constant of spring is k = 50 N/m (Take g = 10 m/s2)
View Solution
Knowledge Check
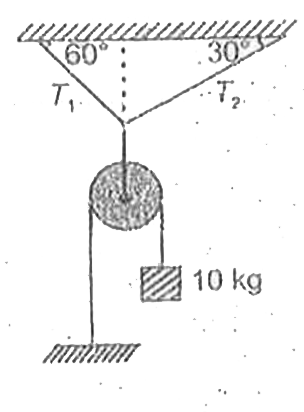
In the arrangement as shown, tension T2 is -
(g=10m/s2)
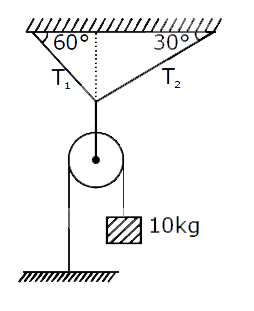 A50 NB100 NC50√3ND100√3N
A50 NB100 NC50√3ND100√3NIn the system shadow in the adjoining figure, the tension T2 is
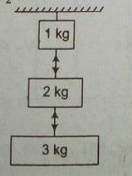 AgB2gC5gD6g
AgB2gC5gD6gIn the system shadow in the adjoining figure, the tension T2 is
 AgB2gC5gD6g
AgB2gC5gD6g
In the figure shown, find acceleration of the system and tenslons T1,T2andT3(Take=10m/s2)
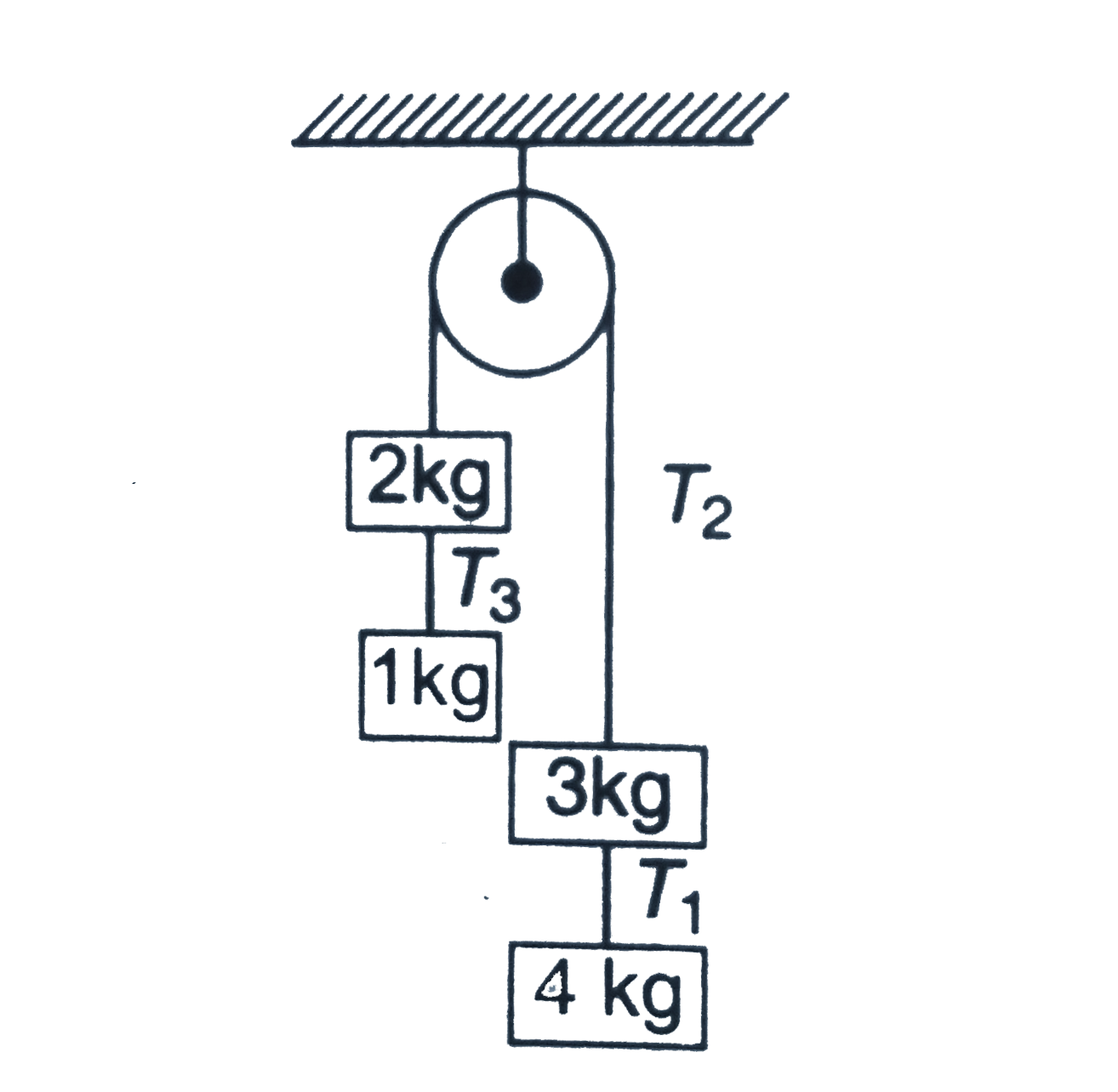
View SolutionFor the system shown in figure, the tensions in the string
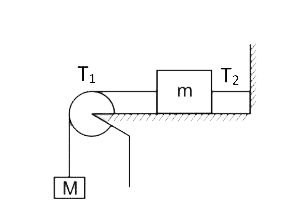
View SolutionIn the arrangement as shown, tension T2 is (g=10m/s2)
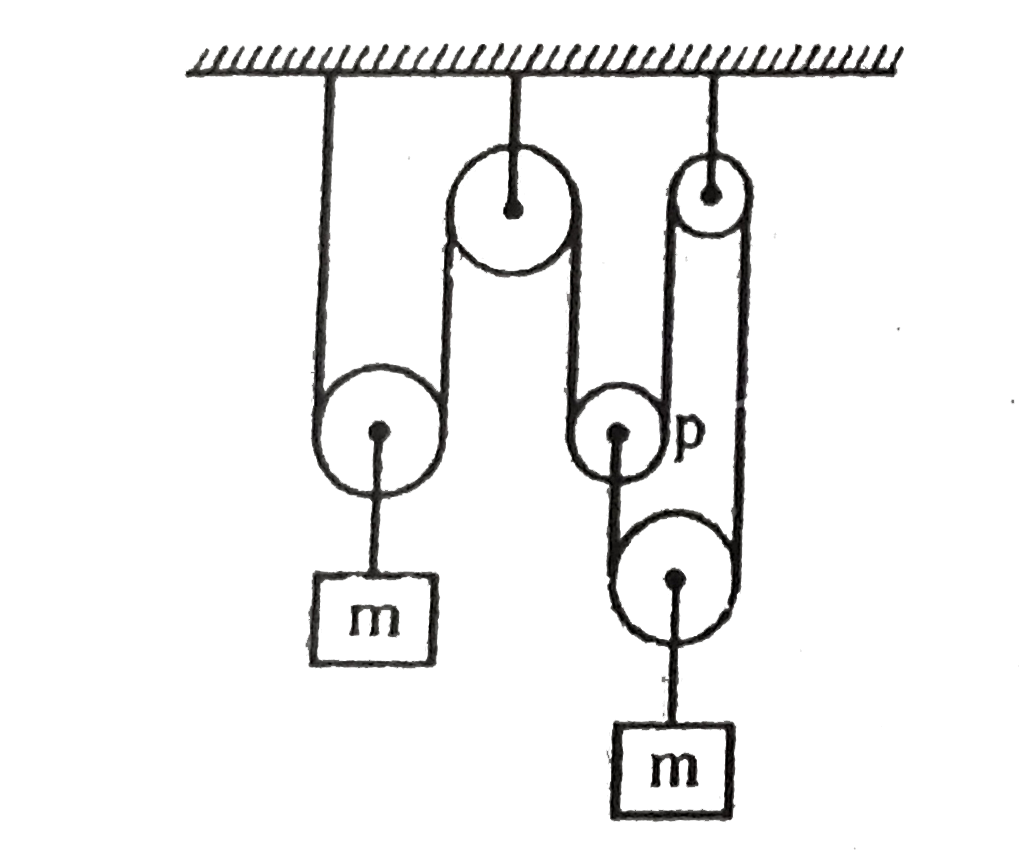
View SolutionAll pulleys are massless The string is light and inextensible Find acceleration of centre of pulley 'p'∈m/s2
View SolutionIn the system shown in the given figure, the inline is frictionless, the string is massless, and inextensible pulley is light and frictionless. The system is released from rest.

View Solution